A. Resolved Color Modelling
Consider the central equation of population synthesis [6], commonly used to model spectral evolution in galaxies:
In this formulation the emergent flux from a galaxy, F
, at
time T is described by the convolution of the spectrum of an
evolving instantaneous starburst, f(t), with an assumed
star-formation rate (SFR) function 
(t). The f(t) term
is in principle known for various choices of initial mass function
(IMF) and metallicity, using libraries of template stellar spectra and
isochrones. Hierarchical formation scenarios suggest that stellar
populations become built up over time, so in reality the age T of a
galaxy is not a constant, and the distribution of internal colors is a
partial record of the formation timescale(s) of the system. This age
information for a given stellar population is diluted by the
convolution with (t), whose
form is usually unknown. But in
regions where the resolution is high enough to resolve the sites of
current star-formation, or where mixing of multiple generations of
stars has not occured, (t)
can be approximated by a
-function, breaking the
convolution degeneracy in Equation 1,
and allowing direct measurement the age distribution and form of
f(t). Consider, for example, the canonical
picture of a late-type spiral galaxy, where the bulk
(t) can be
well-approximated by a constant star-formation rate. This
"constant" overall star formation rate is physically simply a
time-average over the appearance and disappearance of spatially
distinct HII regions and star-formation complexes, each of which
individually can be considered to be a bursting simple stellar
population with a lifetime (before disruption or gas depletion) that
is short compared to the dynamical timescale of the galaxy. Therefore
the distribution of colors for individual resolved young stellar
associations on a color-color diagram directly maps out the shape of
f(t)
for a set of young ages, giving direct access to the
integrand of Equation~1 without first filtering by a convolution. As
these stellar associations age and disappear the convolution with
(t) in Equation 1 becomes
important, as young stars become
assimilated into older galactic components (ie. the disk and bulge)
and are spatially averaged with earlier generations of stars. The
distribution of colors for older stellar populations (
1 Gyr)
are therefore expected to trace out a continuous age track on the
color-color diagram. The shape of this track for older stellar
populations is in effect a measurement of the form of the
star-formation law (t),
while the distribution of colors
along this track is a record of the uniformity with which episodes of
star formation have added to the stellar population (e.g., via numerous
small bursts, or a smaller number of larger bursts).
|
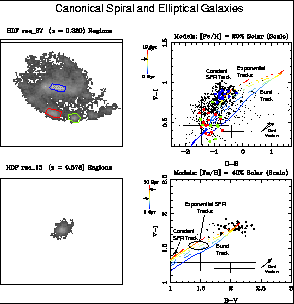
Figure 4. Morphophotometric color-color
diagrams for two "canonical" galaxies in the Hubble Deep Field. The
panels on the left show I-band images of the galaxies, "segmented"
from the background sky by isophotal thresholding. The right
hand panels show the color-color diagrams for individual
pixels in the galaxy. The data points have been subdivided
into high signal-to-noise (SNR) pixels [circles] and low
signal-to-noise pixels [dots]. The mean error bars for the
high SNR and low SNR points are shown by the dark and light
error bars. Model tracks are also shown on the right, and are
calibrated to age in Gyr [keyed to the color bar]. Arrows on
the color bar indicate the age of the Universe in the rest
frame of the galaxy, for H0 = 70 km/s/Mpc and
|
Figure 4 shows the resolved color-color diagram for
two rather typical spiral and elliptical galaxies in the HDF. These
exhibit star-formation characteristics that are in reasonable
agreement with our expectations based on studies of stellar
populations in local galaxies. For example, the spiral galaxy is well
described by a roughly constant star formation history. The bulge is
the oldest component of this system, has a small dispersion in color,
and is several Gyr older than the disk. By contrast the elliptical
system shown is well described by an exponential star-formation
history with a short e-folding timescale (around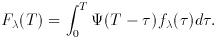 
= 1 Gyr.) This
system is apparently rather old and (from the small dispersion in
color), all parts of the galaxy are well mixed and roughly coeval. The
majority of spirals and ellipticals in the Hubble Deep Field exhibit
morphophotometric diagrams qualitatively similar to those shown in
Figure 4.
= 0.1
and