

The stars in globular clusters are believed to be coeval and, with few exceptions, globular clusters are remarkably homogeneous in chemical composition. For these reasons globular clusters are ideal objects to test stellar evolution theory, and for age determinations (for historical background, the reader is referred to Demarque et al. 1991).
Because globular clusters are the oldest objects in the Galaxy which can
be dated
readily, the ages of the oldest among them puts a strong constraint on
the age of the
Universe. The purpose of this Symposium is to discuss the state of our
knowledge of the
distance scale and the determination of the Hubble parameter
H0. H0 sets an expansion
age for the Universe which depends on the specific cosmological
model. The nuclear age of
the oldest stars thus provides an independent constraint on the age of
the Universe, which
must be consistent with the expansion age within a given cosmological
model. There is
currently much interest in what cosmological model best represents the
Universe. In
standard relativistic cosmology, one can relate in a simple way the age
of the Universe
to the Hubble constant H0 and the mean density of the
Universe  (in units of the
critical density), as shown in Figure 1, where
for simplicity, the cosmological constant
(in units of the
critical density), as shown in Figure 1, where
for simplicity, the cosmological constant
 was set equal to zero. From
Figure 1, we see
for example that for H0 = 60 km s-1
Mpc-1, and if the oldest stars are 14 Gyr old,
was set equal to zero. From
Figure 1, we see
for example that for H0 = 60 km s-1
Mpc-1, and if the oldest stars are 14 Gyr old,
 cannot be larger than 0.2
(indeed must
be unrealistically near zero, since the oldest stars must have formed
some time after the
Big Bang). In this case, standard relativistic cosmology would require
the introduction of a non-zero cosmological constant.
cannot be larger than 0.2
(indeed must
be unrealistically near zero, since the oldest stars must have formed
some time after the
Big Bang). In this case, standard relativistic cosmology would require
the introduction of a non-zero cosmological constant.
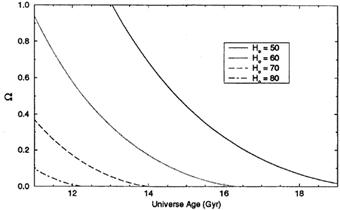
|
Figure 1. The density of the Universe
|
If we are to judge by past experience, however, it would not be surprising if some key pieces were still missing in the cosmological puzzle that we are trying to assemble; these pieces may well transform completely our view of the Universe. But even in the event that we have to abandon most of our present views on cosmology, the ages of the oldest star clusters will remain a basic datum for any cosmological model of the future. Even in a very unconventional cosmology, such as the revised Steady State cosmology recently described by Narlikar et al. (1995) in which the Universe has no beginning, globular cluster ages are significant timeposts, which help set the scale of universal evolution.
Rapid change is now taking place in our understanding of the structure and internal dynamics of stars. Dramatic improvements in our knowledge of opacities and the equation of state of stellar matter have occurred. And these improvements can now be tested with much greater precision due to the combined improvements of CCD detectors and the HST data. At the same time, seismology for the first time enables us to probe directly the structure and dynamics of the inner layers of the Sun (Christensen-Dalsgaard et al. 1996; Guenther et al. 1996), and this work is being extended to stars. Most of the results described in this paper could not have been discussed five years ago.
Another relevant advance in the last few years has been the ability to determine relative ages of globular clusters with much greater reliability than what was previously possible. And this, coupled with progress on their kinematics has led to the realization that the globular cluster system is composite, and that there is a range of ages among globular clusters, as illustrated in Figure 2 (Sarajedini and King 1989; Lee et al. 1990, 1994; Chaboyer et al. 1996a). Understanding these results in terms of the formation and evolution of the galactic halo and disk have become a very active field of research. This challenge to stellar evolution theory has been an opportunity to refine theoretical age calibrations.

|
Figure 2. Age as a function of metallicity for 43 globular clusters, assuming Mv(RR) = 0.20[Fe/H]+0.98. |
Thus understanding stellar evolution is important to cosmology in more than providing one single number, a lower bound to the age of the Universe. Stellar evolution provides a varied array of interfaces with observation and laboratory physics. It helps us understand the formation and evolution of our Galaxy and nearby systems, and the spectral energy distributions of distant galaxies, and their evolution in time (Yi et al. 1995). It provides an interpretation of the light elements abundances relevant to big bang nucleosynthesis (Deliyannis et al. 1995). And the same physical principles that explain the behavior of the Sun and sun-like stars, also apply to Cepheid variables, and to novae and supernovae, which are used as standard candles in cosmology.