


3.2.2. Evolution of the Rich Cluster Abundance
The cluster abundance at z
 0 requires
the rms mass fluctuation
0 requires
the rms mass fluctuation
 8 =
<(
8 =
<( M/M)>1/2|r
= 8h-1 Mpc to
satisfy
(White et al. 1993a;
Eke et al. 1996;
Pen 1998;
Viana & Liddle 1999;
see also
Henry & Arnaud 1991)
M/M)>1/2|r
= 8h-1 Mpc to
satisfy
(White et al. 1993a;
Eke et al. 1996;
Pen 1998;
Viana & Liddle 1999;
see also
Henry & Arnaud 1991)
The evolution of the cluster abundence is sensitive to
The controversy among authors
arises from different estimates of the cluster mass at
high z. This is a subtle effect, since the mass varies little
over the range of relevant redshift, while the cluster number density
evolution is sufficiently rapid at fixed mass
(Pen 1998).
At low z we have an established mass temperature relation,
and the cluster mass is securely estimated
(Henry & Arnaud
1991).
At high z, however, such direct
information is not available. Blanchard & Bartlett and Eke et al.
used mass temperature relations as a function of z
derived from hydrodynamic simulations. Reichart et al. used an
extrapolated mass X-ray luminosity relation. Bahcall and Fan
used more direct estimates of the cluster mass at higher z
for three clusters. A change of a factor of two in the mass estimate
would modify the conclusion.
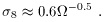
 8 in early
epochs of growth for a given mass; it is z
8 in early
epochs of growth for a given mass; it is z
 0.3 for rich clusters.
The rich cluster abundance at z ~ 0.3-1, when compared with
that at a low z,
determines both
0.3 for rich clusters.
The rich cluster abundance at z ~ 0.3-1, when compared with
that at a low z,
determines both  8
and
8
and  (Oukbir &
Blanchard 1992).
Carlberg et
al. (1997b)
derived
(Oukbir &
Blanchard 1992).
Carlberg et
al. (1997b)
derived  = 0.4 ± 0.2, and
Bahcall & Fan (1998)
obtained
= 0.4 ± 0.2, and
Bahcall & Fan (1998)
obtained  =
0.2+0.3-0.1 corresponding to a slow growth of the
abundance. On the other hand,
Blanchard &
Bartlett (1998)
obtained
=
0.2+0.3-0.1 corresponding to a slow growth of the
abundance. On the other hand,
Blanchard &
Bartlett (1998)
obtained 
 1 from a more rapid growth.
A high value is also claimed by
Reichart et
al. (1999), while
Eke et al. (1998)
reported
1 from a more rapid growth.
A high value is also claimed by
Reichart et
al. (1999), while
Eke et al. (1998)
reported  = 0.43 ± 0.25
for an open,
and
= 0.43 ± 0.25
for an open,
and  = 0.36 ± 0.25 for a
flat universe.
= 0.36 ± 0.25 for a
flat universe.