
Photometric Redshifts |
The concept of photometric redshifts is not new. This essay provides a brief history of photometric redshifts. The list of papers provided below is not exhaustive; however, it does cover the development of the technique up to about 1996. - S.D.J. Gwyn
Baum (1962) was the first to develop a technique for measuring redshifts photometrically. He used a photoelectric photometer and 9 bandpasses spanning the spectrum from 3730Å to 9875Å. With this system he observed the spectral energy distribution (SED) of 6 bright elliptical galaxies in the Virgo cluster. He then observed 3 elliptical galaxies in another cluster (Cl 0925+2044, also known as Abell 0801). By plotting the average SED of the Virgo galaxies and the average SED of the Cl0925 galaxies on the same graph using a logarithmic wavelength scale, he was able to measure the displacement between the two energy distributions, and hence the redshift of the second cluster. His redshift value of z = 0.19 agreed closely with the known spectroscopic value of z = 0.192, so he extended his technique to a handful of clusters of then unknown redshifts out to maximum redshift of z = 0.46. He then derived a very rough value of Omega_0. Baum's technique was fairly accurate, but because of its dependence on a large 4000Å break spectral feature, it could only work on elliptical galaxies.
Koo (1985) followed a different approach. First, he used photographic plates instead of a photometer, making it possible to measure photometric redshifts for a large number of galaxies simultaneously. Second, instead of using 9 filters he used only 4: UJFN (photographic U, BJ, RF and IN). Third, instead of using an empirical spectral energy distribution, he used the theoretical Bruzual (1983, among others) no-evolution models for all galaxy types.
The most important difference, however, was the way the colours were used. Instead of converting the photometric colours into a kind of low resolution spectrum, he converted the Bruzual templates into colours, and plotted lines of constant redshift and varying spectral type, known as iso-z lines, on a colour-colour diagram. Finding that the most normal colour-colour diagrams (e.g. U-J versus J-F and J-F versus F-N) were degenerate in a range of redshifts, he invented what he called colour-shape diagrams. The shape measured whether the SED turned up or down at both ends, that is, whether the spectrum was bowl shaped or humped. Another way to put it is that the colour measured the first derivative with respect to wavelength of the spectrum and the shape measured the second derivative. For colour he used either 2U-2F or U+J-F-N , both of which span a large wavelength range. For shape, he used either U+2J-F or -U+J+F-N . Following this method to measure the redshift of a galaxy, Koo calculated the colour and the shape from the UJFN magnitudes and plotted them on the colour-shape diagram. The redshift of the galaxy was then found by finding the iso-z line closest to the point representing the galaxy. Koo tested this method on a sample of 100 galaxies with known spectroscopic redshifts ranging from z = 0.025 to z = 0.700.
This method is similar to that used by Pello et al. (1996) and Miralles, Pello & Le Borgne (1996). They used the colours of galaxies to determine ``permitted redshifts'' in the following manner: The colors of galaxies are plotted as a function of redshift from the Bruzual & Charlot (1993) models. Each available color (with its associated uncertainty) of a galaxy defines a ``permitted'' redshift range on the corresponding color-redshift diagram. The intersection of the permitted redshift ranges for all the colours determines the redshift. This method was used by Pello et al. (1996) to discover a cluster of galaxies at z > 0.75 by looking for an excess in the redshift distribution in the field of a gravitationally lensed quasar. Miralles et al. (1996) used the method to determine the redshift distribtion of the Hubble Deep Field.
The ``ultra-violet dropout'' techniques of Steidel et al. (1996) and Madau et al. (1996) are similar if simpler. All galaxy spectra have a large Lyman break; shortward of 912Å, the continuum drops dramatically. When this break is redshifted into and past the U filter, the U flux is greatly reduced or non-existant, resulting in very red ultra-violet colours.
In the ultra-violet dropout techniques, an exact redshift of a galaxy is not determined. Rather, the redshift is determined to be in the redshift range where the Lyman break is in or just past the U filter. Since U filters typically have a central wavelength of 3000Å, this works out to a redshift of z > 2.25. In practical terms, redshifted template galaxy spectra are used to determine a locus on a colour-colour plot where most galaxies lie in a particular redshift range. Those galaxies whose measured colours lie within the locus are deemed to be in that redshift range. Clearly, this method is a lot simpler than that of Pello et al. (1996) as only two colours are considered. It is also a lot less precise as the redshift is not very constrained. For both these reasons it is ideally suited for pre-selecting galaxies at high redshift for spectroscopic confirmation. Steidel et al. (1996) did exactly this using the UGR filters. Madau et al. (1996) applied this technique to the Hubble Deep Field using the F300W, F450W, F606W and F814W filters. The technique was extended by using F450W dropouts to find galaxies of redshifts z = 4.
The template fitting technique developped by
Loh & Spillar (1986b) more
closely resembles that of Baum (1962)
than that of Koo (1985).
Loh & Spillar (1986b)
observed 34 galaxies of known redshift in the
galaxy cluster 0023+1654 through 6 non-standard filters to test their
method. The standard deviation of the redshift differences
(zspec - zphot) was 0.12. They went
on to use their technique to
measure photometric redshifts for 1000 field galaxies in order to
determine a value for the density parameter,  0
(Loh & Spillar 1986a).
0
(Loh & Spillar 1986a).
Gwyn (1995)
tested this method using BVRI photometry of the Colless et
al. (Colless et al., 1990;
Colless et al., 1993) galaxies. The
larger uncertainty in photmetric redshifts thus derived
( z
= 0.18) was attributed to the lack of a U filter. Numerous authors
(Gwyn and Hartwick 1996;
Lanzetta et al., 1996;
Mobasher et al., 1996;
Sawicki et al., 1996;
Cowie et al., 1996) have used this
technique to determine redshifts in the Hubble Deep Field.
z
= 0.18) was attributed to the lack of a U filter. Numerous authors
(Gwyn and Hartwick 1996;
Lanzetta et al., 1996;
Mobasher et al., 1996;
Sawicki et al., 1996;
Cowie et al., 1996) have used this
technique to determine redshifts in the Hubble Deep Field.
Prehaps the simplest and certainly the most empirical photometric redshift technique yet is that of Connolly et al. (1995a). This method requires a ``training set'' of a large number of galaxies with multi-color photometry and spectroscopic redshifts. Redshift, z, is assumed to be a linear or quadratic function of the magnitudes (Mi) of the galaxies, i.e. if N is the number of filters:
The constants, ai and aij, are found by
linear regression.
Connolly et al. (1995a)
used a UJFN plus redshift
data set extending to z = 0.5 of 370 galaxies. They showed that this
method could determine redshifts with uncertainties of
The advantage of the linear regression technique is its extreme
simplicity. It has a few disadvantages: (1) A substantial collection
of spectroscopic redshifts must have been measured before the
technique can used. (2) Extension to fainter magnitudes or deeper
redshifts is not possible.
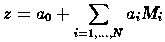
or
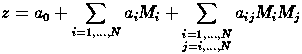
 z = 0.057
with a linear fit and
z = 0.057
with a linear fit and  z = 0.047 with a quadratic fit. There is
little or no loss of accuracy if colors (Ci = Mi -
Mi+1) are used
instead of magnitudes. Using this technique they were able to measure
the luminosity function out to J = 24 (SubbaRao et al., 1996).
z = 0.047 with a quadratic fit. There is
little or no loss of accuracy if colors (Ci = Mi -
Mi+1) are used
instead of magnitudes. Using this technique they were able to measure
the luminosity function out to J = 24 (SubbaRao et al., 1996).
References
Baum, W. A.: 1962, in G. C. McVittie (ed.), Problems of
extra-galactic research, p. 390, IAU Symposium No. 15
Bruzual, G. A.: 1983, Astrophys. J. 273, 105
Bruzual, G. A. and Charlot, S.: 1993, Astrophys. J. 405, 538
Colless, M. M., Ellis, R. S., Broadhurst, T. J., and Peterson, B.
A.: 1993, M.N.R.A.S. 244, 408
Colless, M. M., Ellis, R. S., Taylor, K., and Hook, R. N.: 1990,
M.N.R.A.S. 244, 408
Connolly, A. J., Csabai, I., Szalay, A. S., Koo, D. C., Kron, R. G.,
and Munn, J. A.: 1995a, Astron. J. 110, 2655
Cowie, L. L., Clowe, D., Fulton, E., Cohen, J. G., Hu, E. M., ,
Songaila, A., Hogg, D. W., and Hodapp, K. W.: 1996, Redshifts,
colors and morphologies of the K selected galaxy sample in the
Hubble Deep Field, in preparation
Gwyn, S. D. J.: 1995, Master's thesis, University of Victoria
Gwyn, S. D. J. and Hartwick, F. D. A.: 1996, Astrophys. J. Let. 468, L77
Koo, D. C.: 1985, Astron. J. 90, 418
Lanzetta, K. M., Yahil, A., and Fernandez-Soto, A.: 1996,
Star-forming galaxies at very high redshifts, Preprint [astro-ph/9606171]
Loh, E. D. and Spillar, E. J.: 1986a, Astrophys. J. 307, L1
Loh, E. D. and Spillar, E. J.: 1986b, Astrophys. J. 303, 154
Madau, P., Ferguson, H. C., Dickinson, M. E., Giavalisco, M., Steidel,
C. C., and Fruchter, A. S.: 1996, High redshift galaxies in the
Hubble Deep Field. Color selection and star formation history to
z = 4, Preprint [astro-ph/9607172]
Miralles, J.-M., Pello, R., and Le Borgne, J.-F.: 1996, Photometric
Analysis of the Hubble Deep Field, Preprint
Mobasher, B., Rowan-Robinson, M., Georgakakis, A., and Eaton, N.:
1996, The nature of the faint galaxies in the Hubble Deep Field,
Preprint [astro ph/9604118]
Pello, R., Miralles, J.-M., Picat, J.-P., Soucail, G., and Bruzual,
G. A.: 1996, Identification of a high redshift cluster in the
field of Q2345+007 through deep BRIJK photometry, Preprint [astro-ph/9603146]
Sawicki, M. J., Lin, H., and Yee, H. K. C.: 1996, Evolution of the
Galaxy Population Based on Photometric Redshifts in the Hubble
Deep Field, Preprint
Steidel, C. C., Giavalisco, M., Pettini, M., Dickinson, M. E., and
Adelberger, K. L.: 1996, Spectroscopy of Lyman break galaxies in
the Hubble Deep Field, Preprint [astro-ph/9604140]
SubbaRao, M. U., Connolly, A. J., Szalay, A. S., and Koo, D. C.:
1996, Luminosity Functions from Photometric Redshifts I:
Techniques, Preprint [astro-ph/9606075]
Send comments/suggestions/problems to Stephen D.J. Gwyn gwyn@uvastro.phys.uvic.ca