


With the help of some simple stellar population synthesis tools we can now
set a lower limit to the total stellar mass density
that produced the observed EBL, and constrain
the cosmic history of star birth in galaxies. One of the most serious
uncertainties in this calculation is the lower cutoff, usually treated as a
free parameter, of the initial mass function (IMF).
Observations of M subdwarfs stars with the HST have recently
shed some light on this issue, showing that the IMF in the Galactic disk can
be represented analytically
over the mass range 0.1 < m < 1.6 (here m is in solar
units) by log
 (m) = const -2.33 log
m - 1.82(log m)2
(Gould et al. 1996,
hereafter GBF;
Gould et al. 1997).
For m > 1 this
mass distribution agrees well with a Salpeter function, log
(m) = const -2.33 log
m - 1.82(log m)2
(Gould et al. 1996,
hereafter GBF;
Gould et al. 1997).
For m > 1 this
mass distribution agrees well with a Salpeter function, log
 (m) = const - 2.35 log
m. A shallow mass function (relative to the Salpeter slope)
below 1 M
(m) = const - 2.35 log
m. A shallow mass function (relative to the Salpeter slope)
below 1 M has
been measured in the Galactic bulge as well
(Zoccali et al. 1999).
Observations of normal Galactic star-forming regions also show
some convergence in the basic form of the IMF at intermediate and high masses,
a power-law slope that is consistent with the Salpeter value (see
Elmegreen 1998;
Massey 1998,
and references therein).
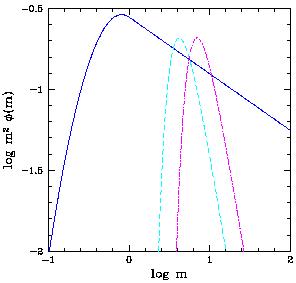
In the following we will use a ``universal'' IMF (shown in
Figure 3) with the
GBF
form for m < 1, matched to a Salpeter slope for m
has
been measured in the Galactic bulge as well
(Zoccali et al. 1999).
Observations of normal Galactic star-forming regions also show
some convergence in the basic form of the IMF at intermediate and high masses,
a power-law slope that is consistent with the Salpeter value (see
Elmegreen 1998;
Massey 1998,
and references therein).
In the following we will use a ``universal'' IMF (shown in
Figure 3) with the
GBF
form for m < 1, matched to a Salpeter slope for m
 1; the mass integral of this
function is 0.6 times that obtained extrapolating a Salpeter function down to
0.1 M
1; the mass integral of this
function is 0.6 times that obtained extrapolating a Salpeter function down to
0.1 M . (2)
. (2)
|
Figure 3. Stellar initial mass functions, |
As shown in Figure 4, the bolometric
luminosity as a function of age  of
a simple stellar population (a single generation of coeval,
chemically homogeneous stars having total mass M, solar
metallicity, and the above IMF) can be well approximated by
of
a simple stellar population (a single generation of coeval,
chemically homogeneous stars having total mass M, solar
metallicity, and the above IMF) can be well approximated by
(cf Buzzoni 1995).
Over a timescale of 13 Gyr (the age of the universe for an EdS cosmology with
h = 0.5), about 1.3 MeV per stellar baryon will be radiated
away. This number
depends only weakly on the assumed metallicity of stars.
In a stellar system with arbitrary star formation rate per
comoving cosmological volume,
Therefore the total background light observed at Earth (t =
tH),
generated by a stellar population with a formation epoch
tF, is
where the factor (1 + z) at the denominator is lost to cosmic expansion
when converting from observed to radiated (comoving) luminosity density.
Figure 4. Left: Synthetic
(based on an update of
Bruzual & Charlot's 1993
libraries) bolometric luminosity versus age of a simple stellar
population having total mass M = 1 M
To set a lower limit to the present-day mass density,
A visible mass density at the level of the above lower limit, while able to
explain the measured sky brightness, requires (most of the stars) that
give origin to the observed light to have formed at very low redshifts
(zF
The observed EBL therefore implies a ``fiducial'' mean star
formation density of <
Perhaps a more realistic scenario is one where the star formation
density evolves as
This fits reasonably well all
measurements of the UV-continuum and H
We conclude that, depending on the star formation history and for the assumed
IMF, the observed EBL requires between 7% and 16% of the nucleosynthetic
baryon density to be today in the forms of stars, processed gas, and their
remnants.
According to the most recent census of cosmic baryons, the mass density in
stars and their remnants observed today is
3 An asymptotic mass fraction
of stars returned as gas, R =
4 Here we have taken yZ
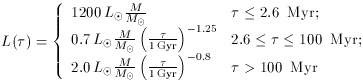
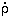 s, the bolometric emissivity at time t is
given by the convolution integral
s, the bolometric emissivity at time t is
given by the convolution integral
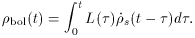
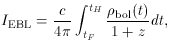
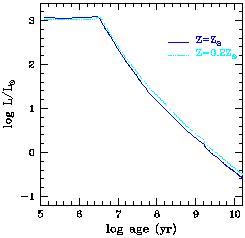

 , metallicity
Z = Z
, metallicity
Z = Z (solid line) and Z = 0.2 Z
(solid line) and Z = 0.2 Z (dotted line), and
a GBF+Salpeter IMF (see text for details).
Right: EBL observed at Earth from the instantaneous formation at
redshift zF of a stellar population having the same
IMF (Z = Z
(dotted line), and
a GBF+Salpeter IMF (see text for details).
Right: EBL observed at Earth from the instantaneous formation at
redshift zF of a stellar population having the same
IMF (Z = Z )
and mass density
)
and mass density  g+s h2 = 0.0018, 0.0013, and 0.0008,
as a function of
zF. Solid curves: EdS universe with h =
0.5 (tH = 13 Gyr).
Dashed curves:
g+s h2 = 0.0018, 0.0013, and 0.0008,
as a function of
zF. Solid curves: EdS universe with h =
0.5 (tH = 13 Gyr).
Dashed curves:
 -dominated universe with
-dominated universe with
 M = 0.3,
M = 0.3,

 = 0.7, and
h = 0.65 (tH = 14.5 Gyr).
= 0.7, and
h = 0.65 (tH = 14.5 Gyr).
 g+s, of
processed gas + stars
(in units of the critical density
g+s, of
processed gas + stars
(in units of the critical density  crit = 2.77
x 1011 h2 M
crit = 2.77
x 1011 h2 M Mpc-3), consider now
a scenario where all stars are formed instantaneously at redshift
zF.
The background light that would be observed at Earth from such an event
is shown in Figure 4 as a function of
zF for
Mpc-3), consider now
a scenario where all stars are formed instantaneously at redshift
zF.
The background light that would be observed at Earth from such an event
is shown in Figure 4 as a function of
zF for
 g+s
h2 = 0.0008,
0.0013, 0.0018, corresponding to 4, 7, and 9 percent of the
nucleosynthetic baryon density,
g+s
h2 = 0.0008,
0.0013, 0.0018, corresponding to 4, 7, and 9 percent of the
nucleosynthetic baryon density,  b h2 = 0.0193 ± 0.0014
(Burles & Tytler 1998).
Two main results are
worth stressing here: (1) the time evolution of the luminosity radiated by a
simple stellar population (eq. 4) makes the dependence of the
observed EBL from zF much shallower than the (1 +
zF)-1
lost to cosmic expansion (eq. 6), as the energy output from
stars is spread over their respective lifetimes; and (2) in order to generate
an EBL at a level of 50 I50 n W m-2
sr-1, one requires
b h2 = 0.0193 ± 0.0014
(Burles & Tytler 1998).
Two main results are
worth stressing here: (1) the time evolution of the luminosity radiated by a
simple stellar population (eq. 4) makes the dependence of the
observed EBL from zF much shallower than the (1 +
zF)-1
lost to cosmic expansion (eq. 6), as the energy output from
stars is spread over their respective lifetimes; and (2) in order to generate
an EBL at a level of 50 I50 n W m-2
sr-1, one requires
 g+s
h2 > 0.0013 I50 for an EdS universe
with h = 0.5, hence a mean
mass-to-blue light ratio today of < M / LB
>g+s > 3.5 I50
(the total blue luminosity density at the present-epoch is
g+s
h2 > 0.0013 I50 for an EdS universe
with h = 0.5, hence a mean
mass-to-blue light ratio today of < M / LB
>g+s > 3.5 I50
(the total blue luminosity density at the present-epoch is
 B =
2 x 108 h L
B =
2 x 108 h L Mpc-3,
Ellis et al. 1996).
The dependence of these estimates on the
cosmological model (through eq. 6) is rather weak.
With the adopted IMF, about 30% of this mass will be returned to
the interstellar medium in 108 yr, after intermediate-mass
stars eject
their envelopes and massive stars explode as supernovae. This return fraction,
R, becomes 50% after about 10 Gyr.
(3)
Mpc-3,
Ellis et al. 1996).
The dependence of these estimates on the
cosmological model (through eq. 6) is rather weak.
With the adopted IMF, about 30% of this mass will be returned to
the interstellar medium in 108 yr, after intermediate-mass
stars eject
their envelopes and massive stars explode as supernovae. This return fraction,
R, becomes 50% after about 10 Gyr.
(3)
 0.5), a scenario which appears to be ruled out by the observed
evolution of the UV luminosity density
(Madau 1999).
For illustrative purposes, it is interesting to consider instead a model where
the star formation rate per unit comoving volume stays approximately constant
with cosmic time. In an EdS cosmology with h = 0.5, one derives
from equations (4), (5), and (6)
0.5), a scenario which appears to be ruled out by the observed
evolution of the UV luminosity density
(Madau 1999).
For illustrative purposes, it is interesting to consider instead a model where
the star formation rate per unit comoving volume stays approximately constant
with cosmic time. In an EdS cosmology with h = 0.5, one derives
from equations (4), (5), and (6)
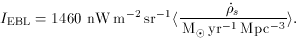
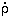 s > = 0.034
I50 M
s > = 0.034
I50 M yr-1 Mpc-3
(or a factor of 1.6 higher in the case of a Salpeter IMF down to 0.1
M
yr-1 Mpc-3
(or a factor of 1.6 higher in the case of a Salpeter IMF down to 0.1
M ). Any value
much greater than this over a sizeable
fraction of the Hubble time will generate an EBL intensity well in excess
of 50 n W m-2 sr-1.
Ignoring for the moment the recycling of returned gas into new stars,
the visible mass density at the present epoch is simply
). Any value
much greater than this over a sizeable
fraction of the Hubble time will generate an EBL intensity well in excess
of 50 n W m-2 sr-1.
Ignoring for the moment the recycling of returned gas into new stars,
the visible mass density at the present epoch is simply
 g+s =
g+s =
 0tH
0tH 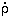 s (t)dt = 4.4 x
108 I50 M
s (t)dt = 4.4 x
108 I50 M Mpc-3,
corresponding to
Mpc-3,
corresponding to  g+s
h2 = 0.0016 I50
(< M / LB >g+s = 4.4 I50).
g+s
h2 = 0.0016 I50
(< M / LB >g+s = 4.4 I50).
 luminosity densities
from the present-epoch to z = 4 after an extinction correction of
A1500 = 1.2 mag (A2800 = 0.55 mag)
is applied to the data
(Madau 1999),
and produce a total EBL of about the right magnitude
(I50
luminosity densities
from the present-epoch to z = 4 after an extinction correction of
A1500 = 1.2 mag (A2800 = 0.55 mag)
is applied to the data
(Madau 1999),
and produce a total EBL of about the right magnitude
(I50  1).
Since about half of the present-day stars are formed at z > 1.3
in this model and their contribution to the EBL is redshifted away,
the resulting visible mass density is
1).
Since about half of the present-day stars are formed at z > 1.3
in this model and their contribution to the EBL is redshifted away,
the resulting visible mass density is  g+s h2 = 0.0031 I50
(< M / LB >g+s = 8.6
I50), almost twice as large as in the
g+s h2 = 0.0031 I50
(< M / LB >g+s = 8.6
I50), almost twice as large as in the
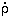 s = const approximation.
s = const approximation.
 s h =
0.00245+0.00125-0.00088
(FHP),
corresponding to a
mean stellar mass-to-blue light
ratio of < M / LB >s =
3.4+1.7-1.3 for h = 0.5 (roughly 70%
of this mass is found in old spheroidal populations). While this is about a
factor of 2.5 smaller than the visible mass density predicted by equation
(8), efficient recycling of ejected material into new star
formation would tend to reduce the apparent discrepancy in the budget.
Alternatively, the gas returned by stars may be ejected into the intergalactic
medium. With an IMF-averaged yield of returned metals of
yZ
s h =
0.00245+0.00125-0.00088
(FHP),
corresponding to a
mean stellar mass-to-blue light
ratio of < M / LB >s =
3.4+1.7-1.3 for h = 0.5 (roughly 70%
of this mass is found in old spheroidal populations). While this is about a
factor of 2.5 smaller than the visible mass density predicted by equation
(8), efficient recycling of ejected material into new star
formation would tend to reduce the apparent discrepancy in the budget.
Alternatively, the gas returned by stars may be ejected into the intergalactic
medium. With an IMF-averaged yield of returned metals of
yZ  1.5 Z
1.5 Z , (4)
the predicted mean metallicity at the present epoch is
yZ
, (4)
the predicted mean metallicity at the present epoch is
yZ  g+s /
g+s /  b
b  0.25 Z
0.25 Z , in good agreement with the values
inferred from cluster abundances
(Renzini 1997).
, in good agreement with the values
inferred from cluster abundances
(Renzini 1997).
2 The bolometric light contributed by
stars less massive than 1
M is very small
for a ``typical'' IMF. The use of the
GBF
mass function at low masses instead of Salpeter then leaves the total
radiated luminosity of a stellar population virtually unaffected.
Back.
is very small
for a ``typical'' IMF. The use of the
GBF
mass function at low masses instead of Salpeter then leaves the total
radiated luminosity of a stellar population virtually unaffected.
Back.
 (m - mf)
(m - mf)  (m)dm x
[
(m)dm x
[ m
m (m)dm]-1
(m)dm]-1
 0.5, can be obtained by
using the semiempirical
initial (m)-final (mf) mass relation of
Weidemann (1987)
for stars with 1 < m < 10, and by assuming
that stars with m > 10 return all but a 1.4 M
0.5, can be obtained by
using the semiempirical
initial (m)-final (mf) mass relation of
Weidemann (1987)
for stars with 1 < m < 10, and by assuming
that stars with m > 10 return all but a 1.4 M remnant.
Back
remnant.
Back

 mpzm
mpzm  (m)dm
x [
(m)dm
x [ m
m (m)dm]-1, the stellar
yields pzm of
Tsujimoto et al. (1995),
and a GBF+Salpeter IMF. Back.
(m)dm]-1, the stellar
yields pzm of
Tsujimoto et al. (1995),
and a GBF+Salpeter IMF. Back.