


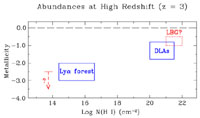
|
Figure 6. Summary of our current knowledge
of abundances at high red-shift.
Metallicity is on a log scale relative to solar and N(H I) is
the column density of neutral hydrogen measured in the
Lyman- |
Naturally recent advances in studies of objects at high red-shift supply
vital clues to the early evolution of galaxies, but, as
Pettini (1999)
has emphasised, our knowledge in this area is severely limited (see
Figure 6), giving rise to serious
observational selection effects. The Lyman forest comes from
condensations in the intergalactic medium, possibly analogues of the
high-velocity H I clouds seen today
(Blitz et al. 1999),
and represents the majority of the baryonic matter in
the universe, while the damped
Lyman- (DLA) systems have a
co-moving density similar to that of disk galaxies today. Then there are
also the Lyman break galaxies, for which there is some information based
on the strength of their
stellar winds. Figure 7 shows the metallicities
of DLA systems, based on zinc abundance, plotted against red-shift, after
Pettini (1999).
When column-density weighted means are formed in distinct red-shift bins,
no evolution is detectable in the metallicity and there
is no obvious way of identifying what sort of objects these systems will
eventually become. Some clues could come from element:element ratios
like N/O or
(DLA) systems have a
co-moving density similar to that of disk galaxies today. Then there are
also the Lyman break galaxies, for which there is some information based
on the strength of their
stellar winds. Figure 7 shows the metallicities
of DLA systems, based on zinc abundance, plotted against red-shift, after
Pettini (1999).
When column-density weighted means are formed in distinct red-shift bins,
no evolution is detectable in the metallicity and there
is no obvious way of identifying what sort of objects these systems will
eventually become. Some clues could come from element:element ratios
like N/O or  /Fe.
Here the difficulty lies in correcting for depletion from the gas phase
on to dust, which can be estimated (when not too large) from the ratio
of Zn to Cr and Fe, since their intrinsic relative abundances are
usually constant. According to
Vladilo (1998)
and Pettini et al.
(1999a,
b),
the resulting relative abundances of
silicon and iron are pretty much solar (or like the Magellanic Clouds
and the "anomalous" halo stars referred to above), suggesting that they
are destined to
become Im galaxies rather than large spirals. The behaviour of N/Si vs
Si/H also shows a resemblance to the behaviour of N/O vs. O/H in
irregular and blue compact galaxies with perhaps an even greater
scatter around the normal primary-secondary pattern than is found in
irregulars and BCGs
(Lu, Sargent & Barlow
1998).
/Fe.
Here the difficulty lies in correcting for depletion from the gas phase
on to dust, which can be estimated (when not too large) from the ratio
of Zn to Cr and Fe, since their intrinsic relative abundances are
usually constant. According to
Vladilo (1998)
and Pettini et al.
(1999a,
b),
the resulting relative abundances of
silicon and iron are pretty much solar (or like the Magellanic Clouds
and the "anomalous" halo stars referred to above), suggesting that they
are destined to
become Im galaxies rather than large spirals. The behaviour of N/Si vs
Si/H also shows a resemblance to the behaviour of N/O vs. O/H in
irregular and blue compact galaxies with perhaps an even greater
scatter around the normal primary-secondary pattern than is found in
irregulars and BCGs
(Lu, Sargent & Barlow
1998).
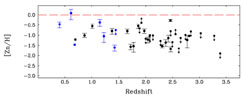
|
Figure 7. Zn abundance against red-shift for 40 DLAs from Pettini et al. (1999). Courtesy Max Pettini. |
What are the consequences of the new star formation rate density
(Fig 1) for "metal"
production and global chemical evolution? To begin with, the SFR which I
shall call
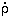 *(conv.) is based on the rest-frame UV
luminosity density combined with a Salpeter power-law IMF between 0.1 and
100 M
*(conv.) is based on the rest-frame UV
luminosity density combined with a Salpeter power-law IMF between 0.1 and
100 M . The
co-moving metal
production-rate density is then usually deduced by dividing by the magic
number of 42 (shades of The Hitch-Hiker's Guide to the
Galaxy), which comes from models of supernova yields in the range
of 10 to 100 M
. The
co-moving metal
production-rate density is then usually deduced by dividing by the magic
number of 42 (shades of The Hitch-Hiker's Guide to the
Galaxy), which comes from models of supernova yields in the range
of 10 to 100 M or so, and I shall call this metal production rate
or so, and I shall call this metal production rate
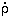 Z(conv.). The overall yield then amounts to
Z(conv.). The overall yield then amounts to
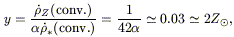
| (3) |
where 
 0.7 is the lock-up
fraction. Such a high yield is excessive
for the solar neighbourhood (although it may be suitable for
intra-cluster gas)
and so people modelling Galactic chemical evolution generally either use
a steeper
slope, a smaller lower mass limit or assume that stars above 40 or 50
M
0.7 is the lock-up
fraction. Such a high yield is excessive
for the solar neighbourhood (although it may be suitable for
intra-cluster gas)
and so people modelling Galactic chemical evolution generally either use
a steeper
slope, a smaller lower mass limit or assume that stars above 40 or 50
M lock the bulk of their element production in black holes. So the true
rate of "metal" production should be
lock the bulk of their element production in black holes. So the true
rate of "metal" production should be

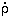 Z(conv.), where
Z(conv.), where

 1 is
some correction factor depending on your favourite model of galactic
chemical evolution. Finally, the true star formation rate density should
be corrected by
some factor
1 is
some correction factor depending on your favourite model of galactic
chemical evolution. Finally, the true star formation rate density should
be corrected by
some factor  , also
, also
 1, for the undoubted flattening
of the IMF power law somewhere below
1 M
1, for the undoubted flattening
of the IMF power law somewhere below
1 M , e.g.
Fukugita, Hogan &
Peebles (1998)
have
, e.g.
Fukugita, Hogan &
Peebles (1998)
have  = 0.65, but this does
not influence the conversion factor (at least
to first order) because it is mainly just the massive stars that produce
both the metals and the UV luminosity.
= 0.65, but this does
not influence the conversion factor (at least
to first order) because it is mainly just the massive stars that produce
both the metals and the UV luminosity.
With these preliminaries, we can use the data supplied by
Pettini (1999)
to draw up the following inventory of stars and metals for the present
epoch and for a red-shift of 2.5, assuming
 = 0.67,
= 0.67,
 = 0.65.
= 0.65.
| z = 0 | z = 2.5 | |
 *
= *
= 


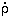 *(conv.) dt *(conv.) dt
| 3.6 × 108
M Mpc-3
Mpc-3
| 9 × 107
M Mpc-3
Mpc-3
|
 *
= *
=  * /
7.7 × 1010 h502 * /
7.7 × 1010 h502
| .0047h50-2 | .0012h50-2 |
 *(FHP 98) *(FHP 98)
| .0049h50-1 | |
 Z
= y Z
= y
 *
= *
=   * /
(42 * /
(42
 ) )
| 2.0 × 107
 M
M Mpc-3
Mpc-3
| 5 × 106
 M
M Mpc-3
Mpc-3
|
 Z (predicted) Z (predicted)
| 2.6 × 10-4
 h50-2
h50-2
| 6.5 × 10-5
 h50-2
h50-2
|
 Z (stars,
Z = Z Z (stars,
Z = Z ) )
| 1.0 × 10-4 h50-1 | |
 Z (hot gas,
Z =
0.3Z Z (hot gas,
Z =
0.3Z ) )
| 1.7 × 10-4h50-1.5 | |
-> 0.4 

 1 1
| ||
 Z (DLA,
Z =
0.07Z Z (DLA,
Z =
0.07Z ) )
| 3 × 10-6 h50-1 | |
 Z (Ly. forest,
Z =
0.003Z Z (Ly. forest,
Z =
0.003Z ) )
| 4 × 10-6 h50-2 | |
 Z (Ly. break gals,
Z =
0.3Z Z (Ly. break gals,
Z =
0.3Z ) )
| ? | |
 Z (hot gas) Z (hot gas)
| ? | |
The z = 0 column shows a fair degree of consistency. We can live
with  = 1
if we wish to explain a metal content of intergalactic gas as high as
suggested by Mushotzky & Loewenstein, or we can take this as a firm
upper limit because we do not
know if there is that much "metal" in intergalactic gas.
= 1
if we wish to explain a metal content of intergalactic gas as high as
suggested by Mushotzky & Loewenstein, or we can take this as a firm
upper limit because we do not
know if there is that much "metal" in intergalactic gas.
Somewhat more troubling questions arise at red-shift 2.5, however, as
Pettini (1999)
has already pointed out. It now seems that about a quarter of the stars
have already been formed by then (in ellipticals, bulges and
thick disks?), but known entries in the table only account for 10 per
cent of the
resulting metals (if  = 1) or
25 per cent (if
= 1) or
25 per cent (if  = 0.4). This
is a good
measure of the incompleteness in our knowledge of the distribution of
the elements at substantial red-shifts.
= 0.4). This
is a good
measure of the incompleteness in our knowledge of the distribution of
the elements at substantial red-shifts.
I thank Max Pettini for supplying data and for enlightening discussions.