Lauer and Postman
[28],
[37]
presented distances of the Brightest Cluster Galaxies (BCG's) of a sample of
119 Abell [2],
[3]
clusters to cz < 15,000 km s-1. Following work of
[18] and
[19],
they found that the
luminosity L of these galaxies within an aperture of radius 10
h-1kpc correlated with the logarithmic slope
of the surface brightness profile
. This yields a distance
indicator with an error of 15 - 20%, depending on
the value of . Their
sample was full-sky (or as much so as the zone of avoidance would allow)
and volume-limited, and great effort was taken to obtain and reduce the data
as uniformly as possible.
To their great surprise, the sample showed a strong signature of bulk
flow, with an amplitude of 764 ± 160 km s-1
[6],
towards l = 341°, b = +49°. This was much larger
than one might expect, given the effective depth of the sample of
8000 km s-1; indeed,
[14] and
[44]
both showed that a bulk flow with the
statistical significance of that of Lauer-Postman ruled out a whole
series of cosmological models at the
> 95% confidence level.
As a follow-up to this survey, Tod Lauer, Marc Postman and I are
extending the sample to
cz = 24, 000 km s-1. The sample now consists of 529
BCG's, an increase of more than a factor of 4 from the
original l19 (the Abell cluster catalog has the beautiful feature of
being volume-limited, at least to
moderate redshifts, and this increase in number of clusters is almost
exactly the increase in volume). The
photometry for this sample is all in hand, and redshifts for all BCG's
are nearly complete. Barring
unseen systematic effects (which we've worked very hard to minimize), we
should be able
to measure the bulk flow on these scales to 130 km s-1 or
so. We have also measured velocity
dispersions for the BCG's, with preliminary indications that this
reduces the scatter in the L -
relation, in analogy to the Dn -
relation. The sky
distribution of this sample is shown in Fig. 4a.
|
Figure 4. a. The BCG sample with
z < 0.08. The substantial region devoid of clusters in the
general direction of the Galactic center is due to the difficulty in
finding clusters in regions of high
stellar density, and is a general feature of the Abell
catalogue. b. The sky distribution in Galactic
coordinates of galaxies in the Sb, Sc shell sample at cz
|
As Fig. 3, and the controversy that the Lauer-Postman result have engendered, make clear, the comparison of various measurements of bulk flows with one another is non-trivial.
The velocity field has components on all scales; it is not purely dipolar in nature. The geometry of any given sample couples to various multipoles of the velocity field (the sparser the sampling is, the larger the extent to which this is true), and therefore not all bulk flow measurements measure the same quantity [48]. Thus [38] published a bulk flow analysis of 13 Type 1a supernovae, which appear to be standard candles to an accuracy of ~ 5% [39]. Their results were inconsistent with that found by Lauer & Postman at the 99% confidence level, assuming that the velocity field was describable by a pure bulk flow plus small-scale incoherent noise. However, the two surveys sample space really very differently, and therefore are very differently sensitive to components of the velocity field on scales smaller than the dipole. Watkins & Feldman [48] calculated the expectation value of the dot product of the bulk flows each measured, normalized by the expectation value of each bulk flow separately:
| (14) |
This quantity, a sort of dimensionless covariance between the two bulk
flow measurements, would be close
to unity if these two surveys were indeed measuring the same
quantity. The results depend on the power
spectrum assumed. If one assumes "realistic" power spectra, the quantity
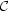is of the order of
10%, but as
mentioned above, the Lauer-Postman result is inconsistent with most
ordinary power spectra. Watkins &
Feldman thus also consider a power spectrum with a huge bump at large
scales; in such a model, the
relative importance of small-scale components of the velocity field is
reduced, but the quantity
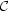is still only 35%.