


4.3. Morphology of stellar deaths
The evolution of stars is determined primarily by their mass, because
evolutionary timescales are set by the rate of consumption of the
nuclear fuel. Figure 13 shows the evolutionary
tracks of 1 M ,
5 M
,
5 M , and 25
M
, and 25
M stellar
models in the luminosity-effective temperature plane. It is clear that
certain phases of stellar evolution are characterized by considerable
mass ejection, either via relatively gentle processes such as radiation
pressure on atomic lines or on dust, or through stellar explosions. A
process of the former type produces the objects known as planetary
nebulae - shells of ejected matter from giant stars that are ionized
by the radiation emitted by the hot
(
stellar
models in the luminosity-effective temperature plane. It is clear that
certain phases of stellar evolution are characterized by considerable
mass ejection, either via relatively gentle processes such as radiation
pressure on atomic lines or on dust, or through stellar explosions. A
process of the former type produces the objects known as planetary
nebulae - shells of ejected matter from giant stars that are ionized
by the radiation emitted by the hot
( 30,000 K)
central core. The explosions known as Type II supernovae are the
result of the dynamical collapse of the iron cores of massive stars, and
the ejected nebulae form the objects known as supernova remnants.
30,000 K)
central core. The explosions known as Type II supernovae are the
result of the dynamical collapse of the iron cores of massive stars, and
the ejected nebulae form the objects known as supernova remnants.
 |
Figure 13. Evolutionary tracks of 1
M |
One of the most striking phenomena revealed by HST observations of
stellar deaths and of stars in their late stages of evolution is the
fact that axisymmetric nebulae are extremely common. This
observation remains true even after recognizing that the morphologies
are blurred by projection effects, and by the lines used to obtain the
image (i.e., the [O III] image may look different than the
[N II] image). Furthermore, axisymmetry is found not only in
planetary nebulae (PNe), that are formed by intermediate-mass stars (1-8
M ), but also
around Luminous Blue Variables (LBVs), that are extremely massive stars
(~ 100 M
), but also
around Luminous Blue Variables (LBVs), that are extremely massive stars
(~ 100 M ), and
supernovae (SNe), that represent the death of massive stars (8-30
M
), and
supernovae (SNe), that represent the death of massive stars (8-30
M ).
).
Eta Carinae
( Car), for
example, belongs to the small class of very massive stars known as
Luminous Blue Variables. These stars are believed to represent a rapid
evolutionary phase, in which the stars experience severe mass loss
(losing many solar masses in ~ 104 years), sometimes via
giant outbursts (e.g.,
Davidson and
Humphreys 1997).
In the 1840s
Car), for
example, belongs to the small class of very massive stars known as
Luminous Blue Variables. These stars are believed to represent a rapid
evolutionary phase, in which the stars experience severe mass loss
(losing many solar masses in ~ 104 years), sometimes via
giant outbursts (e.g.,
Davidson and
Humphreys 1997).
In the 1840s
 Car suffered
such an outburst, increasing in brightness by several magnitudes and
ejecting a large amount of material. The star stabilized around 1870
(except for a minor eruption between 1887 and 1895). The precise cause
for this giant outburst is still unknown, but it has produced a
spectacular, bipolar nebula commonly referred to as the Homunculus
(Fig. 14). In addition to the Homunculus, the
HST images revealed for the first time the presence of a ragged ejecta
disk around the "waist" of the hourglass structure, composed, at least
partly, of radial streaks. The total mass in the equatorial disk has
been estimated (based on conventional gas to dust ratios) to be at least
0.1-0.2 M
Car suffered
such an outburst, increasing in brightness by several magnitudes and
ejecting a large amount of material. The star stabilized around 1870
(except for a minor eruption between 1887 and 1895). The precise cause
for this giant outburst is still unknown, but it has produced a
spectacular, bipolar nebula commonly referred to as the Homunculus
(Fig. 14). In addition to the Homunculus, the
HST images revealed for the first time the presence of a ragged ejecta
disk around the "waist" of the hourglass structure, composed, at least
partly, of radial streaks. The total mass in the equatorial disk has
been estimated (based on conventional gas to dust ratios) to be at least
0.1-0.2 M , but
this could underestimate the mass significantly.
, but
this could underestimate the mass significantly.
 |
Figure 14. The Luminous Blue Variable star Eta Carinae, HST/WFPC2, 1994. Credit: NASA and J. Hester (Arizona State University). http://hubblesite.org/newscenter/archive/1994/09/ |
Recent spectroscopic observations of
 Car by the
Space Telescope Imaging Spectrograph (STIS) on board HST showed that the
spectrum could be fitted well using a model with a mass-loss rate of
~ 10-3
M
Car by the
Space Telescope Imaging Spectrograph (STIS) on board HST showed that the
spectrum could be fitted well using a model with a mass-loss rate of
~ 10-3
M yr-1. The minimum mass of the system is currently estimated
to be 120
M
yr-1. The minimum mass of the system is currently estimated
to be 120
M (Hillier et al. 2001).
(Hillier et al. 2001).
On the basis of photometric and radial velocity variations (in
particular the disappearance of
high-excitation lines like He I, Fe III) and x-ray
observations, it appears that
 Car is a
binary system, with an orbital period of 5.52 years
(Damineli,
Conti, and Lopes 1997,
Damineli et
al. 2000,
Ishibashi et
al. 1999).
The mass of the secondary is not known, but it may be less the 30
M
Car is a
binary system, with an orbital period of 5.52 years
(Damineli,
Conti, and Lopes 1997,
Damineli et
al. 2000,
Ishibashi et
al. 1999).
The mass of the secondary is not known, but it may be less the 30
M (Hillier et
al. 2001).
(Hillier et
al. 2001).
Eta Carinae is an extremely enigmatic object on many fronts. However,
the morphology of its ejected nebula becomes particularly intriguing
when we realize that HST observations reveal almost identical nebular
morphologies in objects of very different mass and evolutionary
history. One of the best known of these is the supernova 1987A
(SN 1987A) in the Large Magellanic Clouds (see, for example,
McCray 2003).
The supernova was first observed in February 1987 (and
hence 3 years prior to the launch of HST) and was immediately
classified as a Type II Supernova (SN II; representing the
collapse of the iron core of a massive star) by virtue of its strong
hydrogen lines (coming from the hydrogen-rich envelope). For the first
time, the detection of neutrino events (formed copiously as matter
rapidly neutronizes) directly confirmed the association between
SNe II and core collapses of massive stars. The exploding star
itself, SK -69°202, had actually been observed prior to the
explosion to be a B3 blue supergiant, with a luminosity of
L  1.1 ×
105
L
1.1 ×
105
L . Since the
HST launch, SN 1987A has become a prime target for the telescope,
being the nearest supernova in modern times. The HST observations have
revealed a remarkable system of circumstellar rings surrounding the
bright center
(Fig. 15;
Burrows et
al. 1995,
Pun 1997).
These rings reflect the morphology of material ejected by the
supernova progenitor a couple of tens of thousands of years before the
explosion
(Burrows et
al. 1995).
This can be inferred from the fact that the central ring is expanding at
about 10 km s-1
(Crotts and
Heathcote 1991)
and it currently has a radius of about
6.3 × 1017 cm. While a full explanation for the
formation of the rings is still lacking, there is very little doubt that
what we are observing is a bipolar structure, in which the inner ring
marks the narrow "waist," while the larger rings are somehow "painted"
on the bipolar lobes, or possibly mark their edges. The entire structure
is thus very similar to the one observed in
. Since the
HST launch, SN 1987A has become a prime target for the telescope,
being the nearest supernova in modern times. The HST observations have
revealed a remarkable system of circumstellar rings surrounding the
bright center
(Fig. 15;
Burrows et
al. 1995,
Pun 1997).
These rings reflect the morphology of material ejected by the
supernova progenitor a couple of tens of thousands of years before the
explosion
(Burrows et
al. 1995).
This can be inferred from the fact that the central ring is expanding at
about 10 km s-1
(Crotts and
Heathcote 1991)
and it currently has a radius of about
6.3 × 1017 cm. While a full explanation for the
formation of the rings is still lacking, there is very little doubt that
what we are observing is a bipolar structure, in which the inner ring
marks the narrow "waist," while the larger rings are somehow "painted"
on the bipolar lobes, or possibly mark their edges. The entire structure
is thus very similar to the one observed in
 Car.
Car.
 |
Figure 15. Rings around Supernova 1987A, HST/WPFC2, February 1994. Credit: NASA and C. Burrows (ESA/STScI). http://hubblesite.org/newscenter/archive/1994/22/ |
Bipolar structures have not been restricted only to massive stars,
however. Some planetary nebulae and symbiotic nebulae exhibit
morphologies that are almost identical to those of
 Car and
SN 1987A. Some of the best examples are probably My Cn18
(Fig. 16; the "hourglass" nebula, usually
classified as a planetary nebula), and the "Southern Crab"
(Fig. 17), now recognized as a symbiotic nebula
(Corradi et
al. 2001).
Car and
SN 1987A. Some of the best examples are probably My Cn18
(Fig. 16; the "hourglass" nebula, usually
classified as a planetary nebula), and the "Southern Crab"
(Fig. 17), now recognized as a symbiotic nebula
(Corradi et
al. 2001).
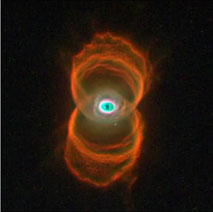 |
Figure 16. Planetary Nebula MyCn18, The
Hourglass Nebula, HST/WFPC2.
Credit: NASA, R. Sahai, J. Trauger (JPL) and the WFPC2
Science Team. |
 |
Figure 17. Nebula surrounding the symbiotic star system He2-104, the Southern Crab Nebula, HST/WFPC2, May 1999. Credit: NASA and R. Corradi (Instituto de Astrofisica de Canarias, Tenerife, Spain), M. Livio (Space Telescope Science Institute), U. Munari (Osservatorio Astronomico di Padova-Asiago, Italy), H. Schwarz (Nordic Optical Telescope, Canarias, Spain). http://hubblesite.org/newscenter/archive/1999/32/ |
Planetary nebulae represent the late stages in the lives of stars of
about 1-8
M . At that
phase, the stars eject their outer envelopes exposing the hot cores
(T
. At that
phase, the stars eject their outer envelopes exposing the hot cores
(T  30,
000 K), which, in turn, ionize the nebulae causing them to
fluoresce. Symbiotic nebulae have at their centers symbiotic binary
systems, consisting typically of a red supergiant and a white dwarf that
provides the ionizing radiation.
30,
000 K), which, in turn, ionize the nebulae causing them to
fluoresce. Symbiotic nebulae have at their centers symbiotic binary
systems, consisting typically of a red supergiant and a white dwarf that
provides the ionizing radiation.
The main question that arises, therefore, is: What is (are) the mechanism(s) that is (are) capable of producing such bipolar morphologies in stars of different masses, and different ages and evolutionary histories? The two main mechanisms that have been proposed are: (1) Interacting winds in the presence of an equatorial to polar density contrast, and (2) Magnetic tension of a toroidal field (see Balick and Frank 2002, for a more extensive discussion).
Let us first examine the interacting winds model. The original "interacting winds" model for planetary nebulae (Kwok 1982, Kahn 1982) suggested that old, intermediate-mass stars, in the phase of evolution known as the asymptotic giant branch (AGB), first emit a slow (~ 20 km s-1; of the order of the escape velocity from the AGB star's surface) wind, followed by a fast wind (~ 1000 km s-1), once the hot and compact nucleus (the AGB star's core) is exposed. The fast wind catches up with the slowly moving material and shocks it. Balick (1987) proposed that when the interacting winds are allowed to operate in the presence of a density contrast between the equator and the pole, a variety of axially symmetric morphologies can be obtained. The idea is that, for reasons that will be explored below, the slow wind contains a non-spherical density distribution, with material being denser around the equator than in the polar direction. Consequently, the (spherically symmetric) fast wind can penetrate more easily at the poles, forming an axisymmetric nebula. Numerical simulations have shown that when a range of density contrasts (between the equatorial and polar directions) is used, and, in addition, the nebular inclination with respect to the line of sight is taken into consideration, most of the observed morphologies can be reproduced (Soker and Livio 1989, Icke et al. 1992, Frank et al. 1993, Mellema 1995, Dwarkadas et al. 1996).
The second class of models involves the action of a magnetic field.
The toroidal field component in an outflow from a star is given by (Parks 1991)
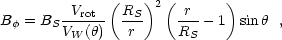 |
(11) |
where RS, BS are the stellar radius
and the surface magnetic field,
Vrot is the equatorial rotational velocity and
VW( )
is the wind terminal velocity. Consequently, the ratio of the toroidal
to radial component increases like (r/RS) at
large distances, leading potentially to an axisymmetric configuration,
by the fact that magnetic stresses can slow down the flow in the
equatorial direction (while not interfering with the polar
direction). The key physical parameters determining the obtained
morphology are the stellar rotation rate, the ionizing radiation, and
the stellar magnetic field. These can be expressed by:
)
is the wind terminal velocity. Consequently, the ratio of the toroidal
to radial component increases like (r/RS) at
large distances, leading potentially to an axisymmetric configuration,
by the fact that magnetic stresses can slow down the flow in the
equatorial direction (while not interfering with the polar
direction). The key physical parameters determining the obtained
morphology are the stellar rotation rate, the ionizing radiation, and
the stellar magnetic field. These can be expressed by:
 rot /
rot /
 crit,
F*, and
crit,
F*, and
 (Chevalier and
Luo 1994,
Garcia-Segura et
al. 1999).
Here
(Chevalier and
Luo 1994,
Garcia-Segura et
al. 1999).
Here  crit is
the critical (Keplerian) angular velocity,
crit is
the critical (Keplerian) angular velocity,
 crit =
(GMS /
RS3)1/2,
F* is the average flux of
Ly
crit =
(GMS /
RS3)1/2,
F* is the average flux of
Ly photons (of order
1045-1047 s-1 for typical PNe nuclei),
and
photons (of order
1045-1047 s-1 for typical PNe nuclei),
and
 is the ratio of the
magnetic energy density to the kinetic energy density in the wind (e.g.,
Begelman and Li
1992)
is the ratio of the
magnetic energy density to the kinetic energy density in the wind (e.g.,
Begelman and Li
1992)
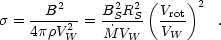 |
(12) |
Let us now examine the effects of each one of these parameters.
For the rotation to have a significant effect and produce a bipolar
morphology, the star needs to rotate at a significant fraction of its
breakup speed
( rot /
rot /
 crit
crit
 0.5). Under these
conditions, conservation of angular momentum results in a significant
focusing of the wind towards the equatorial plane, leading to an
equatorially compressed outflow (e.g.,
Bjorkman and
Cassinelli 1993,
Owocki et
al. 1994,
Livio 1994).
The concomitant equator to pole density contrast leads to bipolar
morphologies.
0.5). Under these
conditions, conservation of angular momentum results in a significant
focusing of the wind towards the equatorial plane, leading to an
equatorially compressed outflow (e.g.,
Bjorkman and
Cassinelli 1993,
Owocki et
al. 1994,
Livio 1994).
The concomitant equator to pole density contrast leads to bipolar
morphologies.
Ionization does not, in itself, produce a bipolar morphology. Rather, ionization fronts tend to excite instabilities (similar to the Rayleigh-Taylor instability or the instability discussed by Vishniac 1983), which, in turn, produce finger-shaped structures and dense "knots." Observations of a number of relatively nearby planetary nebulae, and in particular of the "Helix" nebula, reveal that such dense knots are probably very common (O'Dell et al. 2003), Speck et al. 2002)).
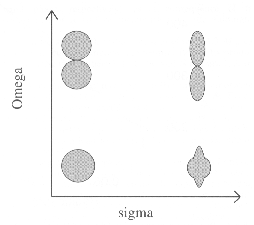 |
Figure 18. A schematic distribution of the
planetary nebulae morphology in the rotation
( |
The toroidal magnetic field is carried by the fast wind and it can, in
principle, produce a bipolar morphology even if the slow wind is
spherically symmetric. Basically, as the magnetic energy density of the
shocked wind becomes larger than the thermal energy density, the flow
becomes (due to the increasing importance of the toroidal component)
bipolar, with an increased collimation as the value of
 is increased. Numerical
simulations
(Garcia-Segura et
al. 1999)
show that qualitatively, the morphologies obtained for different
combinations of
is increased. Numerical
simulations
(Garcia-Segura et
al. 1999)
show that qualitatively, the morphologies obtained for different
combinations of
 rot /
rot /
 crit and
crit and
 are as shown in
Fig. 18. The minimum field required to produce
magnetic shaping was found to be of order
(Chevalier and
Luo 1994)
are as shown in
Fig. 18. The minimum field required to produce
magnetic shaping was found to be of order
(Chevalier and
Luo 1994)
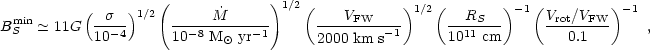 |
(13) |
where VFW is the velocity of the fast wind.
From eq. 13 we see that if the star rotates too slowly, the minimum required field may be unattainable. Similarly, if the star does not rotate at a significant fraction of its breakup speed, an equatorially compressed outflow is not formed. The question of: What is the mechanism that produces highly bipolar outflows? can therefore be reduced to: What causes the star to rotate close to breakup? or: What can produce a strong density contrast between the equatorial and polar directions in the slow wind?
One obvious possibility is: binary companions!
Companions to the central star can act in several ways to aid in the
formation of bipolar morphologies:
(1) For binaries that were initially relatively close (separation
less than ~ 1000
R ), so that the
primary could fill its Roche lobe (the critical potential surface beyond
which mass transfer onto the companion occurs) during the asymptotic
giant branch (AGB) phase, an unstable mass transfer ensues. As a result,
the companion and the AGB star's core start spiralling-in inside a
common envelope (see, e.g., a review by
Iben and Livio
1993).
This has two effects. First, the envelope of the primary can be spun-up
to angular velocities of the order of
), so that the
primary could fill its Roche lobe (the critical potential surface beyond
which mass transfer onto the companion occurs) during the asymptotic
giant branch (AGB) phase, an unstable mass transfer ensues. As a result,
the companion and the AGB star's core start spiralling-in inside a
common envelope (see, e.g., a review by
Iben and Livio
1993).
This has two effects. First, the envelope of the primary can be spun-up
to angular velocities of the order of
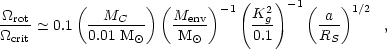 |
(14) |
where MC is the companion's mass, Menv is the mass of the giant's envelope, MS Kg2 RS2 is the star's moment of inertia and a is the initial separation between the giant and the companion. Equation 14 shows that even brown dwarf (sub-stellar) companions can bring the envelope close to critical rotation. Second, since the envelope mass is ejected (due to orbital energy deposition) primarily close to the orbital plane (because angular momentum is also deposited into the envelope), the common envelope phase can generate an equator-to-pole density contrast. Hydrodynamic simulations of common envelope evolution reveal that during the late stages about 80% of the mass is ejected within 30° of the binary orbital plane (e.g., Terman, Taam, and Hernquist 1994, 1995, Rasio and Livio 1996). One can expect that due to cooling, the mass will sink even more toward the orbital plane at later times.
Another possibility for the presence of a higher equatorial density, in principle at least, that does not involve binary companions, is the inner rim of the protostellar disk. If the outer part of the protostellar disk survives till late stages in the stellar life (which may not be difficult in the case of massive, short-lived stars), then the fast wind could interact with the inner rim of this disk (Pringle 1989). In a few planetary nebulae (for example, the "Red Rectangle" and the "Egg Nebula," Bond et al. 1996, Thompson et al. 1997, respectively). HST observations reveal the presence of relatively large disks, similar to the ones observed in young stellar objects.
In at least some symbiotic nebulae (e.g., M2-9), the bipolar morphology may reflect the action of the white dwarf companion. The white dwarf accretes from the wind of the AGB star, an accretion disk is formed, and the disk powers a mildly collimated fast wind, which in turn produces the bipolar morphology (Soker and Rappaport 2001, Livio and Soker 2001).
The conclusion from this discussion is that several mechanisms are
capable, in principle at least, to produce the observed bipolar
morphologies. Different mechanisms may be operating in different
systems. In some cases, we can look forward to the future and expect
more definitive answers to emerge. For example, in SN 1987A, the
supernova blast wave will eventually hit the entire inner ring, and the
luminosity that will be generated by this interaction will illuminate
the entire SN vicinity
(McCray 2003).
A few brightening spots, where the blast wave has already hit
protrusions on the ring, have been observed by HST
(Fig. 19; see also
Panagia 2002).
Generally, a transmitted shock at normal incidence is
expected to propagate into the ring with a speed of
Vring  (no / nring)1/2
Vblast (where no,
nring are the number densities of the circumstellar
matter and the ring, respectively, and
Vblast is the blast wave velocity). For SN 1987A,
Vblast ~ 4000 km s-1,
no ~ 150 cm-3,
nring ~ 104 cm-3 giving
Vring ~ 500 km s-1. Eventually, the
H
(no / nring)1/2
Vblast (where no,
nring are the number densities of the circumstellar
matter and the ring, respectively, and
Vblast is the blast wave velocity). For SN 1987A,
Vblast ~ 4000 km s-1,
no ~ 150 cm-3,
nring ~ 104 cm-3 giving
Vring ~ 500 km s-1. Eventually, the
H flux from the ring is
expected to be more than 30 times higher than today
(FH
flux from the ring is
expected to be more than 30 times higher than today
(FH
 3 ×
10-12 erg cm-2 s-1), and even brighter
in UV lines
(Luo, McCray, and
Slavin 1994).
This ionizing flux will turn the circumstellar matter into an emission
nebula, thus revealing its distribution and velocity field, and
hopefully allowing for a reconstruction of the mass-loss history of the
system. In all of this, HST will provide a front seat view.
3 ×
10-12 erg cm-2 s-1), and even brighter
in UV lines
(Luo, McCray, and
Slavin 1994).
This ionizing flux will turn the circumstellar matter into an emission
nebula, thus revealing its distribution and velocity field, and
hopefully allowing for a reconstruction of the mass-loss history of the
system. In all of this, HST will provide a front seat view.
The collapses of very massive stars are believed to produce stellar-mass black holes. In some cases, these collapses produce the most spectacular explosions in the universe since the "big bang" - Gamma Ray Bursts. The Hubble Space Telescope has played an important role in the attempts to understand the nature of these dramatic explosions. However, much more massive black holes are also produced in the universe quite commonly, and the questions of how they form and evolve have intrigued astronomers for decades. The initial answers had to come from large collections of stars like galaxies and stellar clusters.
Given the unprecedented resolution that HST provides, very crowded fields, like the centers of galaxies, were obvious targets. In particular, the early suggestive evidence that galactic centers harbor supermassive black holes (see Kormendy and Richstone 1995, for a review), virtually ensured that centers of galaxies will be observed extensively with HST. Indeed, these observations were carried out and proved to be extremely fruitful.