Interstellar dust grains are heated primarily by absorption of starlight
photons.
A small fraction of the absorbed starlight energy goes into luminescence
or ejection of a photoelectron, but the major part of the absorbed starlight
energy goes into heating (i.e., vibrationally exciting)
the interstellar grain material.
Figure 5 shows
grain temperature vs. time simulated for four sizes of carbonaceous grains
exposed to the average interstellar radiation field. For grain radii
a 
100
Å, individual photon absorptions are
relatively frequent, and the grain heat capacity is large enough that
the temperature excursions following individual photon absorptions
are relatively small; it is reasonable to approximate the grain temperature
as approximately constant in time. For
a 
50 Å
grains, however, the grain is able to cool appreciably
in the time between photon absorptions; as a result, individual photon
absorption events raise the grain temperature to well above the mean value.
To calculate the time-averaged infrared emission spectrum for these grains,
one requires the temperature distribution function (see, e.g.,
Draine & Li 2001).
 |
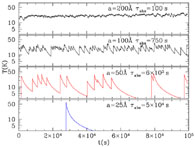Figure 5. A day in the life of 4
carbonaceous grains, exposed to the average starlight background.
|
Figure 6 shows the average emission spectrum of interstellar dust, based on observations of the FIR emission at high galactic latitudes, plus observations of a section of the galactic plane where the surface brightness is high enough to permit spectroscopy by the IRTS satellite (Onaka et al. 1996; Tanaka et al. 1996).
 |
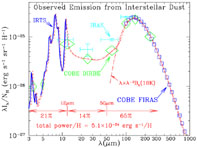Figure 6. Observed emission spectrum of diffuse interstellar dust in the Milky Way. Crosses: IRAS (Boulanger & Perault 1988); squares: COBE-FIRAS (Finkbeiner et al. 1999); diamonds: COBE-DIRBE (Arendt et al. 1998); heavy curve for 3-4.5 µm and 5 - 11.5µm: IRTS (Onaka et al. 1996, Tanaka et al. 1996). The total power ~ 5.1 × 10-24 ergs s-1 / H is estimated from the interpolated broken line. |
The similarity of the 5-15 µm
spectrum with that of reflection nebulae is evident
(see Figure 3).
Approximately 21% of the total power is radiated between 3 and 12
µm, with another ~ 14% between 12 and 50 µm.
This emission is from dust grains that are so small that single-photon
heating (see Figure 5) is important.
The remaining ~ 65 % of the power is radiated in the far-infrared,
with 
I peaking at ~ 130 µm.
At far-infrared wavelengths, the grain opacity varies as
~ -2,
and
I
-6 /
(ehc /
k Td
- 1) peaks at
= hc / 5.985
k Td = 134 µm (18 K / Td).
The emission spectrum for 18 K dust with opacity
-2
shown in Figure 6, provides a good fit to the
observed spectrum for
80 µm, but falls
far below the observed emission at
50 µm.
From Figure 6 it is apparent that ~ 60% of
the radiated power appears to originate from grains
which are sufficiently large (radii
a 100
Å ) so that individual photon absorption events do not appreciably
raise the grain temperature.
abs
is the mean time between photon absorptions.