


Armed with the SBBN-predicted primordial abundances, as well as with those in a variation on the standard model, we now turn to the observational data. The four light nuclides of interest, D, 3He, 4He, and 7Li follow different evolutionary paths in the post-BBN universe. In addition, the observations leading to their abundance determinations are different for all four. Neutral D is observed in absorption in the UV; singly-ionized 3He is observed in emission in galactic HII regions; both singly- and doubly-ionized 4He are observed in emission via their recombinations in extragalactic HII regions; 7Li is observed in absorption in the atmospheres of very metal-poor halo stars. The different histories and observational strategies provides some insurance that systematic errors affecting the inferred primordial abundances of any one of the light nuclides will not influence the inferred abundances of the others.
The post-BBN evolution of D is simple. As gas is incorporated into stars the very loosely bound deuteron is burned to 3He (and beyond). Any D which passes through a star is destroyed. Furthermore, there are no astrophysical sites where D can be produced in an abundance anywhere near that which is observed (Epstein, Lattimer, & Schramm 1976). As a result, as the universe evolves and gas is cycled through generations of stars, Deuterium is only destroyed. Therefore, observations of the deuterium abundance anywhere, anytime, provide lower bounds on its primordial abundance. Furthermore, if D can be observed in "young" systems, in the sense of very little stellar processing, the observed abundance should be very close to the primordial value. Thus, while there are extensive data on deuterium in the solar system and the local interstellar medium (ISM) of the Galaxy, it is the handful of observations of deuterium absorption in high-redshift (hi-z), low-metallicity (low-Z), QSO absorption-line systems (QSOALS) which are, potentially the most valuable. In Figure 3 the extant data (circa November 2001) are shown for D/H as a function of redshift from the work of Burles & Tytler (1998a, b), O'Meara et al. (2001), D'Odorico et al. (2001), and Pettini & Bowen (2001). Also shown for comparison are the local ISM D/H (Linsky & Wood 2000) and that for the presolar nebula as inferred from solar system data (Geiss & Gloeckler 1998, Gloeckler & Geiss 2000).
On the basis of our discussion of the post-BBN evolution of D/H, it would be expected that there should be a "Deuterium Plateau" at high redshift. If, indeed, one is present, the dispersion in the limited set of current data hide it. Alternatively, to explore the possibility that the D-abundances may be correlated with the metallicity of the QSOALS, we may plot the observed D/H versus the metallicity, as measured by [Si/H], for these absorbers. This is shown in Figure 4 where there is some evidence for an (unexpected!) increase in D/H with decreasing [Si/H]; once again, the dispersion in D/H hides any plateau.
 |
Figure 4. The deuterium abundance, D/H, versus metallicity ([Si/H]) for the same QSOALS as in Figure 3 (filled circles). Also shown for comparison are the D-abundances for the local ISM (filled square) and the solar system ("Sun"; filled triangle). |
Aside from observational errors, there are several sources of
systematic error which may account for the observed dispersion.
For example, the
Ly absorption of
HI in these systems
is saturated, potentially hiding complex velocity structure.
Usually, but not always, this velocity structure can be revealed
in the higher lines of the Lyman series and, expecially, in the
narrower metal-absorption lines. Recall, also, that the lines
in the Lyman series of DI are identical
to those of HI, only
shifted by
absorption of
HI in these systems
is saturated, potentially hiding complex velocity structure.
Usually, but not always, this velocity structure can be revealed
in the higher lines of the Lyman series and, expecially, in the
narrower metal-absorption lines. Recall, also, that the lines
in the Lyman series of DI are identical
to those of HI, only
shifted by  81
km/s. Given the highly saturated HI
Ly
81
km/s. Given the highly saturated HI
Ly , it may be difficult
to identify which, and how much, of the HI corresponds to
an absorption feature identified as
DI Ly
, it may be difficult
to identify which, and how much, of the HI corresponds to
an absorption feature identified as
DI Ly .
Furthermore, are such features really DI or,
an interloping, low column density HI-absorber? After all,
there are many more low-, rather than high-column density HI
systems. Statistically, the highest column density absorbers
may be more immune to these systematic errors. Therefore, in
Figure 5 are shown the very same D/H data, now
plotted against the neutral hydrogen column density. The three highest
column density absorbers (Damped
Ly
.
Furthermore, are such features really DI or,
an interloping, low column density HI-absorber? After all,
there are many more low-, rather than high-column density HI
systems. Statistically, the highest column density absorbers
may be more immune to these systematic errors. Therefore, in
Figure 5 are shown the very same D/H data, now
plotted against the neutral hydrogen column density. The three highest
column density absorbers (Damped
Ly Absorbers: DLAs) fail to
reveal the D-plateau, although it may be the case that some
of the D associated with the two lower column density systems
may be attributable to an interloper, which would reduce the
D/H inferred for them.
Absorbers: DLAs) fail to
reveal the D-plateau, although it may be the case that some
of the D associated with the two lower column density systems
may be attributable to an interloper, which would reduce the
D/H inferred for them.
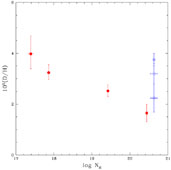 |
Figure 5. The same QSOALS D/H data as in Figures 3 & 4 versus the HI column density (log scale). The open circle symbols are for the original (D'Odorico et al. 2001) and the revised (Levshakov et al. 2002) D/H values for Q0347-3819. |
Actually, the situation is even more confused. The highest column density absorber, from D'Odorico et al. (2001), was reobserved by Levshakov et al. (2002) and revealed to have a more complex velocity structure. As a result, the D/H has been revised from 2.24 × 10-5 to 3.2 × 10-5 to 3.75 × 10-5. To this theorist, at least, this evolution suggests that the complex velocity structure in this absorber renders it suspect for determining primordial D/H. The sharp-eyed reader may notice that if this D/H determination if removed from Figure 5, there is a hint of an anticorrelation between D/H and NH among the remaining data points, suggesting that interlopers may be contributing to (but not necessarily dominating) the inferred DI column density.
However, the next highest column density HI-absorber
(Pettini & Bowen
2001),
has the lowest D/H ratio, at a value
indistinguishable from the ISM and solar system abundances.
Why such a high-z, low-Z system should have destroyed so
much of its primordial D so early in the evolution of the
universe, apparently without producing very many heavy elements,
is a mystery. If, for no really justifiable reason, this system
is arbitrarily set aside, only the three "UCSD" systems of Burles &
Tytler
(1998a,
b)
and O'Meara et
al. (2001)
remain. The weighted mean for these three absorbers is D/H = 3.0 ×
10-5.
O'Meara et al. note the larger than expected dispersion, even
for this subset of D-abundances, and they suggest increasing
the formal error in the mean, leading to: (D/H)P = 3.0 ±
0.4 × 10-5. I will be even more cautious;
when the SBBN predictions are compared with the primordial
abundances inferred from the data, I will adopt: (D/H)P =
3.0+1.0-0.5 × 10-5. Since the
primordial D abundance is sensitive to the baryon abundance (D/H

 -1.6),
even these perhaps overly generous errors will still result in
SBBN-derived baryon abundances which are accurate to 10 - 20%.
-1.6),
even these perhaps overly generous errors will still result in
SBBN-derived baryon abundances which are accurate to 10 - 20%.