While the large-scale structure and kinematics of nearby bulges can be studied from the ground, HST is required to reach scales of a few tens of parsecs. An HST/WFPC2 study of a large sample of bulges (mostly unbarred, Sa–Sbc) by Carollo et al. (1997, 1998) reveals a large variety of nuclear properties, even among early types. Some “classical” bulges exist, but in half the cases a bulge is not even clearly detected. i) Many early-type galaxies show no evidence for a smooth bulge (also dust lanes, spiral structure, etc); ii) 30% of bulges have an irregular central bright component with scattered star forming regions. Other nuclear star formation occurs, sometimes in ring-like structures, but it is unclear whether it is associated with the bulge or inner disk; iii) for types later than S0/a, half the objects have a resolved, compact central source (often associated with an elongated structure), the luminosity of which correlates with that of the host galaxy but not the type (typically brighter in star forming objects); iv) the brightest compact sources appear similar to young star clusters in the MV − Re plane, while fainter sources are intermediate between ellipticals and R1/4 bulges and globular clusters, possibly indicating an age sequence. Those sources are photometrically distinct from their surroundings and are not a simple steepening of the light profile. These facts suggest a late formation epoch for some bulges, possibly in disk-driven dissipative accretion events.
The nuclear light profiles of spheroids (bulges and ellipticals) is well described by the cusp slope γ (I(r) ∝ r−γ as r → 0; Byun et al. 1996). In the above sample, R1/4-like bulges have cusps and nuclear densities similar to ellipticals (at a given spheroid luminosity Ls), and also steeper cusp slopes as Ls is lowered (Faber et al. 1997, Carollo & Stiavelli 1998). Exponential-like bulges show the same (weaker) dependence on Ls, but they have smaller cusps and nuclear densities at a given luminosity (Fig. 5). As a group, they thus break the general trend among spheroids of increasing density with decreasing luminosity, a rare indication for a different formation mechanism. This does not indicate a simple evolution along the Hubble sequence, however, as it holds true for a given type.
 |
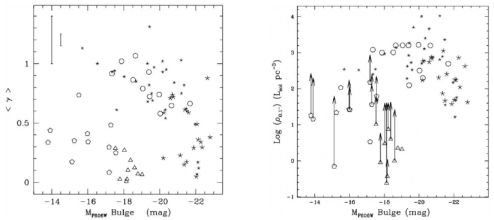Figure 5. Nuclear cusp slopes and densities of spheroids. Left: Average logarithmic cusp slope (0.1″–0.5″) versus spheroid absolute magnitude. Error bars are shown for galaxies with and without a central compact source. Right: Stellar density at 0.1″ (from deprojected analytic fits) versus spheroid absolute magnitude. Triangles and pentagons: Exponential-like bulges. Circles: R1/4-like bulges. Asterisks: Ellipticals. Reproduced with permission from Carollo & Stiavelli (1998). |
Magorrian et al. (1998) proposed the first central BH mass M• to spheroid mass Ms (or spheroid luminosity Ls) relation, showing that power-law galaxies (steep cusps) have smaller M• and M / L than core galaxies (shallow cusps). But the masses, based on ground-based kinematics and two-integral axisymmetric dynamical models, were overestimated. Using HST/STIS kinematics (resolving the sphere of influence of the BH) and three-integral models (allowing velocity anisotropy near the center) reduces the masses by a few. Essentially all galaxies require M• ∼ 0.001 Ms, suggesting a universal baryon fraction going in the BH. M• correlates significantly better with Ls than the total galactic luminosity, indicating that BHs are not related to disks. The correlation is also independent of bulge type (R1/4, exponential, pseudo), suggesting a close link between BH and bulge (spheroid) formation, independently of how the latter proceeds.
The relation between M• and (some measure of)
the central velocity dispersion σ is much tighter, although its exact
dependence is debated: M• ∝
σ3.8−4.8, with a steep slope favored
(Gebhardt et al. 2000a,
Ferrarese & Merritt
2000,
Merritt & Ferrarese
2001).
The scatter of ≈ 0.3 dex (at
fixed σ) is consistent with observational errors, indicating
negligible intrinsic scatter. The previous relation with
Ms can now be “understood”, since
Ms
Ls5/4
(Faber et al. 1987)
and Ls
σ4 (FJ), hence Ms
σ5
also. Bulges (spheroids) can now be seen as populating a 2D plane in a
4D space (logM•, logRe,
logσ, logL), the
M• − σ relation being an edge-on
projection of this plane
(while the M• − Ls
relation is not, thus the larger
scatter). BH masses predicted from the M•
− σ relation are consistent with reverberation mapping
measurements in active galactic nuclei (AGN;
Gebhardt et al. 2000b,
Ferrarese et al. 2001),
indicating a close relationship between quiescent and active BHs, and
strengthening the link between BHs, AGN, and bulge (spheroid) formation
(e.g.
Silk & Rees 1998).
There have been many suggestions that bars can be destroyed by central masses, secularly building bulges over many generations, and moving galaxies along the Hubble sequence (e.g. Norman, Sellwood, & Hasan 1996). BH masses reported are however an order of magnitude lower than required (∼ 0.1% of Ms rather than a few). The central stellar clusters discussed above do have the right masses, but they would prevent bars from (re-)forming in late-type exponential bulges, inhibiting their growth and evolution into early-type R1/4 bulges unless there is a substantial accretion of cold material (the same applies to BHs). The omnipresence of bars (70% of galaxies; e.g. Seiger & James 1998) implies a very fast duty cycle, however, which seems unlikely. At the moment, the evidence is thus against bar (destruction)-driven secular evolution in bulges.