Copyright © 1997 by Annual Reviews. All rights reserved
|
| Annu. Rev. Astron. Astrophys. 1997. 35:
357-88 Copyright © 1997 by Annual Reviews. All rights reserved |
The nature of the discordant redshift members of compact groups has been a subject of debate for many years (eg. Burbidge & Burbidge 1961a, Burbidge & Sargent 1971, Nottale & Moles 1978, Sulentic 1983). If the frequency of discordant galaxies is inconsistent with the statistics of chance projection, it might signify the need for new physical theories (Arp 1987), or for gravitational amplification of background galaxies (Hammer & Nottale 1986). Initial estimates of the chance probability of finding discordant galaxies in groups like Stepfan's Quintet, Seyfert's Sextet and VV 172, were very small (Burbidge & Sargent 1971). However, such probabilities were recognized to be difficult to determine reliably, because the a priori probability of any particular configuration of galaxies is also very small (Burbidge & Sargent 1971). Only with a well-defined sample of groups and a complete characterization of selection effects, can meaningful estimates of the probabilities be made. The explicit selection criteria of the HCG catalog in principal makes this sample suitable for a quantitative statistical investigation of the discordant-redshift question. Sulentic (1987) first concluded that the number of discordant redshifts in the catalog is too large to explain by chance. On the other hand, Hickson et al. (1988a) and Mendes de Oliveira (1995), applying the selection criteria more rigorously, found no strong statistical evidence for this. Their result, however, may be biased by incompleteness in the HCG catalog: There seem to be too-few low surface brightness groups in the catalog, and the "missing" groups may have a higher fraction of discordant redshifts (Sulentic 1997).
In order to address the incompleteness issue, Iovino & Hickson (1996) combined observational results from both the HCG and SCG catalogs with Monte-Carlo simulations. Their technique exploits the unbiased nature of the SCG catalog and the complete redshift coverage of the HCG sample. They conclude that for all except the two highest-surface-brightness quintets (Stefan's Quintet and Seyfert's Sextet), the number of discordant redshifts is consistent with chance projections. For these two, the chance probabilities are low. However, for both of these systems there is independent physical evidence that the discordant galaxies are at the cosmological distances that correspond to their redshifts and are therefore not group members (Kent 1981, Wu et al. 1994).
One should not assume that the situation is now completely settled. Further studies will be possible when redshifts have been obtained for the SCG galaxies. There are still other questions that have not been adequately addressed, such as reported redshift quantization (Cocke & Tifft 1983). However, at this point it appears that the frequency of discordant galaxies does not require a new interpretation of galaxy redshifts. In fact, physical evidence suggests the opposite. The discordant galaxies all have physical properties consistent with a cosmological distance. For example those with higher redshift tend to be smaller and fainter than other members of the group, and vice versa (Mendes de Oliveira 1995).
6.2 Physical association and density
Because we can measure only three phase-space dimensions for galaxies in compact groups (two components of position and one of velocity), the groups are subject to projection effects. Because of this, they may not be physically dense, or even physically related systems.
The following interpretations have so far been suggested for compact groups:
Evidence for and against physical association and high density in the HCG sample, to 1988, was summarized by Hickson & Rood (1988), and by Walke and Mamon (1989) respectively. Since that time, several new results have emerged. From an analysis of optical images, Mendes de Oliveira & Hickson (1994) concluded that 43% of all HCG galaxies show morphological features indicative of interaction and/or merging, and that 32% of all HCGs contain three or more interacting galaxies. These percentages are likely to rise with more-detailed studies and sophisticated image analysis (Longo et al. 1994). This high frequency of interactions observed in compact groups is difficult to reconcile with the chance alignment and filament hypotheses, even if the alignments contain physical binaries (Mamon 1995).
The high fraction of HCGs showing diffuse X-ray emission is very strong evidence that a large fraction of these systems are physically dense, and are not transient configurations or projection effects. Although the exact numbers are not final, due to the faintness of the sources and the problems of contamination by sources associated with the individual galaxies, it seems evident that many groups are dense bound systems. The correlations seen between X-ray and optical properties, and the fact that the X-ray properties of compact groups are not inconsistent with those of clusters reinforces this conclusion.
Ostriker et al. (1995) have argued that the relatively low X-ray luminosities of compact groups might not be due to a low gas fraction, but instead could be understood if the groups are filaments seen in projection (Hernquist et al. 1995). However, Ponman et al. (1996) point out that in order to explain even the fainter compact groups, gas temperatures T ~ 1 keV and densities n ~ 10-4 cm-3 would be required. These appear to be ruled out by both observations (Briel & Henry 1995) and simulations (Diaferio et al. 1995, Pildis et al. 1996).
Even if compact groups are physically dense, they may not be as dense as they
appear. As mentioned in Section 2, a sample
of groups selected on the basis of
high apparent density will be biased by the inclusion of looser systems which
appear more compact due to geometrical or kinematic effects. Is this bias
large? Its magnitude can be estimated as follows: Consider n galaxies
randomly located within a circle of radius R on the sky. What is the
probability f (x, n) that they will fall within some circular
subarea of radius
x R? The answer can be obtained using analytic expressions
derived by
Walke & Mamon
(1989). From their Equations 1 and 6 (setting N = n and
ext = 1) we
obtain
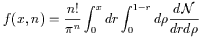
| (1) |
where
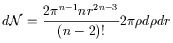
| (2) |
is the number of possible configurations with radius between r
and r + dr
and distance from the center between 
and + d. Here we have
neglected a small edge contribution that is unimportant for small values of
x (Walke and Mamon's case 3). This gives
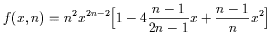
| (3) |
Now, an observer would infer a galaxy space density that is higher by a
factor 
=
x-3, so the average apparent space density enhancement is
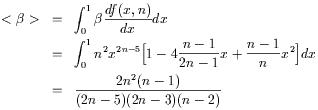
| (4) |
Thus we expect to typically overestimate the space density by about a factor of 12.0 for triplets (or quartets containing a physical binary), 3.2 for true quartets and 2.0 for quintets.