


As we look forward to the abundance (avalanche!) of high-quality observations that will test Inflation + CDM, we have to make sure the predictions of the theory match the precision of the data. In so doing, CDM + Inflation becomes a ten (or more) parameter theory. For astrophysicists, and especially cosmologists, this is daunting, as it may seem that a ten-parameter theory can be made to fit any set of observations. This is not the case when one has the quality and quantity of data that will be coming. The standard model of particle physics offers an excellent example: it is a nineteen-parameter theory and because of the high-quality of data from experiments at Fermilab's Tevatron, SLAC's SLC, CERN's LEP and other facilities it has been rigorously tested and the parameters measured to a precision of better than 1% in some cases. My worry as an inflationist is not that many different sets of parameters will fit the upcoming data, but rather that no set of parameters will!
In fact, the ten parameters of CDM + Inflation are an opportunity rather than a curse: Because the parameters depend upon the underlying inflationary model and fundamental aspects of the Universe, we have the very real possibility of learning much about the Universe and inflation. The ten parameters can be organized into two groups: cosmological and dark-matter (Dodelson et al, 1996).
 B
h2, the baryon density. Primeval deuterium
measurements and together with the theory of BBN imply:
B
h2, the baryon density. Primeval deuterium
measurements and together with the theory of BBN imply:
 B
h2 = 0.02 ± 0.002.
B
h2 = 0.02 ± 0.002.
 0.95; range of
predictions runs from 0.7 to 1.2
(Lyth & Riotto, 1996).
0.95; range of
predictions runs from 0.7 to 1.2
(Lyth & Riotto, 1996).
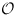 ( ±
10-3) or smaller
(Kosowsky & Turner,
1995).
( ±
10-3) or smaller
(Kosowsky & Turner,
1995).

 , the fraction of critical
density in neutrinos
(=
, the fraction of critical
density in neutrinos
(=  i
m
i
m i /
90h2). While the hot dark matter theory of structure
formation is not viable, it is possible that a small fraction of
the matter density exists in the form of neutrinos.
Further, small - but nonzero - neutrino masses are
a generic prediction of theories that unify the
strong, weak and electromagnetic interactions - and the
Super-Kamiokande Collaboration has presented
evidence that the at least one of the neutrino species has a
mass of greater than about 0.1eV, based upon the deficit
of atmospheric muon neutrinos
(Fukuda et al, 1998).
i /
90h2). While the hot dark matter theory of structure
formation is not viable, it is possible that a small fraction of
the matter density exists in the form of neutrinos.
Further, small - but nonzero - neutrino masses are
a generic prediction of theories that unify the
strong, weak and electromagnetic interactions - and the
Super-Kamiokande Collaboration has presented
evidence that the at least one of the neutrino species has a
mass of greater than about 0.1eV, based upon the deficit
of atmospheric muon neutrinos
(Fukuda et al, 1998).
 X, the
fraction of critical density in a smooth component
of unknown composition and negative pressure
(wX
X, the
fraction of critical density in a smooth component
of unknown composition and negative pressure
(wX  - 0.3). There is
mounting evidence for such a component, with the simplest example being
a cosmological constant (wX = - 1).
- 0.3). There is
mounting evidence for such a component, with the simplest example being
a cosmological constant (wX = - 1).
3.1. Present status of Inflation + CDM
A useful way to organize the different CDM models is by their dark-matter content; within each CDM family, the cosmological parameters vary. One list of models is:
 B = 0.05), but is
now ruled out (see Fig. 3).
B = 0.05), but is
now ruled out (see Fig. 3).
 CDM: This model has
extra radiation, e.g., produced by the decay of an unstable
massive tau neutrino (hence the name); here we take
g* = 7.45.
CDM: This model has
extra radiation, e.g., produced by the decay of an unstable
massive tau neutrino (hence the name); here we take
g* = 7.45.

 = 0.2 (about 5eV
worth of neutrinos).
= 0.2 (about 5eV
worth of neutrinos).

 = 0.6.
= 0.6.

|
Figure 3. Summary of viable CDM models, based upon CBR anisotropy and determinations of the present power spectrum of inhomogeneity (Dodelson et al, 1996). |
Figure 3 summarizes the viability of these
different CDM models,
based upon CBR measurements and current determinations of
the present power spectrum of inhomogeneity derived from
redshift surveys. sCDM is only viable for low values of the
Hubble constant (less than
55 km s-1 Mpc-1) and/or
significant tilt (deviation from scale invariance); the region
of viability for  CDM is similar
to sCDM, but shifted
to larger values of the Hubble constant (as large as
65 km s-1 Mpc-1). CDM has an island of viability
around
H0 ~ 60 km s-1 Mpc-1 and
n ~ 0.95. CDM
can tolerate the largest values of the Hubble constant.
CDM is similar
to sCDM, but shifted
to larger values of the Hubble constant (as large as
65 km s-1 Mpc-1). CDM has an island of viability
around
H0 ~ 60 km s-1 Mpc-1 and
n ~ 0.95. CDM
can tolerate the largest values of the Hubble constant.
Considering other relevant data too - e.g.,
age of the Universe, determinations of
 M,
measurements of the Hubble constant, and limits to
M,
measurements of the Hubble constant, and limits to

 - CDM emerges as the
"best-fit CDM model"
(Krauss & Turner,
1995;
Ostriker & Steinhardt,
1995;
Liddle et al, 1996);
see Fig. 4.
Moreover, its "smoking gun signature,"
negative q0, has apparently been confirmed
(Riess et al, 1998;
Perlmutter et al, 1998).
Despite my general
enthusiasm, I would caution that it is premature
to conclude that CDM is anything but the model
to take aim at.
- CDM emerges as the
"best-fit CDM model"
(Krauss & Turner,
1995;
Ostriker & Steinhardt,
1995;
Liddle et al, 1996);
see Fig. 4.
Moreover, its "smoking gun signature,"
negative q0, has apparently been confirmed
(Riess et al, 1998;
Perlmutter et al, 1998).
Despite my general
enthusiasm, I would caution that it is premature
to conclude that CDM is anything but the model
to take aim at.