2. DARK MATTER SUBSTRUCTURE

Interpreting the observations

Figure 2 shows the cumulative distribution of subhalos within the high resolution Local Group halos of Figure 1. The open circles show the observed distribution for the Milky Way satellites, where I have normalised the distribution using v_peak = 210 km/s. However, baryons dominate the central region of the Galaxy and subtracting the contribution from the disk and bulge gives the maximum allowed CDM halo that has v_peak = 160 km/s (Moore etal 2001). A minimum value of v_peak = 130 km/s is required for the Galactic CDM halo to be massive enough to cool the observed mass of baryons. Figure 2 shows the effect of this correction.

Figure 1. The distribution of dark matter with a CDM "Local Group" candidate. This is a binary pair of dark matter halos at a redshift z = 0, separated by 1 Mpc and infalling at 100km/s. The large halos have virial masses of 2 × 1012 M, and with a particle mass of 106 M they are resolved with over 106 particles and 0.5 kpc force resolution. The grey scale represents the local density of dark matter - there are over 2000 dark matter satellites with circular velocity larger than 10 km/s.

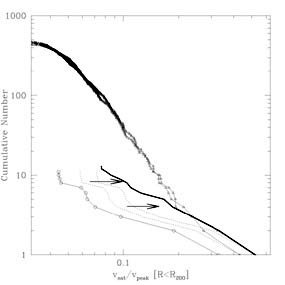Figure 2. The cumulative number of satellites within the virial radius of the Milky Way (open circles) and within the two CDM halos from Figure 1 (open triangles). Here I have taken vpeak = 210 km/s for the Milky Way, however the CDM contribution to the Milky Way can be constrained to lie in the range 130 - 160 km/s once the baryonic component has been considered (Moore etal 2001). The dotted curves show the effects of this correction. The arrows show a correction for converting central velocity dispersion to vsat. The thick solid curve shows the distribution of CDM satellites that could form stars before the universe is re-ionised.

Simon White has pointed out that the velocity dispersion of the dSph's are measured well within the cores of their dark matter halos (White 2000). We originally assumed isotropic orbits and isothermal potentials to derive v_sat from observations of the 1d central velocity dispersion (Moore etal 1999). CDM halos have central density profiles flatter than r^-2 therefore one expects the velocity dispersion to drop in the inner region. M87 provides a good example of this. This galaxy lies at the center of the Virgo cluster and has a central velocity dispersion of 350 km/s whereas the cluster has a global value that is a factor of two larger. If we assume that the dSph's are similar to M87, then the correction should scale roughly as the concentration parameter. Since c_M87 / c_dSph 0.4 - 0.5, then we expect the maximum correction to v_peak to be an increase of 50% over our quoted values. This correction is indicated by the arrows in Figure 2 which brings the observed data into good agreement with a crude model for re-ionisation discussed later.