10. HORIZONS, TEMPERATURE AND ENTROPY

One of the remarkable features of classical gravity is that it can wrap up regions of spacetime thereby producing surfaces which act as one way membranes. The classic example is that of Schwarzschild black hole of mass M which has a compact spherical surface of radius r = 2M that act as a horizon. Since the horizon can hide information - and information is deeply connected with entropy - one would expect a fundamental relationship between gravity and thermodynamics. [There is extensive literature in this subject and our citation will be representative rather than exhaustive; for a text book discussion and earlier references, see [288]; for a recent review, see [289].] As we saw in the last section, the de Sitter universe also has a horizon which suggests that de Sitter spacetime will have non trivial thermodynamic features [290].

This result can be demonstrated mathematically in many different ways of which the simplest procedure is based on the relationship between temperature and the Euclidean extension of the spacetime. To see this connection, let us recall that the mean value of some dynamical variable f (q) in quantum statistical mechanics can be expressed in the form

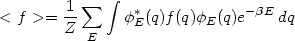(148)

where _E(q) is the stationary state eigen function of the Hamiltonian with H _E = E _E, = (1 / T) is the inverse temperature and Z() is the partition function. This expression calculates the mean value < E| f| E > in a given energy state and then averages over a Boltzmann distribution of energy states with the weightage Z^-1 exp(- E). On the other hand, the quantum mechanical kernel giving the probability amplitude for the system to go from the state q at time t = 0 to the state q' at time t is given by

(149)

Comparing (148) and (149) we find that the thermal average in (148) can be obtained by

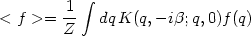(150)

in which we have done the following: (i) The time coordinate has been analytically continued to imaginary values with it = . (ii) The system is assumed to exhibit periodicity in the imaginary time with period in the sense that the state variable q has the same values at = 0 and at = . These considerations continue to hold even for a field theory with q denoting the field configuration at a given time. If the system, in particular the Greens functions describing the dynamics, are periodic with a period p in imaginary time, then one can attribute a temperature T = (1/p) to the system. It may be noted that the partition function Z() can also be expressed in the form

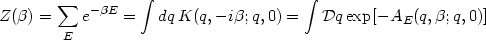(151)

The first equality is the standard definition for Z(); the second equality follows from (149) and the normalization of _E(q); the last equality arises from the standard path integral expression for the kernel in the Euclidean sector (with A_E being the Euclidean action) and imposing the periodic boundary conditions. (It is assumed that the path integral measure q includes an integration over q.) We shall have occasion to use this result later. Equations (150) and (151) represent the relation between the periodicity in Euclidean time and temperature.

Spacetimes with horizons possess a natural analytic continuation from Minkowski signature to the Euclidean signature with t = it. If the metric is periodic in , then one can associate a natural notion of a temperature to such spacetimes. For example, the de Sitter manifold with the metric (134) can be continued to imaginary time arriving at the metric

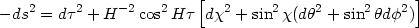(152)

which is clearly periodic in with the period (2 / H). [The original metric was a 4-hyperboloid in the 5-dimensional space while equation (152) represents a 4-sphere in the 5-dimensional space.] It follows that de Sitter spacetime has a natural notion of temperature T = (H / 2) associated with it.

It is instructive to see how this periodicity arises in the static form of the metric in (141). Consider a metric of the form

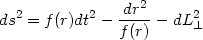(153)

where dL² denotes the transverse 2-dimensional metric and f (r) has a simple zero at r = r_H. Near r = r_H, we can expand f (r) in a Taylor series and obtain f (r) B(r - r_H) where B f'(r_H). The structure of the metric in (153) shows that there is a horizon at r = r_H. Further, since the general relativistic metric reduces to g₀₀ (1 + 2_N) in the Newtonian limit, where _N is the Newtonian gravitational potential, the quantity

(154)

can be interpreted as the gravitational attraction on the surface of the horizon - usually called the surface gravity. Using the form f (r) 2(r - r_H) near the horizon and shifting to the coordinate [2^-1(r - r_H)]^1/2 the metric near the horizon becomes

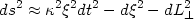(155)

The Euclidean continuation t = it now leads to the metric

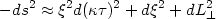(156)

which is essentially the metric in the polar coordinates in the - plane. For this metric to be well defined near the origin, should behave like an angular coordinate with periodicity 2. Therefore, we require all well defined physical quantities defined in this spacetime to have a periodicity in with the period (2 / ||). Thus, all metrics of the form in (153) with a simple zero for f (r) leads to a horizon with temperature T = || / 2 = | f'(r_H) | / 4. In the case of de Sitter spacetime, this gives T = (H / 2); for the Schwarzschild metric, the corresponding analysis gives the well known temperature T = (1 / 8 M) where M is the mass of the black-hole.