


The early (0.6  1
ms), hot, dense Universe is filled with radiation
(
1
ms), hot, dense Universe is filled with radiation
( s,
e ± pairs,
s,
e ± pairs,
 s of all flavors), along with
dynamically
and numerically insignificant amounts of baryons (nucleons) and dark
matter particles. Nuclear and weak interactions are occurring among
the neutrons, protons, e ± , and
s of all flavors), along with
dynamically
and numerically insignificant amounts of baryons (nucleons) and dark
matter particles. Nuclear and weak interactions are occurring among
the neutrons, protons, e ± , and
 s (e.g., n +
p
s (e.g., n +
p 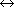 D +
D +  ;
p + e-
;
p + e-
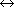 n +
n +
 e) at rates fast
compared to the universal expansion rate. At such high temperatures
(T
e) at rates fast
compared to the universal expansion rate. At such high temperatures
(T  3
MeV), in an environment where the nucleon to photon ratio is very small
(
3
MeV), in an environment where the nucleon to photon ratio is very small
( 10
10
 3 - 10), the
abundances of complex nuclei (D, 3He,4He,
7Li) are tiny in comparison to those of the
free nucleons (neutrons and protons). At the same time, the
charged-current weak interactions are regulating the neutron to proton
ratio, initially keeping it close to its equilibrium value
3 - 10), the
abundances of complex nuclei (D, 3He,4He,
7Li) are tiny in comparison to those of the
free nucleons (neutrons and protons). At the same time, the
charged-current weak interactions are regulating the neutron to proton
ratio, initially keeping it close to its equilibrium value
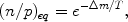 |
(13) |
where  m is
the neutron - proton mass (energy) difference.
In this context it is worth noting that if there is an asymmetry
between the numbers of
m is
the neutron - proton mass (energy) difference.
In this context it is worth noting that if there is an asymmetry
between the numbers of
 e and
e and
 e the
equilibrium
neutron-to-proton ratio is modified to (n / p)eq =
exp(-
e the
equilibrium
neutron-to-proton ratio is modified to (n / p)eq =
exp(- m /
T - µe / T) =
e-
m /
T - µe / T) =
e- e(n /
p)eq0.
e(n /
p)eq0.
As the Universe expands and cools, the lighter protons are favored
over the heavier neutrons and the neutron-to-proton ratio decreases,
tracking the equilibrium form in eq. 13. But, as the temperature
decreases below T ~ 0.8 MeV, when the Universe is ~ 1 second
old, the weak interactions are too slow to maintain equilibrium and
the neutron-to-proton ratio, while continuing to fall, deviates from
(exceeds) the equilibrium value. Since the n / p
ratio depends on the competition between the weak interaction
rates and the early-Universe expansion rate (as well as on a possible
neutrino asymmetry), deviations from the standard model (e.g.,
 R
R

 R +
R +
 X
or
X
or  e
e
 0) will change
the relative numbers of neutrons and protons available for building
the complex nuclides.
0) will change
the relative numbers of neutrons and protons available for building
the complex nuclides.
As noted above, while neutrons and protons are interconverting, they are also colliding among themselves creating complex nuclides, e.g., deuterons. However, at early times, when the density and average energy of the CBR photons are very high, the newly formed deuterons find themselves bathed in a background of high-energy gamma rays capable of photodissociating them. Since there are more than a billion CBR photons for every nucleon in the Universe, the deuteron is photodissociated before it can capture a neutron (or a proton, or another deuteron) to build the heavier nuclides. This bottleneck to BBN persists until the temperature drops sufficiently below the binding energy of the deuteron, when there are too few photons energetic enough to photodissociate them before they capture nucleons, launching BBN. This transition (smooth, but rapid) occurs after e ± annihilation, when the Universe is a few minutes old and the temperature has dropped below ~ 80 keV.
Once BBN begins in earnest, neutrons and protons quickly combine to build
D, 3H, 3He, and 4He. Since there are no
stable mass-5 nuclides, a new bottleneck appears at
4He. Nuclear reactions quickly incorporate
all available neutrons into 4He, the most strongly bound of
the light nuclides. Jumping the gap at mass-5 requires Coulomb
suppressed reactions of 4He with D, or 3H, or
3He, guaranteeing that the abundances of
the heavier nuclides are severely depressed below that of 4He
(and even of D and 3He), and that the 4He
abundance is determined by the
neutron abundance when BBN begins. The few reactions that manage to
bridge the mass-5 gap lead mainly to mass-7 (7Li or, to
7Be which, later, when the Universe has cooled further, will
capture an electron and decay to 7Li); for the range of
 B of
interest, the BBN-predicted abundance of
6Li is more than 3 orders of magnitude
below that of the more tightly bound 7Li. Finally, there is
another gap at mass-8, ensuring that there is no astrophysically
significant production of heavier nuclides.
B of
interest, the BBN-predicted abundance of
6Li is more than 3 orders of magnitude
below that of the more tightly bound 7Li. Finally, there is
another gap at mass-8, ensuring that there is no astrophysically
significant production of heavier nuclides.
The primordial nuclear reactor is short-lived. As the temperature drops
below T  30 keV, when the Universe is ~ 20 minutes old, Coulomb
barriers abruptly suppress all nuclear reactions. Afterwards, until
the first stars form, no pre-existing, primordial nuclides are destroyed
(except for those like 3H and 7Be that are
unstable and decay) and no new nuclides are created. In ~ 1000 seconds
BBN has run its course.
30 keV, when the Universe is ~ 20 minutes old, Coulomb
barriers abruptly suppress all nuclear reactions. Afterwards, until
the first stars form, no pre-existing, primordial nuclides are destroyed
(except for those like 3H and 7Be that are
unstable and decay) and no new nuclides are created. In ~ 1000 seconds
BBN has run its course.
With this as background, the trends of the SBBN-predicted primordial
abundances of the light nuclides with baryon abundance shown in
Figure 1 can be understood. The reactions
burning D and 3He (along with 3H) to
4He are very fast (compared to the universal
expansion rate) once the deuterium bottleneck is breached, ensuring
that almost all neutrons present at that time are incorporated into
4He. As a result, since 4He production is not
rate limited, its primordial abundance is very insensitive (only
logarithmically) to the baryon abundance. The very slight increase in
YP with increasing
 B
reflects the fact that for a higher baryon abundance BBN
begins slightly earlier, when slightly more neutrons are available. The
thickness of the YP curve in
Fig. 1 reflects the very
small uncertainty in the BBN prediction; the uncertainty in
YP (~ 0.2%;
B
reflects the fact that for a higher baryon abundance BBN
begins slightly earlier, when slightly more neutrons are available. The
thickness of the YP curve in
Fig. 1 reflects the very
small uncertainty in the BBN prediction; the uncertainty in
YP (~ 0.2%;
 Y ~ 0.0005)
is dominated by the very small
error in the weak interaction rates which are normalized by the neutron
lifetime (
Y ~ 0.0005)
is dominated by the very small
error in the weak interaction rates which are normalized by the neutron
lifetime ( n =
885.7 ± 0.8 s). The differences among the YP predictions
from independent BBN codes are typically no larger than
n =
885.7 ± 0.8 s). The differences among the YP predictions
from independent BBN codes are typically no larger than
 YP ~ 0.0002.
YP ~ 0.0002.
Nuclear reactions burn D, 3H, and 3He to
4He, the most tightly
bound of the light nuclides, at a rate which increases with increasing
nucleon density, accounting for the decrease in the abundances of D and
3He (the latter receives a contribution from the
 -decay of
3H) with higher values of
-decay of
3H) with higher values of
 B.
The behavior of 7Li is more interesting, reflecting two
pathways to mass-7. At the relatively low values of
B.
The behavior of 7Li is more interesting, reflecting two
pathways to mass-7. At the relatively low values of
 10
10
 3, mass-7 is
largely synthesized as 7Li by
3H(
3, mass-7 is
largely synthesized as 7Li by
3H( ,
,
 )7Li reactions. 7Li is easily
destroyed in collisions with protons. So, for low nucleon abundance, as
)7Li reactions. 7Li is easily
destroyed in collisions with protons. So, for low nucleon abundance, as
 B
increases, destruction is faster than production and 7Li/H
decreases. In contrast, at relatively high values of
B
increases, destruction is faster than production and 7Li/H
decreases. In contrast, at relatively high values of
 10
10
 3, mass-7 is
largely synthesized as 7Be via
3He(
3, mass-7 is
largely synthesized as 7Be via
3He( ,
,
 )7Be reactions.
7Be is more tightly bound than 7Li and, therefore,
harder to destroy.
As
)7Be reactions.
7Be is more tightly bound than 7Li and, therefore,
harder to destroy.
As  B
increases at high nucleon abundance, the primordial abundance of
7Be increases. Later in the evolution of the Universe,
when it is cooler and neutral atoms begin to form, 7Be
captures an electron and
B
increases at high nucleon abundance, the primordial abundance of
7Be increases. Later in the evolution of the Universe,
when it is cooler and neutral atoms begin to form, 7Be
captures an electron and
 -decays to
7Li. These two paths to mass-7 account
for the valley shape of the 7Li abundance curve in
Fig. 1.
-decays to
7Li. These two paths to mass-7 account
for the valley shape of the 7Li abundance curve in
Fig. 1.
Not shown on Figure 1 are the BBN-predicted
relic abundances of 6Li, 9Be, 10B, and
11B. Their production is suppressed by
the gap at mass-8. For the same range in
 B,
all of them lie offscale, in the range 10-20 - 10-13.
B,
all of them lie offscale, in the range 10-20 - 10-13.
For SBBN the relic abundances of the light nuclides depend on only one
free parameter, the nucleon abundance parameter
 B.
As Figure 1 reveals, for the "interesting" range
(see below) of 4
B.
As Figure 1 reveals, for the "interesting" range
(see below) of 4

 10
10
 8, the 4He mass fraction is expected to be YP
8, the 4He mass fraction is expected to be YP
 0.25, with
negligible dependence on
0.25, with
negligible dependence on
 B
while D/H and 3He/H decrease from
B
while D/H and 3He/H decrease from
 10-4 to
10-4 to
 10-5, and
7Li/H increases from
10-5, and
7Li/H increases from
 10-10 to
10-10 to  10-9. The light nuclide relic abundances span
some nine orders of magnitude, yet if SBBN is correct, one choice of
10-9. The light nuclide relic abundances span
some nine orders of magnitude, yet if SBBN is correct, one choice of
 B
(within the errors) should yield predictions consistent
with observations. Before confronting the theory with data, it is useful
to consider a few generic examples of BBN in the presence of nonstandard
physics and/or cosmology.
B
(within the errors) should yield predictions consistent
with observations. Before confronting the theory with data, it is useful
to consider a few generic examples of BBN in the presence of nonstandard
physics and/or cosmology.
The variety of modifications to the standard models of particle physics
and of cosmology is very broad, limited only by the creativity of theorists.
Many nonstandard models introduce several, new, free parameters in addition
to the baryon abundance parameter
 B.
Since there are only
four nuclides whose relic abundance is large enough to be astrophysically
interesting and, as will be explained below in more detail, only three for
which data directly relating to their primordial abundances exist at
present (D, 4He, 7Li), nonstandard models with two
or more additional parameters
may well be unconstrained by BBN. Furthermore, as discussed in the
Introduction (see Section 1.2 and
Section 1.3), there already exist two
additional parameters with claims to relevance: the expansion rate
parameter S (or,
B.
Since there are only
four nuclides whose relic abundance is large enough to be astrophysically
interesting and, as will be explained below in more detail, only three for
which data directly relating to their primordial abundances exist at
present (D, 4He, 7Li), nonstandard models with two
or more additional parameters
may well be unconstrained by BBN. Furthermore, as discussed in the
Introduction (see Section 1.2 and
Section 1.3), there already exist two
additional parameters with claims to relevance: the expansion rate
parameter S (or,
 N
N ; see eqs. 8, 11) and the
lepton asymmetry parameter L (or,
; see eqs. 8, 11) and the
lepton asymmetry parameter L (or,
 ; see eq. 12).
; see eq. 12).
The primordial abundance of 4He depends sensitively on the
pre- and the post-e ± annihilation early universe
expansion rate (the Hubble parameter H) and on the magnitude of a
 e -
e -
 e asymmetry
because each will affect the n/p ratio at BBN (see, e.g.,
Steigman, Schramm & Gunn 1977 (SSG)
[6];
for recent results see Kneller & Steigman 2004 (KS)
[9])
A faster expansion (S > 1;
e asymmetry
because each will affect the n/p ratio at BBN (see, e.g.,
Steigman, Schramm & Gunn 1977 (SSG)
[6];
for recent results see Kneller & Steigman 2004 (KS)
[9])
A faster expansion (S > 1;
 N
N > 0) leaves less time
for neutrons to convert into protons and the higher neutron abundance
results in increased production of 4He. For small changes at
fixed
> 0) leaves less time
for neutrons to convert into protons and the higher neutron abundance
results in increased production of 4He. For small changes at
fixed
 B,
B,
 YP
YP
 0.16(S - 1)
0.16(S - 1)
 0.013
0.013 N
N (KS). Although the relic abundances of D and 3He do depend
on the competition between the nuclear reaction rates and the
post-e ± annihilation expansion rate (faster
expansion
(KS). Although the relic abundances of D and 3He do depend
on the competition between the nuclear reaction rates and the
post-e ± annihilation expansion rate (faster
expansion  less D and
3He destruction
less D and
3He destruction
 more D and
3He), they are much less sensitive to
relatively small deviations from S = 1
(
more D and
3He), they are much less sensitive to
relatively small deviations from S = 1
( N
N = 0)
[9].
For mass-7 the effect of a nonstandard expansion rate is different at
low and high values of
= 0)
[9].
For mass-7 the effect of a nonstandard expansion rate is different at
low and high values of
 B. At low baryon abundance
(
B. At low baryon abundance
( 10
10
 3),
a faster expansion leaves less time for 7Li destruction and
the relic abundance of mass-7 increases. In contrast, at high baryon
abundance
(
3),
a faster expansion leaves less time for 7Li destruction and
the relic abundance of mass-7 increases. In contrast, at high baryon
abundance
( 10
10
 3), S
> 1 leaves less time for 7Be production
and the relic abundance of mass-7 decreases. As for D and
3He, the quantitative change in the 7Li abundance
is small for small deviations from SBBN.
3), S
> 1 leaves less time for 7Be production
and the relic abundance of mass-7 decreases. As for D and
3He, the quantitative change in the 7Li abundance
is small for small deviations from SBBN.
For similar reasons, YP is sensitive to an asymmetry in the
electron neutrinos which, through the charged current weak interactions,
help to regulate the n/p ratio. For
 e
> 0, there are more neutrinos
than antineutrinos, so that reactions such as n +
e
> 0, there are more neutrinos
than antineutrinos, so that reactions such as n +
 e
e
 p + e-, drive down the n/p ratio. For small
asymmetry at fixed
p + e-, drive down the n/p ratio. For small
asymmetry at fixed
 B,
KS find
B,
KS find  YP
YP
 -0.23
-0.23 e.
The primordial abundances of D, 3He, and 7Li,
while not entirely
insensitive to neutrino degeneracy, are much less affected by a nonzero
e.
The primordial abundances of D, 3He, and 7Li,
while not entirely
insensitive to neutrino degeneracy, are much less affected by a nonzero
 e
than is 4He (e.g., Kang & Steigman 1992
[10]).
e
than is 4He (e.g., Kang & Steigman 1992
[10]).
Each of these nonstandard cases (S
 1,
1,

 0) will be
considered below. While certainly not exhaustive of the nonstandard
models proposed in the literature, they actually have the potential
to provide semi-quantitative, if not quantitative, understanding of
BBN in a large class of nonstandard models. Note that data constraining
the primordial abundances of at least two different relic nuclei (one
of which should be 4He) are required to break the degeneracy
between the baryon density and the additional parameter resulting from new
physics or cosmology. 4He is a poor baryometer but a very good
chronometer and/or, leptometer; D, 3He, 7Li have
the potential to be good baryometers.
0) will be
considered below. While certainly not exhaustive of the nonstandard
models proposed in the literature, they actually have the potential
to provide semi-quantitative, if not quantitative, understanding of
BBN in a large class of nonstandard models. Note that data constraining
the primordial abundances of at least two different relic nuclei (one
of which should be 4He) are required to break the degeneracy
between the baryon density and the additional parameter resulting from new
physics or cosmology. 4He is a poor baryometer but a very good
chronometer and/or, leptometer; D, 3He, 7Li have
the potential to be good baryometers.
2.2. Simple - But Accurate - Fits To The Primordial Abundances
While BBN involves only a limited number of coupled differential equations,
they are non-linear and not easily solved analytically. As a result,
detailed comparisons of the theoretical predictions with the inferred
relic abundances of the light nuclei requires numerical calculations,
which may obscure key relations between abundances and parameters, as
well as the underlying physics. In particular, the connection between
the cosmological parameter set
{ B,
S,
B,
S,
 e} and
the abundance data set {yD, YP,
yLi} 2
may be blurred,
especially when attempting to formulate a quantitative understanding
of how the latter constrains the former. However, it is clear from
Figure 1 that the relic, light
nuclide abundances are smoothly varying, monotonic functions of
e} and
the abundance data set {yD, YP,
yLi} 2
may be blurred,
especially when attempting to formulate a quantitative understanding
of how the latter constrains the former. However, it is clear from
Figure 1 that the relic, light
nuclide abundances are smoothly varying, monotonic functions of
 B
over a limited
but substantial range. While the BBN-predicted primordial abundances
are certainly not linearly related to the baryon density (nor to
the other parameters S and
B
over a limited
but substantial range. While the BBN-predicted primordial abundances
are certainly not linearly related to the baryon density (nor to
the other parameters S and
 e),
over the restricted ranges identified above, KS
[9]
found linear fits to the predicted abundances
(or, to powers of them) which work very well indeed. Introducing them
here enables and simplifies the comparison of theory with data (below)
and permits a quick, reasonably accurate, back of the envelope,
identification of the successes of and challenges to BBN.
e),
over the restricted ranges identified above, KS
[9]
found linear fits to the predicted abundances
(or, to powers of them) which work very well indeed. Introducing them
here enables and simplifies the comparison of theory with data (below)
and permits a quick, reasonably accurate, back of the envelope,
identification of the successes of and challenges to BBN.
For the adopted range of
 B,
yD = yD(
B,
yD = yD( B)
is well fit by a power law,
B)
is well fit by a power law,
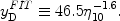 |
(14) |
While the true yD -
 B
relation is not precisely a power law, this fit (for 4
B
relation is not precisely a power law, this fit (for 4

 10
10
 8) is accurate
(compared to a numerical calculation) to better than 1%, three times
smaller than the ~ 3% BBN uncertainty estimated by Burles, Nollett, Turner
2001 (BNT)
[11];
this fit and the numerical calculation agree with
the BNT result to 2% or better over the adopted range in
8) is accurate
(compared to a numerical calculation) to better than 1%, three times
smaller than the ~ 3% BBN uncertainty estimated by Burles, Nollett, Turner
2001 (BNT)
[11];
this fit and the numerical calculation agree with
the BNT result to 2% or better over the adopted range in
 B.
Note that since different BBN codes are largely independent and often use
somewhat different nuclear reaction data sets, the differences
among their predicted abundances may provide estimates of the overall
uncertainties. It is convenient to introduce a "deuterium baryon
density parameter"
B.
Note that since different BBN codes are largely independent and often use
somewhat different nuclear reaction data sets, the differences
among their predicted abundances may provide estimates of the overall
uncertainties. It is convenient to introduce a "deuterium baryon
density parameter"
 D,
the value of
D,
the value of
 10
corresponding to an observationally determined primordial D abundance.
10
corresponding to an observationally determined primordial D abundance.
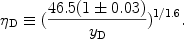 |
(15) |
Generalizing this to include the two other parameters, KS find
 |
(16) |
This fit works quite well for 2
 yD
yD
 4, corresponding
to 5
4, corresponding
to 5 
 D
D
 7. In
Figure 2 the deuterium
isoabundance curves are shown in the S -
7. In
Figure 2 the deuterium
isoabundance curves are shown in the S -
 10
plane, while Figure 3 shows the same
isoabundance contours in the
10
plane, while Figure 3 shows the same
isoabundance contours in the
 e -
e -
 10
plane. It is clear from Figures 2 and
3
that D is a sensitive baryometer since, for these ranges of
S and
10
plane. It is clear from Figures 2 and
3
that D is a sensitive baryometer since, for these ranges of
S and
 e,
e,
 D
D

 10.
10.
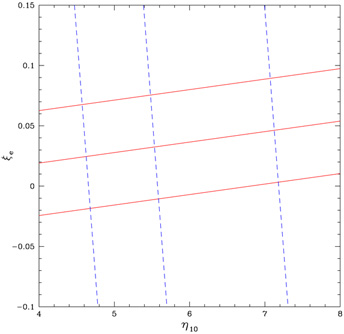 |
Figure 3. As in
Figure 2, in the neutrino asymmetry
( |
Next, consider 4He. While over a much larger range in
 10,
YP varies nearly logarthmically with the baryon
density parameter, a linear fit to the YP versus
10,
YP varies nearly logarthmically with the baryon
density parameter, a linear fit to the YP versus
 10
relation is actually
remarkably accurate over the restricted range considered here.
10
relation is actually
remarkably accurate over the restricted range considered here.
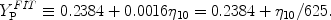 |
(17) |
Over the same range in
 10
this fit agrees with the numerical calculation and with the BNT
[11]
predictions for YP to within
0.0002 (
10
this fit agrees with the numerical calculation and with the BNT
[11]
predictions for YP to within
0.0002 ( 0.1%), or
better. Any differences between this fit
and independent, numerical calculations are smaller (much smaller)
than current estimates of the errors in the observationally inferred
primordial value of YP. The following linear fits, including
the total error estimate, to the YP - S and
YP -
0.1%), or
better. Any differences between this fit
and independent, numerical calculations are smaller (much smaller)
than current estimates of the errors in the observationally inferred
primordial value of YP. The following linear fits, including
the total error estimate, to the YP - S and
YP -
 e
relations from KS work very well over the adopted parameter ranges (see
Figures 2 & 3).
e
relations from KS work very well over the adopted parameter ranges (see
Figures 2 & 3).
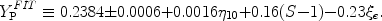 |
(18) |
As an aside, the dependence of the 4He mass fraction on the
neutron lifetime
( n) can be
included in eq. 18 by adding a term
0.0002(
n) can be
included in eq. 18 by adding a term
0.0002( n -
887.5), where
n -
887.5), where  n
is in seconds. A very recent, new measurement of
n
is in seconds. A very recent, new measurement of
 n by Serebrov
et al.
[12]
suggests that the currently accepted value
(
n by Serebrov
et al.
[12]
suggests that the currently accepted value
( n = 887.5 s)
should be
reduced by 7.2 s. If confirmed, this would lead to a slightly smaller
BBN-predicted 4He abundance:
n = 887.5 s)
should be
reduced by 7.2 s. If confirmed, this would lead to a slightly smaller
BBN-predicted 4He abundance:
 YP =
-0.0014. The
corresponding shift in the 4He inferred baryon density parameter
is negligible compared to its range of uncertainty
(
YP =
-0.0014. The
corresponding shift in the 4He inferred baryon density parameter
is negligible compared to its range of uncertainty
(
 B /
B /
 B
= -0.14), as is that for the shift in the upper bound
to N
B
= -0.14), as is that for the shift in the upper bound
to N (
( N
N max =
+0.11). These corrections are ignored here.
max =
+0.11). These corrections are ignored here.
In analogy with the deuterium baryon density parameter introduced
above, it is convenient to introduce
 He,
defined by
He,
defined by
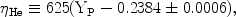 |
(19) |
so that
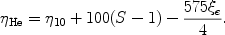 |
(20) |
For SBBN (S = 1 &
 e
= 0),
e
= 0),
 He
is the value of
He
is the value of
 10
corresponding to the adopted value of YP. Once YP
is chosen, the resulting value of
10
corresponding to the adopted value of YP. Once YP
is chosen, the resulting value of
 He
provides a linear constraint on the combination of
He
provides a linear constraint on the combination of
 10,
S, and
10,
S, and
 e
in eq. 20. This fit works well
[9]
for 0.23
e
in eq. 20. This fit works well
[9]
for 0.23  YP
YP  0.25,
corresponding to -5
0.25,
corresponding to -5 
 He
He
 7. As
Figures 2
& 3 reveal, 4He is an excellent
chronometer and/or leptometer, since the YP isoabundance
curves are nearly horizontal
(and very nearly orthogonal to the deuterium isoabundance curves).
7. As
Figures 2
& 3 reveal, 4He is an excellent
chronometer and/or leptometer, since the YP isoabundance
curves are nearly horizontal
(and very nearly orthogonal to the deuterium isoabundance curves).
As with D, the 7Li abundance
3 is well described by a power
law in
 10
over the range in baryon abundance explored here:
yLi
10
over the range in baryon abundance explored here:
yLi  1010(Li/H)
1010(Li/H) 
 102. The following KS
fit agrees with the BBN predictions to better than 3% over the adopted
range in
102. The following KS
fit agrees with the BBN predictions to better than 3% over the adopted
range in
 10,
10,
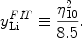 |
(21) |
While this fit predicts slightly smaller lithium abundances compared to those of BNT [11], the differences are at the 5-8% level, small compared to the BNT uncertainty estimates as well as those of Hata et al. (1995) [13] (~ 10 - 20%).
In analogy with
 D
and
D
and
 He
defined above, the lithium baryon abundance parameter
He
defined above, the lithium baryon abundance parameter
 Li
(allowing for a 10% overall uncertainty) is defined by
Li
(allowing for a 10% overall uncertainty) is defined by
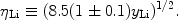 |
(22) |
The simple, linear relation for
 Li
as a function of
Li
as a function of
 10,
S,
10,
S,
 e,
which KS find fits reasonably well over the adopted parameter ranges is,
e,
which KS find fits reasonably well over the adopted parameter ranges is,
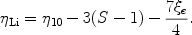 |
(23) |
This fit works well for 3
 yLi
yLi
 5, corresponding
to 5
5, corresponding
to 5 
 Li
Li
 7, but it breaks
down for yLi
7, but it breaks
down for yLi
 2
(
2
( Li
Li
 4); see
Fig. 1. As is the
case for deuterium, lithium can be an excellent baryometer since,
for the restricted ranges of S and
4); see
Fig. 1. As is the
case for deuterium, lithium can be an excellent baryometer since,
for the restricted ranges of S and
 e
under consideration here,
e
under consideration here,
 Li
Li

 10.
10.
Finally, it may be of interest to note that for 3He the power
law y3 -
 B
relation, where y3
B
relation, where y3
 105(3He/H),
which is reasonably accurate for 4
105(3He/H),
which is reasonably accurate for 4

 10
10
 8 is
8 is
 |
(24) |
The difficulty of using current observational data, limited to
chemically evolved regions of the Galaxy, to infer the primordial
abundance of 3He, along with the relatively weak dependence of
y3 on
 10,
limits the utility of this nuclide as a baryometer
[14].
3He can, however, be used as a test of BBN consistency.
10,
limits the utility of this nuclide as a baryometer
[14].
3He can, however, be used as a test of BBN consistency.
2.3. SBBN-Predicted Primordial Abundances
Before discussing the current status of the observationally determined
abundances (and their uncertainties) of the light nuclides, it is
interesting to assume SBBN and, for the one free parameter,
 B,
use the value inferred from non-BBN data such as the
CBR (WMAP) and Large Scale Structure (LSS)
[2]
to predict the relic abundances.
B,
use the value inferred from non-BBN data such as the
CBR (WMAP) and Large Scale Structure (LSS)
[2]
to predict the relic abundances.
From WMAP alone, Spergel et al. 2003
[2]
derive
 10
= 6.3 ± 0.3. Using the fits from Section 2.2,
with S = 1 and
10
= 6.3 ± 0.3. Using the fits from Section 2.2,
with S = 1 and
 e
= 1, the SBBN-predicted relic abundances are: yD =
2.45 ± 0.20;
y3 = 1.03 ± 0.04; YP = 0.2485 ±
0.0008; yLi = 4.67 ± 0.64
([Li]P = 2.67 ± 0.06).
e
= 1, the SBBN-predicted relic abundances are: yD =
2.45 ± 0.20;
y3 = 1.03 ± 0.04; YP = 0.2485 ±
0.0008; yLi = 4.67 ± 0.64
([Li]P = 2.67 ± 0.06).
When Spergel et al. 2003
[2]
combine the WMAP CBR data with
those from Large Scale Structure, they derive a consistent, but slightly
smaller (slightly more precise) baryon abundance parameter
 10
= 6.14 ± 0.25. For this choice the SBBN-predicted relic abundances
are: yD = 2.56 ± 0.18; y3 =
1.04 ± 0.04; YP = 0.2482 ±
0.0007; yLi = 4.44 ± 0.57 ([Li]P =
2.65-0.06+0.05).
10
= 6.14 ± 0.25. For this choice the SBBN-predicted relic abundances
are: yD = 2.56 ± 0.18; y3 =
1.04 ± 0.04; YP = 0.2482 ±
0.0007; yLi = 4.44 ± 0.57 ([Li]P =
2.65-0.06+0.05).
2 YP
is the 4He mass fraction while the other abundances
are measured
by number compared to hydrogen. For numerical convenience,
yD
 105(D/H) and
yLi
105(D/H) and
yLi
 1010(Li/H).
Back.
1010(Li/H).
Back.
3 It is common in the astronomical
literature to present the lithium abundance logarithmically: [Li]
 12 + log(Li/H) = 2 + log(yLi).
Back.
12 + log(Li/H) = 2 + log(yLi).
Back.