3.1 Type Ia supernovae

The most direct and theory-independent way to measure the cosmological constant would be to actually determine the value of the scale factor as a function of time. Unfortunately, the appearance of _k in formulae such as (42) renders this difficult. Nevertheless, with sufficiently precise information about the dependence of a distance measure on redshift we can disentangle the effects of spatial curvature, matter, and vacuum energy, and methods along these lines have been popular ways to try to constrain the cosmological constant.

Astronomers measure distance in terms of the ``distance modulus'' m - M, where m is the apparent magnitude of the source and M its absolute magnitude. The distance modulus is related to the luminosity distance via

(47)

Of course, it is easy to measure the apparent magnitude, but notoriously difficult to infer the absolute magnitude of a distant object. Methods to estimate the relative absolute luminosities of various kinds of objects (such as galaxies with certain characteristics) have been pursued, but most have been plagued by unknown evolutionary effects or simply large random errors [6].

Recently, significant progress has been made by using Type Ia supernovae as ``standardizable candles''. Supernovae are rare - perhaps a few per century in a Milky-Way-sized galaxy - but modern telescopes allow observers to probe very deeply into small regions of the sky, covering a very large number of galaxies in a single observing run. Supernovae are also bright, and Type Ia's in particular all seem to be of nearly uniform intrinsic luminosity (absolute magnitude M ~ -19.5, typically comparable to the brightness of the entire host galaxy in which they appear) [36]. They can therefore be detected at high redshifts (z ~ 1), allowing in principle a good handle on cosmological effects [37, 38].

The fact that all SNe Ia are of similar intrinsic luminosities fits well with our understanding of these events as explosions which occur when a white dwarf, onto which mass is gradually accreting from a companion star, crosses the Chandrasekhar limit and explodes. (It should be noted that our understanding of supernova explosions is in a state of development, and theoretical models are not yet able to accurately reproduce all of the important features of the observed events. See [39, 40, 41] for some recent work.) The Chandrasekhar limit is a nearly-universal quantity, so it is not a surprise that the resulting explosions are of nearly-constant luminosity. However, there is still a scatter of approximately 40% in the peak brightness observed in nearby supernovae, which can presumably be traced to differences in the composition of the white dwarf atmospheres. Even if we could collect enough data that statistical errors could be reduced to a minimum, the existence of such an uncertainty would cast doubt on any attempts to study cosmology using SNe Ia as standard candles.

Fortunately, the observed differences in peak luminosities of SNe Ia are very closely correlated with observed differences in the shapes of their light curves: dimmer SNe decline more rapidly after maximum brightness, while brighter SNe decline more slowly [42, 43, 44]. There is thus a one-parameter family of events, and measuring the behavior of the light curve along with the apparent luminosity allows us to largely correct for the intrinsic differences in brightness, reducing the scatter from 40% to less than 15% - sufficient precision to distinguish between cosmological models. (It seems likely that the single parameter can be traced to the amount of ⁵⁶Ni produced in the supernova explosion; more nickel implies both a higher peak luminosity and a higher temperature and thus opacity, leading to a slower decline. It would be an exaggeration, however, to claim that this behavior is well-understood theoretically.)

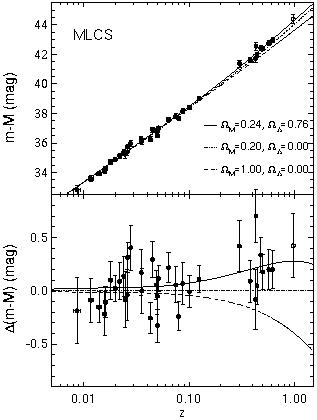
Figure 3. Hubble diagram (distance modulus vs. redshift) from the High-Z Supernova Team [48]. The lines represent predictions from the cosmological models with the specified parameters. The lower plot indicates the difference between observed distance modulus and that predicted in an open-universe model.

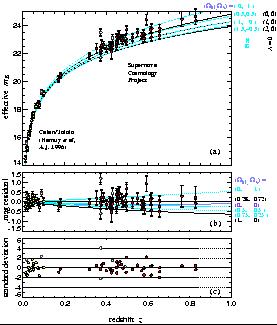
Figure 4. Hubble diagram from the Supernova Cosmology Project [52]. The bottom plot shows the number of standard deviations of each point from the best-fit curve.

Following pioneering work reported in [45], two independent groups have undertaken searches for distant supernovae in order to measure cosmological parameters. Figure (3) shows the results for m - M vs. z for the High-Z Supernova Team [46, 47, 48, 49] and Figure (4) shows the equivalent results for the Supernova Cosmology Project [50, 51, 52]. Under the assumption that the energy density of the universe is dominated by matter and vacuum components, these data can be converted into limits on _M and , as shown in Figures (5) and (6).

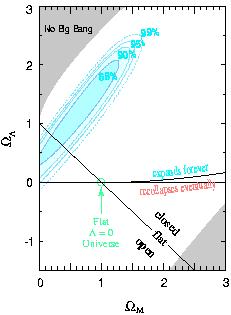
Figure 5. Constraints in the M - plane from the High-Z Supernova Team [48].

Figure 6. Constraints in the M - plane from the Supernova Cosmology Project [52].

Given the significance of these results, it is natural to ask what level of confidence we should have in them. There are a number of potential sources of systematic error which have been considered by the two teams; see the original papers [47, 48, 52] for a thorough discussion. The two most worrisome possibilities are intrinsic differences between Type Ia supernovae at high and low redshifts [53, 54] possible extinction via intergalactic dust [55, 56, 57, 58, 59]. (There is also the fact that intervening weak lensing can change the distance-magnitude relation, but this seems to be a small effect in realistic universes [60, 61]. Both effects have been carefully considered, and are thought to be unimportant, although a better understanding will be necessary to draw firm conclusions. Here, I will briefly mention some of the relevant issues.

As thermonuclear explosions of white dwarfs, Type Ia supernovae can occur in a wide variety of environments. Consequently, a simple argument against evolution is that the high-redshift environments, while chronologically younger, should be a subset of all possible low-redshift environments, which include regions that are ``young'' in terms of chemical and stellar evolution. Nevertheless, even a small amount of evolution could ruin our ability to reliably constrain cosmological parameters [53]. In their original papers [47, 48, 52], the supernova teams found impressive consistency in the spectral and photometric properties of Type Ia supernovae over a variety of redshifts and environments (e.g., in elliptical vs. spiral galaxies). More recently, however, Riess et al. [54] have presented tentative evidence for a systematic difference in the properties of high- and low-redshift supernovae, claiming that the risetimes (from initial explosion to maximum brightness) were higher in the high-redshift events. Apart from the issue of whether the existing data support this finding, it is not immediately clear whether such a difference is relevant to the distance determinations: first, because the risetime is not used in determining the absolute luminosity at peak brightness, and second, because a process which only affects the very early stages of the light curve is most plausibly traced to differences in the outer layers of the progenitor, which may have a negligible affect on the total energy output. Nevertheless, any indication of evolution could bring into question the fundamental assumptions behind the entire program. It is therefore essential to improve the quality of both the data and the theories so that these issues may be decisively settled.

Other than evolution, obscuration by dust is the leading concern about the reliability of the supernova results. Ordinary astrophysical dust does not obscure equally at all wavelengths, but scatters blue light preferentially, leading to the well-known phenomenon of ``reddening''. Spectral measurements by the two supernova teams reveal a negligible amount of reddening, implying that any hypothetical dust must be a novel ``grey'' variety. This possibility has been investigated by a number of authors [55, 56, 57, 58, 59]. These studies have found that even grey dust is highly constrained by observations: first, it is likely to be intergalactic rather than within galaxies, or it would lead to additional dispersion in the magnitudes of the supernovae; and second, intergalactic dust would absorb ultraviolet/optical radiation and re-emit it at far infrared wavelengths, leading to stringent constraints from observations of the cosmological far-infrared background. Thus, while the possibility of obscuration has not been entirely eliminated, it requires a novel kind of dust which is already highly constrained (and may be convincingly ruled out by further observations).

According to the best of our current understanding, then, the supernova results indicating an accelerating universe seem likely to be trustworthy. Needless to say, however, the possibility of a heretofore neglected systematic effect looms menacingly over these studies. Future experiments, including a proposed satellite dedicated to supernova cosmology [62], will both help us improve our understanding of the physics of supernovae and allow a determination of the distance/redshift relation to sufficient precision to distinguish between the effects of a cosmological constant and those of more mundane astrophysical phenomena. In the meantime, it is important to obtain independent corroboration using other methods.