Essay

BLACK BODY

Adapted from P. Coles, 1999, The Routledge Critical Dictionary of the New Cosmology, Routledge Inc., New York. Reprinted with the author's permission. To order this book click here: http://www.routledge-ny.com/books.cfm?isbn=0415923549

If a body emits electromagnetic radiation in a state of thermal equilibrium, then that radiation is described as black-body radiation and the object is said to be a black body. Of course, this does not mean that the body is actually black - the Sun, for example, radiates light approximately as a black body. The word `black' here simply indicates that radiation can be perfectly absorbed and re-radiated by the object. The spectrum of light radiated by such an idealised black body is described by a universal spectrum called the Planck spectrum, which is described below. The precise form of the spectrum depends on the absolute temperature T of the radiation.

The cosmic microwave background radiation has an observed spectrum which is completely indistinguishable from the theoretical black-body shape described by the Planck spectrum. Indeed, the spectrum of this relic radiation is a much closer to that of a black body than any that has yet been Figure overleaf). This serves to prove beyond reasonable doubt that this radiation has its origins in a stage of the thermal history of the Universe during which thermal equilibrium was maintained. This, in turn, means that matter must have been sufficiently hot for scattering processes to have maintained thermal contact between the matter and the radiation in the Universe. In other words, it shows that the Big Bang must have been hot. The spectrum and isotropy of the cosmic microwave background radiation are two essential pieces of evidence supporting the standard version of the Big Bang theory, based on the Friedmann models.

In thermodynamics, we can associate the temperature T with a fundamental quantity of energy E = kT, where k is a fundamental physical constant known as the Boltzmann constant. In thermal equilibrium, the principle of equipartition of energy applies: each equivalent way in which energy can be stored in a system is allocated the same amount, which turns out to be one-half of this fundamental amount. This principle can be applied to all sorts of systems, so calculating the properties of matter in thermal equilibrium is quite straight-forward. This fundamental property of objects in thermal equilibrium has been known since the 19th century, but it was not fully applied to the properties of thermal black-body radiation until the early years of the 20th century. The reason for this delay was that applying the thermodynamic rules to the problem of black-body radiation was found to give non-sensical results. In particular, if the principle of equipartition is applied to the different possible wavelengths of electromagnetic radiation, we find that a black body should radiate an infinite amount of energy at infinitely short wavelengths. This disaster became known as the ultraviolet catastrophe.

The avoidance of the ultraviolet catastrophe was one of the first great achievements of quantum physics. Max Planck stumbled upon the idea of representing the thermal behaviour of the black body in terms of oscillators, each vibrating at a well-defined frequency. He could then apply thermodynamical arguments to this set of oscillators and get a sensible (non-divergent) answer for the shape of the spectrum emitted. Only later, however, did Albert Einstein put forward the idea that is now thought to be the real reason for the black-body spectrum: that the radiation field itself is composed of small packets called quanta, each with discrete energy levels. The quanta of electromagnetic radiation are now universally known as photons (see elementary particles). The energy E of each photon is related to its frequency by the fundamental relation E = h, where h is the Planck constant. Note that this constant has the same dimensions (energy) as kT: the ratio h / kT is therefore a dimensionless number. With the aid of the quantum theory of light, we can obtain the form of the Planck spectrum:

B(T) = (2h / c²) [exp(h / kT) - 1]^-1

where c is the speed of light. As one might have expected, this curve has a maximum at h approx kT, so that hotter bodies radiate most of their energy at higher frequencies. Note, however, that the exponential function guarantees that the function B(T) does not diverge at high values of h / kT. At low frequencies (h / kT >> 1) this function reproduces the Rayleigh-Jeans law, which states that B(T) = 2² kT / c², and at high frequencies one finds that B(T) falls off exponentially according to Wien's law:

B(T) = (2h³ / c²) exp(-h / kT)

This latter behaviour is responsible for avoiding the ultraviolet catastrophe.

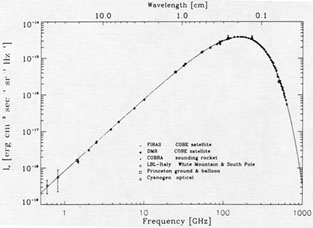
Black body. A compilation of experimental measurements of the spectrum of the cosmic microwave background radiation reveals an accurate black-body spectrum.

Everything gives off photons in this way, and the higher the temperature of an object, the more energetic the emitted photons are. Hot furnaces with black walls glow red; hotter furnaces glow brighter and whiter. Furnaces emit a black-body spectrum determined by their temperature, of around 600 K. The Sun is ten times hotter, and emits a nearly black-body spectrum at 6000 K. The last scattering surface emitted like a black body at about 3000 K, and now, when the Universe is a thousand times larger, the Universe is pervaded by photons that have maintained their black-body spectrum but have undergone a redshift to about 3 K(i.e. 3000K/1000) because of the expansion of the Universe.

Measurements of the spectrum of the cosmic microwave background (CMB) radiation indicate that it matches the Planck spectrum perfectly, to an accuracy better than one part in ten thousand, with a temperature T of around 2.73 degrees Kelvin. The accuracy to which this temperature is known allows us to determine very accurately exactly how many photons there are in the Universe and therefore work out the details of the thermal history of the Universe.

Although no deviations from a black-body spectrum have yet been detected, we do not expect the CMB to have an exact black-body spectrum. Features in the spectrum such as emission lines and absorption lines, an infrared excess (see infrared astronomy) or any of the distortions mentioned below would tell us about the details of the sources responsible for them.

The process by which a system reaches thermal equilibrium is called thermalisation. Thermalisation of both the photon energy and photon number are required to erase a distortion and make the spectrum black-body. But thermalisation requires scattering between the matter and radiation. As the Universe cools and rarefies, thermalisation (or equipartition of energy) becomes more difficult. Up to 1 year after the Big Bang, both photon energy and photon number were thermalised because scattering was very efficient. This is unfortunate, because it means that all remnants of events in the early Universe have been lost. Between 1 year and 100 years after the Big Bang, photon energy but not photon number were thermalised. Later than 100 years after the Big Bang neither photon energy nor photon number could be thermalised. Any distortions in the spectrum during this epoch should be observable.

There are many possible effects which can cause the CMB spectrum to deviate from a true black-body spectrum. In general, any photonÄmatter interaction which has a frequency dependence will distort the spectrum. When hot electrons in the intergalactic medium heat up the CMB photons, they introduce a distortion called the Sunyaev-Zel'dovich effect, sometime also known as the y-distortion. Electrons scattering off ions produce radiation known as bremsstrahlung, `braking radiation', which can distort the CMB spectrum. Dust emission produces an infrared excess in the spectrum. Recombination itself produces a Lyman-alpha emission line. The temperature anisotropies also produce distortions when the spectrum is obtained over different regions of the sky, thus adding together spots of different temperature, but this effect is at a low level. The lack of any distortions places strong limits on any non-thermal processes that might have injected energy into the radiation field at early times. For example, it has been speculated that a so-called µ-distortion might have been produced by non-thermal processes, perhaps associated with Silk damping or via the decay of some form of elementary particle. Under these circumstances the exponential term in the Planck law becomes modified to (h + µ) / kT. But the limits from spectral measurements on the size of m are exceedingly strong: it has to have an effect of less than 0.01% on the shape of the spectrum, thus effectively ruling out such models.

Despite the many physically possible sources of distortions, none has so far been found in the averaged CMB spectrum. As more precise measurements of the CMB spectrum come to be made, spectral distortions are expected to be found at some level. New instruments which are currently being developed, such as the DIMES satellite, may detect distortions at low frequencies. Their results would tell us much about the thermal history of the Universe. Measurements of the Sunyaev-Zel'dovich (SZ) effect in the directions of galaxy clusters are giving us information about the hot gases in clusters, cluster size and an independent determination of the Hubble constant. SZ measurements in the directions of distant clusters also independently confirm that the CMB is not local in origin.