4.2. Quark Masses and the Stability of the Proton and Deuteron

It has long been noted that the stability of the proton depends on the up and down quark masses, requiring m_d - m_u E_em ^3/2 m_proton to overcome the extra electromagnetic mass-energy E_em of a proton relative to a neutron. Detailed considerations suggest that m_d - m_u is quite finely tuned, in the sense that if it were changed by more than a fraction of its value either way, nuclear astrophysics as we know it would radically change.

Quarks being always confined never appear ``on-shell'' so their masses are tricky to measure precisely. A recent review by Fusaoka and Koide (1998) gives m_u = 4.88 ± 0.57 MeV, m_d = 9.81 ± 0.65 MeV, larger than the 0.511 MeV of the electron but negligible compared to the 938.272 MeV mass of the proton, 939.566 MeV of the neutron, or 1875.613 MeV of the deuteron. On the other hand small changes in m_d-m_u can have surprisingly profound effects on the world through their effect on the relative masses of the proton, neutron and deuteron. If m_n < m_p the proton is unstable and there are no atoms, no chemistry. It is thus important that m_n > m_p, but not by too much since the neutron becomes too unstable. The neutron - decay rate is as small as it is only because of the small n, p mass difference: it is closely controlled by the phase space suppression. With a small increase in the mass difference the neutron decays much faster and the deuteron becomes unstable, also leading to radical changes in the world.

Consider for example the pp reaction,

(14)

which begins the conversion of hydrogen to helium in the Sun. The endpoint of this reaction is only 420 keV, meaning that if the deuteron were 420 keV heavier (relative to the other reactants) the reaction would not even be exothermic and would tend to run in the other direction.

Although the quark masses are uncertain, we can estimate the effect a change in their difference would have. To the extent that the neutron and proton structures preserve isospin symmetry, the calculation is simple since their masses just change additively in response to a change in the quark masses. For the deuteron the story is a little more involved because of the effect on the nuclear potential.

Consider a transformation to a different world with different values of the quark and electron masses,

(15)

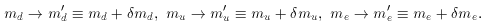
We then have

(16)

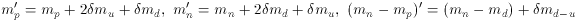
We have defined a key parameter, the amount of change in the mass difference, m_d-u m_d - m_u.

Now consider the effect of this transformation on the reactions

(17)

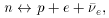
The heat balance of these reactions in our world is

(18)

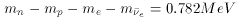
In the transformed world, a hydrogen atom (HI) is unstable (through the proton capturing the electron and converting into a stable neutron) if

(19)

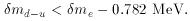
In atoms, or in plasmas where electrons are readily available, the neutron becomes the energetically favored state. As m_d-u drops, Big Bang nucleosynthesis first increases the helium abundance to near 1, then makes most of the baryons into neutrons. There would be no hydrogen atoms except a small residue of deuterium. Synthesis of heavy elements could still continue (although as shown below, with the nuclei somewhat altered). Indeed there is no Coulomb barrier to keep the neutrons apart and hardly any electrons to provide opacity, so the familiar equilibrium state of main-sequence stars would disappear. The effects get even more radical as m_d-u decreases even more; rapid, spontaneous decay of a free proton to a neutron happens if

(20)

For positive m_d-u, we have the opposite problem; neutrons and deuterons are destabilized. First, we restrict ourselves to constant m_d+u m_d + m_u = 0, so changes in nuclear potential can be neglected. Then we consider just the effect of the change in deuteron mass,

(21)

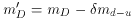
on the pp reactions p + p -> D + e⁺ + _e. In our world the heat balance is

(22)

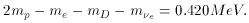
The pp -> D direction stops being energetically favored if

(23)

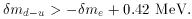
In the Big Bang plasma, the abundance of deuterons in this world is highly suppressed, so there is no stepping-stone to the production of helium and heavier nuclei, so the universe initially is made of essentially pure protons. ⁽⁹⁾ Furthermore, since the pp chain is broken, cosmic chemical history would be radically altered: For example, there is no two-body reaction for nucleosynthesis in stars to get started so main-sequence stars would all have to use catalytic cycles such as the CNO process (where the heavy catalysts would have to be generated in an early generation under degenerate conditions).

As long as stable states of heavier nuclei exist, some of them would likely be produced occasionally in degenerate deflagrations (akin to Type Ia supernovae). As m_d-u increases, the valley of -stability moves to favor fewer neutrons; a free deuteron spontaneously fissions into two protons if

(24)

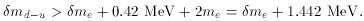
Above some threshold, stable states of heavier nuclei disappear altogether and there is no nuclear physics at all.

Thresholds for these effects are shown in figure 1. Note that m_d-m_u is bounded within a small interval - if it departs from this range one way or another a major change in nuclear astrophysics results. The total width of the interval, of the order of an MeV, depending on how drastic the changes are, should be compared with the values m_u 5 MeV and m_d 10 MeV, or the mass of the proton, 1 GeV.

Figure 1. Effects of changes in the light quark mass difference and electron mass on the stability of the proton and deuteron. Our world sits at the origin; outside the bold lines nuclear astrophysics changes qualitatively in four ways described in the text. The physical effects are: destabilization of an isolated deuteron; destabilization of a proton in the presence of an electron; pp reaction goes the wrong way; destabilization of an isolated proton. Thresholds are shown for the four effects - solid lines from equations 19 and 21, dashed lines from 23 and 24, the latter assuming md + mu = 0. Dotted lines show a constraint (appropriate in an SO(10) GUT) imposed by positive up-quark mass for fixed me / md, and we plot only the region of positive electron mass. The change in the sum md+u (the combination not shown here) is similarly constrained within less than 0.05 MeV of its actual value so as not to drastically alter carbon-producing reactions.

We should consider these constraints with the kind of additional joint constraints that unification symmetry is likely to impose on the fermion masses. For example, suppose that some symmetry fixes the ratio m_d / m_e (e.g., Fukugita et al. 1999), thereby fixing m_d / m_e, and we require that m_u > 0. The resulting constraint is illustrated in figure 1.

⁹ The reactions are of course also affected by couplings which enter into reaction rates. The balance between the expansion rate and weak interaction rates controls nucleosynthesis both in supernovae and in the Big Bang. For example, Carr and Rees (1979) argue that avoiding a universe of nearly pure helium requires the weak freeze-out to occur at or below the temperature equal to the n, p mass difference, requiring (m_n - m_p)³ > m_Planck^{-1 -2} m_proton^-2 m_W⁴. Back.