


5.2. Equations of motion and solutions
The equations for an expanding Universe containing a homogeneous scalar field are easily obtained by substituting Eqs. (33) and (34) into the Friedmann and fluid equations, giving
where prime indicates d /
d
Since
we will have inflation whenever the potential energy dominates. This should
be possible provided the potential is flat enough, as the scalar field would
then be expected to roll slowly. The potential should also have a minimum in
which inflation can end.
The standard strategy for solving these equations is the slow-roll
approximation (SRA); this assumes that a term can be neglected in each of
the equations of motion to leave the simpler set
If we define slow-roll parameters
[3]
where the first measures the slope of the potential and the second the
curvature, then necessary conditions for the slow-roll approximation to hold
are (4)
Unfortunately, although these are necessary conditions for the slow-roll
approximation to hold, they are not sufficient, since even if the
potential is very flat it may be that the scalar field has a large
velocity. A more elaborate version of the SRA exists, based on the
Hamilton-Jacobi formulation of inflation,
[4] which is sufficient
as well as necessary.
[5]
Note also that the SRA reduces the order of the system of equations by
one, and so its general solution contains one less initial condition.
It works only because one can prove
[4,
5]
that the solution to
the full equations possesses an attractor property, eliminating the
dependence on the extra parameter.
4 Note that
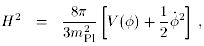
(38)
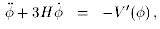
(39)
 . Here I have ignored the
curvature term
k, since we know that by definition it will quickly become negligible
once inflation starts. This is done for simplicity only; there is no
obstacle to including that term.
. Here I have ignored the
curvature term
k, since we know that by definition it will quickly become negligible
once inflation starts. This is done for simplicity only; there is no
obstacle to including that term.

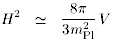
(41)
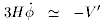
(42)
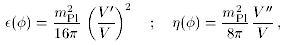
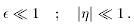
 is positive by definition,
whilst
is positive by definition,
whilst  can have
either sign.
Back.
can have
either sign.
Back.