


8.2. Angular momentum and dissipative collapse
In Section 6.3 we showed that the tidal torque theory for the origin of angular momentum is inconsistent with the idea that spiral discs formed by the dissipative collapse of a self-gravitating gas cloud. The White and Rees theory offers an interesting way out of this difficulty (Efstathiou and Jones, 1980; Fall and Efstathiou, 1980).
Although some aspects of the theory are inevitably rather vague, one that appears to be on fairly safe ground is the expectation that the total angular momentum per unit mass of the gas destined to form a disc should be equal to that of the dark halo material:
 |
(8.9) |
This is because all the material in the system experiences the same
external torques and violent relaxation before separating into two
distinct components. The numerical simulations discussed in Section
(6.3) predict that a typical system will have a pre-collapse value of
the dimensionless spin parameter of
 = 0.07. The goal is
now to
compute the factor by which the protocloud must collapse in order to
produce a centrifugally supported disc. We assume that the equilibrium
disc has an exponential surface density profile,
= 0.07. The goal is
now to
compute the factor by which the protocloud must collapse in order to
produce a centrifugally supported disc. We assume that the equilibrium
disc has an exponential surface density profile,
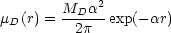 |
(8.10) |
and a flat-rotation curve with amplitude vm which we parametrize by the dimensionless ratio,
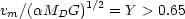 |
(8.11) |
where the constraint Y > 0.65 is required so that the halo density be everywhere positive. Thus the disc's angular momentum is,
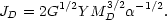 |
(8.12) |
We now assume that disc self-gravity may be neglected. Thus, the halo density profile must be of the form,
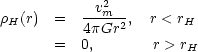 |
(8.13) |
in order to produce a flat rotation curve. Equations (8.11) and (8.13) thus give,
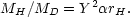 |
(8.14) |
Now,
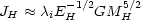 |
where  i is
the pre-collapse spin-parameter of the system and
EH is
the total energy of the halo component. Since the halo component
collapses without dissipation we may compute EH from
(8.13) and since angular momentum is conserved, the collapse factor of
the disc component,
i is
the pre-collapse spin-parameter of the system and
EH is
the total energy of the halo component. Since the halo component
collapses without dissipation we may compute EH from
(8.13) and since angular momentum is conserved, the collapse factor of
the disc component,
 rH,
may be fixed from Eqs. (8.9) and (8.12). This gives,
rH,
may be fixed from Eqs. (8.9) and (8.12). This gives,
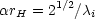 |
(8.15) |
and is independent of the parameter Y. Thus, if
 i = 0.07 we
find
i = 0.07 we
find  rH
rH
 20, which is an
order of magnitude smaller than the prediction of Eq.
(6.26) and is compatible with the requirement that the collapse time
be considerably less than the age of the Universe and with
observational arguments which suggest that the disc collapsed by a
factor of ~ 10. A detailed computation, using a more realistic
rotation curve and which does not neglect the self-gravity of the
disc, is given by
Fall and Efstathiou
(1980).
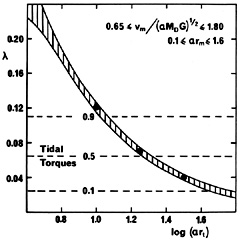
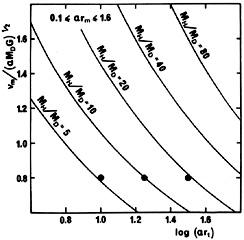
Their results are
summarized in Figures 8.2(a, b). Notice that
their results are well fitted by Eq. (8.15) for
20, which is an
order of magnitude smaller than the prediction of Eq.
(6.26) and is compatible with the requirement that the collapse time
be considerably less than the age of the Universe and with
observational arguments which suggest that the disc collapsed by a
factor of ~ 10. A detailed computation, using a more realistic
rotation curve and which does not neglect the self-gravity of the
disc, is given by
Fall and Efstathiou
(1980).
Their results are
summarized in Figures 8.2(a, b). Notice that
their results are well fitted by Eq. (8.15) for
 rH
rH
 10.
10.
The fraction of the total mass of a galaxy in the halo component is
given by Eq. (8.14). However, this depends on the parameter Y which
cannot be directly determined from observations of disc galaxies.
Theoretical arguments concerning the stability of disc galaxies
suggest that Y ~ 1
(Efstathiou, Lake and
Negroponte, 1982)
in which case MH / MD

 rH
rH
 20 if
20 if
 i = 0.07.
i = 0.07.
The available data are thus consistent with the general picture in
which dark material and gas collapsed simultaneously to produce
disc-halo systems. The collapse factors and mass factors predicted by
White and Rees agree remarkably well with those necessary to explain
the rotation of disc galaxies by tidal torques. However, the slow
rotation observed in giant elliptical galaxies does not have such a
natural explanation within the framework of the White-Rees theory. The
mass-to-luminosity ratio within a de Vaucouleurs' effective radius,
re, is observed to be
M/L  14h(M/L)
14h(M/L) for typical giant ellipticals
(Section 2.1D). This is far less than the
M/L values of rich clusters
but comparable to the M/L values within the
Holmberg radii of spiral
discs. In order to explain these observations, one infers that giant
ellipticals must have collapsed by a factor of ~ 10, similar to the
collapse factor required for the formation of a disc galaxy. It is,
therefore, difficult to understand why giant ellipticals are observed
to rotate so slowly, with
for typical giant ellipticals
(Section 2.1D). This is far less than the
M/L values of rich clusters
but comparable to the M/L values within the
Holmberg radii of spiral
discs. In order to explain these observations, one infers that giant
ellipticals must have collapsed by a factor of ~ 10, similar to the
collapse factor required for the formation of a disc galaxy. It is,
therefore, difficult to understand why giant ellipticals are observed
to rotate so slowly, with
 ~ 0.07, similar to a
typical pre-collapse value of the spin parameter from tidal-torques.
~ 0.07, similar to a
typical pre-collapse value of the spin parameter from tidal-torques.
 |
|
 |
|
Figure 8.2. (a) Pre-collapse spin parameter
as a function of collapse factor
where
(b) Halo-disc mass ratio as a function of collapse factor
|
One possibility
(Kashlinsky, 1982)
utilizes the broad distribution
of the  -parameter from
tidal torques (cf. Figures 8.2a) to argue that
ellipticals form from proto-clouds which collapse in halos with
unusually low pre-collapse
-parameter from
tidal torques (cf. Figures 8.2a) to argue that
ellipticals form from proto-clouds which collapse in halos with
unusually low pre-collapse
 values. Another
possibility
(Hogan, 1979)
is that a pregalactic generation of massive stars that formed soon
after recombination may have photoionized the remaining gas. The
ionized gas will remain coupled to the background radiation by Compton
drag whilst the stellar component (ultimately to become the dark
material) can continue to cluster hierarchically. The gas can only
collapse when the sources expire, or when Compton drag becomes
ineffective at
zc
values. Another
possibility
(Hogan, 1979)
is that a pregalactic generation of massive stars that formed soon
after recombination may have photoionized the remaining gas. The
ionized gas will remain coupled to the background radiation by Compton
drag whilst the stellar component (ultimately to become the dark
material) can continue to cluster hierarchically. The gas can only
collapse when the sources expire, or when Compton drag becomes
ineffective at
zc  140(
140( h2)1/5, whichever happens
first. Because the
gas remains locked to the comoving frame whilst it is ionized, tidal
torques will not operate on the gas. Thus when Compton drag ceases,
the gas will have a lower specific angular momentum than the dark
material (violating the assumption of Eq. 8.9) and may be able to
dissipate a large fraction of its binding energy yet still produce a
slowly rotating elliptical.
h2)1/5, whichever happens
first. Because the
gas remains locked to the comoving frame whilst it is ionized, tidal
torques will not operate on the gas. Thus when Compton drag ceases,
the gas will have a lower specific angular momentum than the dark
material (violating the assumption of Eq. 8.9) and may be able to
dissipate a large fraction of its binding energy yet still produce a
slowly rotating elliptical.
A major difficulty with either of these suggestions lies in accounting for the observation that ellipticals occur predominantly in rich clusters of galaxies. In Kashlinsky's picture it is difficult to see how the spin-parameters of proto-galaxies could be correlated on the scales of galaxy clusters at the time of galaxy formation. Whilst in Hogan's picture, Compton drag becomes ineffective at a relatively high redshift, when a rich cluster was still a small density perturbation.
Several authors (Fall, 1979b; Efstathiou and Jones, 1980; Silk and Norman, 1981) have pointed out that galaxy mergers might resolve the problem of forming ellipticals in the White-Rees theory. As we have seen above, there seems to be little difficulty (at this level!) in accounting for spiral discs. Thus we can argue that all proto-clouds collapse and form disc galaxies. Ellipticals then form from mergers between disc systems. In this way, we might hope to account for the correlation between morphological type and environment, the fraction of elliptical galaxies relative to spirals and the rotational properties of ellipticals. The extent to which the merger scheme can account for these facts has been reviewed in detail in Section 7, so we will not repeat the discussion here. Suffice it to say that a good deal more work needs to be done before we have a final answer.