


5.5.1. Isothermal distributions
The simplest distribution of gas temperatures would be an isothermal
distribution, with Tg being constant. The intracluster
gas would become
isothermal if thermal conduction were sufficiently rapid (see equation
5.41 for the relevant time scale). Alternatively, the gas may have been
introduced into the
cluster with an approximately constant temperature, and its thermal
distribution be unchanged since that time.
Lea et al. (1973)
fit the gas
distributions in the Coma, Perseus, and M87/Virgo clusters, assuming
that the gas distribution were self-gravitating and isothermal. If
 (r) is due only to
(r) is due only to
 g,
then equation (5.56) and Poisson's equation for the
gravitational potential are equivalent to equation (2.9), the equation of an
isothermal sphere. Note, however, that this is not trivially true, since
equation
(2.9) was derived from the stellar dynamical equation for a collisionless
gas, while equation (5.56) is the hydrostatic equation for a collisionally
dominated fluid. Lea et al. therefore used equation (5.57) to fit
the gas density
g,
then equation (5.56) and Poisson's equation for the
gravitational potential are equivalent to equation (2.9), the equation of an
isothermal sphere. Note, however, that this is not trivially true, since
equation
(2.9) was derived from the stellar dynamical equation for a collisionless
gas, while equation (5.56) is the hydrostatic equation for a collisionally
dominated fluid. Lea et al. therefore used equation (5.57) to fit
the gas density
 g.
This is not consistent, since the gas masses derived from these
fits were generally less than 20% of the virial mass of the cluster, and
the core radii derived were larger than those of the galaxies
(Section 4.4).
g.
This is not consistent, since the gas masses derived from these
fits were generally less than 20% of the virial mass of the cluster, and
the core radii derived were larger than those of the galaxies
(Section 4.4).
More consistent isothermal models can be derived if the gravitational potential of the cluster is not assumed to come only from the gas (Cavaliere and Fusco-Femiano, 1976, 1978; Sarazin and Bahcall, 1977). Equation (5.56) can be written

| (5.62) |
If the potential is given by equations (5.59) and (5.61), then the gas distribution is given by
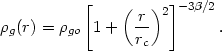
| (5.63) |
Here
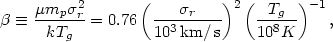
| (5.64) |
where the numerical value follows if solar abundances are assumed so that
µ = 0.63. Another way to derive equation (5.63) is to note
that if the galaxy velocity dispersion is isotropic, the galaxy density
 gal
in a cluster will be given
by equation (5.62), replacing kTg /
µmp with
gal
in a cluster will be given
by equation (5.62), replacing kTg /
µmp with
 r2.
Then eliminating the potential between the equations for
r2.
Then eliminating the potential between the equations for
 g
and
g
and
 gal
yields
gal
yields
 g
g

 gal
gal , and equation (5.63)
follows if the galaxy distribution is given by equation (5.57)
(Cavaliere and
Fusco-Femiano, 1976).
, and equation (5.63)
follows if the galaxy distribution is given by equation (5.57)
(Cavaliere and
Fusco-Femiano, 1976).
This self-consistent isothermal model (equation 5.63) assumes that the gas and galaxy distributions are both static and isothermal and that the galaxy and total mass distributions are identical. While none of these assumptions is fully justified, and the gas is probably not generally isothermal, this model has the advantage that the resulting gas distribution is analytic and that basically all the integrals needed to compare the model to the observations of clusters are also analytic. For example, the total gas mass and emission integral (equation 5.20) are
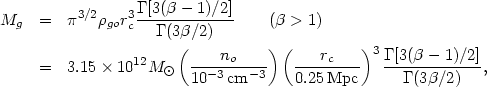
| (5.65) |

| (5.66) |
where n0 is the central proton density,
 is the gamma
function, and solar
abundances have been assumed in deriving the numerical values. The
values of
is the gamma
function, and solar
abundances have been assumed in deriving the numerical values. The
values of  in
the parentheses give the limits such that the appropriate
integrals converge at large radii. Similarly, the X-ray surface
brightness at a
projected radius b is proportional to the emission measure
EM, defined as
in
the parentheses give the limits such that the appropriate
integrals converge at large radii. Similarly, the X-ray surface
brightness at a
projected radius b is proportional to the emission measure
EM, defined as
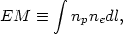
| (5.67) |
where l is the distance along the line-of-sight through the cluster at a projected radius b. Then, for the self-consistent isothermal model, the emission measure is
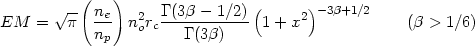
| (5.68) |
where x  b/rc. The microwave diminution
(Section 3.5) at low frequencies is given by
b/rc. The microwave diminution
(Section 3.5) at low frequencies is given by
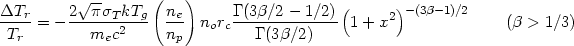
| (5.69) |
where  T is
the Thomson cross section, and Tr is the cosmic
background radiation temperature. Numerically,
T is
the Thomson cross section, and Tr is the cosmic
background radiation temperature. Numerically,
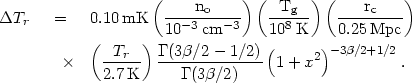
| (5.70) |
Equation (5.68) has been used extensively to model the surface
brightness I(b) of the X-ray emission from clusters
(Gorenstein et
al., 1978;
Branduardi-Raymont et
al., 1981;
Abramopoulos and Ku,
1983;
Jones and Forman, 1984;
Section 4.4.1). The most accurate
data have come from the
Einstein X-ray satellite, which was sensitive only to low energy
X-rays
(h < 4 keV). For high
temperature gas (Tg
< 4 keV). For high
temperature gas (Tg
 3 × 107 K), the low energy X-ray emissivity is nearly
independent of temperature, and thus I(b)
3 × 107 K), the low energy X-ray emissivity is nearly
independent of temperature, and thus I(b)
 EM even if the
gas temperature varies. Large surveys of X-ray distributions fit by
equation (5.68) have been made
by Abramopoulos and Ku
(1983),
who set
EM even if the
gas temperature varies. Large surveys of X-ray distributions fit by
equation (5.68) have been made
by Abramopoulos and Ku
(1983),
who set  = 1
(equal gas and galaxy distributions), and
Jones and Forman (1984),
who allowed
= 1
(equal gas and galaxy distributions), and
Jones and Forman (1984),
who allowed  to vary. Figure 16
shows the data on the X-ray surface brightness of three clusters from
Jones and Forman (1984)
and their best fit models using equation (5.68). Equation (5.68) is
a good fit to the majority of clusters, but fails in the central regions
of some
clusters, possibly because these clusters contain cooling accretion
flows (Sections 4.3.3,
4.4.1, and
5.7).
The average value of
to vary. Figure 16
shows the data on the X-ray surface brightness of three clusters from
Jones and Forman (1984)
and their best fit models using equation (5.68). Equation (5.68) is
a good fit to the majority of clusters, but fails in the central regions
of some
clusters, possibly because these clusters contain cooling accretion
flows (Sections 4.3.3,
4.4.1, and
5.7).
The average value of
 determined
by fits to the X-ray
surface brightness of a large number of clusters was found to be
(Jones and Forman, 1984)
determined
by fits to the X-ray
surface brightness of a large number of clusters was found to be
(Jones and Forman, 1984)
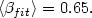
| (5.71) |
Thus the X-ray surface brightness and implied gas density vary on average as

| (5.72) |
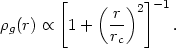
| (5.73) |
This indicates that the gas density should fall off less rapidly with
radius than
the galaxy density (in agreement with many other observations, such as
Abramopoulos and Ku,
1983),
and that the energy per unit mass is higher in the gas than in the galaxies
(Jones and Forman,
1984).
For this average value of
 ,
the total X-ray luminosity converges, but the total gas mass given by
equation (5.65) does not.
,
the total X-ray luminosity converges, but the total gas mass given by
equation (5.65) does not.
Unfortunately, this does not agree with the determinations of the X-ray
spectral temperatures and the galaxy velocity dispersions of clusters
(Mushotzky, 1984).
For example, the observed correlation between
 r and
Tg in equation
(4.10) implies that the average value
r and
Tg in equation
(4.10) implies that the average value
 determined
by gas temperatures and galaxy velocity dispersions is
<
determined
by gas temperatures and galaxy velocity dispersions is
< spect>
spect>
 1.3.
1.3.
From a sample of clusters with well-determined spectra,
Mushotzky (1984)
finds < spect>
spect>
 1.2, which he notes
is about twice the value determined from
observations of the X-ray surface brightness. While
Jones and Forman (1984)
argue that their values of
1.2, which he notes
is about twice the value determined from
observations of the X-ray surface brightness. While
Jones and Forman (1984)
argue that their values of
 fit
are in excellent agreement with the
determinations from spectral observations, in fact their data show that
the two values do not agree to within the errors in the majority of
cases. For the clusters they studied
<
fit
are in excellent agreement with the
determinations from spectral observations, in fact their data show that
the two values do not agree to within the errors in the majority of
cases. For the clusters they studied
< spect>
spect>
 1.1. Thus the general
result seems to be that
1.1. Thus the general
result seems to be that

| (5.74) |
A number of suggestions have been made as to the origin of this discrepancy.
First, the gas may very well not be isothermal. However,
Mushotzky (1984)
has argued that the same problem occurs for other thermal distributions
in the gas. Second, it may be that the line-of-sight velocity dispersion
does not represent accurately the energy per unit mass of the
galaxies. Equation (5.63) assumes that
the galaxy velocity distribution is isotropic. The distribution could be
anisotropic, either if the cluster is highly flattened
(Section 2.9.3) or if
the cluster is spherical but galaxy orbits are largely radial (rather than
having a uniform distribution of eccentricities; see
Section 2.8). A detailed study
(Kent and Sargent, 1983)
of the positions and velocities of galaxies
in the Perseus cluster has produced a more accurate
description of the
cluster potential and significantly reduced the discrepancy, although it
still is significant. Third, it may be that many of the galaxy velocity
dispersions measured for clusters are contaminated by foreground or
background groups
(Geller and Beers,
1982).
The velocity dispersions may
also be affected by subclustering or nonvirialization of the cluster. All
of these effects will cause the data to overestimate the actual velocity
dispersion (the cluster potential), and thus to overestimate
 spect. Perhaps one
indication that such systematic errors in the velocity dispersion might be
occurring is that Coma, the best studied regular cluster, does not show
a
spect. Perhaps one
indication that such systematic errors in the velocity dispersion might be
occurring is that Coma, the best studied regular cluster, does not show
a  discrepancy. Another possible solution to the
discrepancy. Another possible solution to the
 discrepancy
would be if the gas dominated the total cluster mass at large radii
(Henriksen and
Mushotzky, 1985;
Section 5.5.5);
this would invalidate the assumption that the galaxies and gas were test
particle distributions in the missing mass potential.
discrepancy
would be if the gas dominated the total cluster mass at large radii
(Henriksen and
Mushotzky, 1985;
Section 5.5.5);
this would invalidate the assumption that the galaxies and gas were test
particle distributions in the missing mass potential.
Finally, let me point out an utterly trivial possible explanation of the
 discrepancy. The particular form of the gas distribution in the
self-consistent isothermal model as given above (equations 5.63 through
5.70) depends on assuming that the cluster potential is fit by the
King approximation to the
isothermal sphere (equations 5.59 and 5.61). This approximation breaks
down at large radii, where the King model density varies as
discrepancy. The particular form of the gas distribution in the
self-consistent isothermal model as given above (equations 5.63 through
5.70) depends on assuming that the cluster potential is fit by the
King approximation to the
isothermal sphere (equations 5.59 and 5.61). This approximation breaks
down at large radii, where the King model density varies as

 r-3
while a real isothermal sphere density varies as
r-3
while a real isothermal sphere density varies as

 r-2. Of course, it
is the gas distribution at large radii which has the greatest leverage in
affecting the fit to equation 5.63. If the mass density in clusters is
really
isothermal, then equation 5.63 will not fit the observed gas
distribution for the correct value of
r-2. Of course, it
is the gas distribution at large radii which has the greatest leverage in
affecting the fit to equation 5.63. If the mass density in clusters is
really
isothermal, then equation 5.63 will not fit the observed gas
distribution for the correct value of
 . As an
alternative, let us assume that the cluster mass
distribution can be fit by the simple analytic form
. As an
alternative, let us assume that the cluster mass
distribution can be fit by the simple analytic form
 (r)
=
(r)
=  0(1
+ x2)-1, which is
similar to equation (5.57) but has the correct asymptotic form for an
isothermal sphere. Then all the formulae for the self-consistent isothermal
sphere remain unchanged if we substitute
0(1
+ x2)-1, which is
similar to equation (5.57) but has the correct asymptotic form for an
isothermal sphere. Then all the formulae for the self-consistent isothermal
sphere remain unchanged if we substitute

 (2/3)
(2/3) in
equations (5.63) through (5.70). Retaining the present definition of
in
equations (5.63) through (5.70). Retaining the present definition of
 , this is
equivalent to
, this is
equivalent to
 fit
= (2/3)
fit
= (2/3) spect, which is essentially consistent with the
observations. The observed
<
spect, which is essentially consistent with the
observations. The observed
< fit>
fit>
 2/3 implies
<
2/3 implies
< spect>
spect>
 1 which is consistent
with the spectral observations. Another way of expressing all this is to
say that the observed gas distribution (equation 5.73) is essentially
that of an isothermal sphere.
1 which is consistent
with the spectral observations. Another way of expressing all this is to
say that the observed gas distribution (equation 5.73) is essentially
that of an isothermal sphere.
This possible discrepancy between the globally determined X-ray temperatures of clusters and their surface brightness will probably only be resolved when spatially resolved X-ray spectra are available, allowing a simultaneous determination of the spatial variation of the gas density and temperature (Chapter 6).
One of the derivations of the self-consistent isothermal model involves
noting that the galaxy distribution also solves the hydrostatic equation
if the galaxy velocity dispersion is isotropic (see discussion following
equation (5.64)). If the
galaxy distribution is spherical, but the galaxy velocity dispersions in the
directions parallel to the cluster radius
( r)
and transverse to that direction
(
r)
and transverse to that direction
( t)
are different, this derivation fails. However, for a constant
(isothermal) galaxy
velocity dispersion and a constant anisotropy of the velocity dispersion
t)
are different, this derivation fails. However, for a constant
(isothermal) galaxy
velocity dispersion and a constant anisotropy of the velocity dispersion

 1 -
1 -
 t2
/
t2
/  r2,
the gas density is given by
r2,
the gas density is given by
 g
g
 (
( gal
r2
gal
r2 )
) (White, 1985).
(White, 1985).
Finally,
Henry and Tucker (1979)
have pointed out the existence of a simple
relationship (Lx rc)2/5
 Tg
between X-ray temperatures, luminosities, and core
radii in clusters based on the isothermal model.
Tg
between X-ray temperatures, luminosities, and core
radii in clusters based on the isothermal model.