


3.1. Plane Waves in a Thin Mass Sheet
Since solutions of Poisson's equation can be obtained analytically
only for the simplest mass distributions, theoretical treatments
necessarily make quite drastic simplifying assumptions. Local theory,
summarized in this section, is built around a WKB-type potential
solution for density variations in the form of short wavelength plane
waves in a locally uniform, razor thin disk. In this case, a
plane-wave disturbance
 1
eikx to the surface density in the
(x, y)-plane at some instant gives rise to the potential
1
eikx to the surface density in the
(x, y)-plane at some instant gives rise to the potential
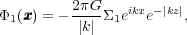 |
(7) |
(cf. eq. 6.31 of BT08). This relation strictly applies only to straight waves of infinite extent. However, the sinusoidal density variations cause the field of distant waves to cancel quickly, and the formula is reasonably accurate near the center of a short wave packet. Note that eq. (7) does not depend on the inclination of the wavefronts to the radial direction, but the curvature of the wavefronts must also be negligible, which requires |kR| >> 1, with R being the distance from the disk center.
A dispersion relation gives the relationship between the wavenumber
k and frequency  of self-consistent waves. Assuming (a)
the above relation between density and potential for a razor-thin disk
and (b) the wave is of small amplitude, so that second order terms are
negligible, the local dispersion relation for short axisymmetric
density waves may be written
of self-consistent waves. Assuming (a)
the above relation between density and potential for a razor-thin disk
and (b) the wave is of small amplitude, so that second order terms are
negligible, the local dispersion relation for short axisymmetric
density waves may be written
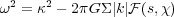 |
(8) |
(Kalnajs 1965),
where  is the local
undisturbed surface density and the complicated reduction factor
is the local
undisturbed surface density and the complicated reduction factor
 , with two
dimensionless arguments, is explained below.
Lin & Shu (1966)
independently derived a generalized relation for tightly-wrapped
spiral waves, which required two additional assumptions: (c) that the wave
vector k is approximately radial, and (d) that the disturbance is
not close to any of the principal resonances. Their better-known
relation simply replaces the frequency of the wave with the
Doppler-shifted forcing frequency experienced by the orbit guiding
center:
, with two
dimensionless arguments, is explained below.
Lin & Shu (1966)
independently derived a generalized relation for tightly-wrapped
spiral waves, which required two additional assumptions: (c) that the wave
vector k is approximately radial, and (d) that the disturbance is
not close to any of the principal resonances. Their better-known
relation simply replaces the frequency of the wave with the
Doppler-shifted forcing frequency experienced by the orbit guiding
center:
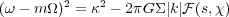 |
(9) |
(see also BT08 Section 6.2.2), where now

 m
m  p.
p.
The reduction factor,
 , is always positive and is
unity when the disk has no random motion. Since all the factors in the
self-gravity term on the RHS are intrinsically positive,
eqs. (8) & (9) say that self-gravity enables a
supporting response from the stars at a frequency that is lower than
their free epicycle frequency
, is always positive and is
unity when the disk has no random motion. Since all the factors in the
self-gravity term on the RHS are intrinsically positive,
eqs. (8) & (9) say that self-gravity enables a
supporting response from the stars at a frequency that is lower than
their free epicycle frequency
 .
.
When the disk has random motion, the vigor of the stellar response
depends on two factors: the ratio of the forcing frequency experienced
by a star to its natural frequency, s
 |
| -
m
-
m | /
| /
 , and the ratio of the
typical sizes of the stellar
epicycles (
, and the ratio of the
typical sizes of the stellar
epicycles ( < vR2 >1/2 /
< vR2 >1/2 /
 ) to the wavelength of
the wave (
) to the wavelength of
the wave ( |k|-1). Thus for a Gaussian
distribution of radial velocities,
|k|-1). Thus for a Gaussian
distribution of radial velocities,
 is a function of both
s and
is a function of both
s and 

 R2
k2
/
R2
k2
/  2. Clearly,
when
2. Clearly,
when  is
large, most stars will oscillate over many radial waves, and the
weakened supporting response arises mainly from the small fraction of
stars near the center of the velocity distribution.
is
large, most stars will oscillate over many radial waves, and the
weakened supporting response arises mainly from the small fraction of
stars near the center of the velocity distribution.
The dispersion relation for barotropic fluid (gaseous) disks 2 is similar (BT08 eq. 6.55)
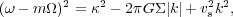 |
(10) |
where vs is the sound speed in the gas. Both dispersion relations are shown graphically in Fig. 4, below.
Toomre (1964)
determined the condition for marginal stability of short
axisymmetric waves by solving for
 2 = 0 in
eq. (8). Since all the factors in the self-gravity term are
intrinsically positive,
2 = 0 in
eq. (8). Since all the factors in the self-gravity term are
intrinsically positive,
 2 could be
negative for large |k|, implying instability. Without random motion,
2 could be
negative for large |k|, implying instability. Without random motion,
 = 1 and short
waves with k > kcrit or
= 1 and short
waves with k > kcrit or
 <
<
 crit =
4
crit =
4 2
G
2
G /
/
 2, will be
unstable. Unlike for the Jeans instability in a stationary medium,
longer waves are stabilized by rotation, embodied in the
2, will be
unstable. Unlike for the Jeans instability in a stationary medium,
longer waves are stabilized by rotation, embodied in the
 2 term.
2 term.
For disks with random motion, the reduction factor

 0 for large
|k|, irrespective of the frequency, for the
reason given above. Thus random motion stabilizes short waves, as for
the Jeans instability.
Toomre (1964)
showed for a Gaussian
distribution of radial velocities that the RHS of eq. (8) is
0 for large
|k|, irrespective of the frequency, for the
reason given above. Thus random motion stabilizes short waves, as for
the Jeans instability.
Toomre (1964)
showed for a Gaussian
distribution of radial velocities that the RHS of eq. (8) is
 0 for all |k| when
0 for all |k| when
 R
R

 R,crit, where
R,crit, where
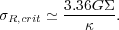 |
(11) |
Thus Toomre's local axisymmetric stability criterion is Q

 R
/
R
/  R,crit
R,crit
 1. When reasonably
constant with radius, the locally estimated Q value is a good
indicator of global axisymmetric stability. For example, the local
values in the equilibrium models proposed by
Kalnajs (1976)
are in reasonable agreement with those he derived from global axisymmetric
modes (his Fig. 2). It should be noted that an axisymmetrically
stable disk, i.e. for which Q
1. When reasonably
constant with radius, the locally estimated Q value is a good
indicator of global axisymmetric stability. For example, the local
values in the equilibrium models proposed by
Kalnajs (1976)
are in reasonable agreement with those he derived from global axisymmetric
modes (his Fig. 2). It should be noted that an axisymmetrically
stable disk, i.e. for which Q
 1 everywhere, could still be
unstable to non-axisymmetric modes.
1 everywhere, could still be
unstable to non-axisymmetric modes.
The local axisymmetric stability criterion for rotating gas disks is
similar. The longest unstable wavelength,
 crit in a
cold (vs = 0) disk is the same as for a stellar
disk. The minimum of
the quadratic expression in k on the RHS of eq. (10) occurs
for k =
crit in a
cold (vs = 0) disk is the same as for a stellar
disk. The minimum of
the quadratic expression in k on the RHS of eq. (10) occurs
for k =  G
G /
vs2,
and the condition of marginal stability
(
/
vs2,
and the condition of marginal stability
( 2 = 0) at
this minimum is readily solved, yielding
vs,crit =
2 = 0) at
this minimum is readily solved, yielding
vs,crit =  G
G /
/
 .
.
Vandervoort (1970),
Romeo (1992),
and others have extended the discussion
of axisymmetric stability to disks of finite thickness. The principal
difference is the dilution of the self-gravity term caused by
spreading the disk matter in the vertical direction, resulting in a
somewhat more stable disk. The precise correction depends on the
assumed vertical structure of both the disk and the wave, but is minor
when the characteristic disk thickness z0 <<
 crit,
which is usually the case.
crit,
which is usually the case.
More realistic composite disks consist of multiple stellar populations
having a range of velocity dispersions, as well as a cool gas
component.
Jog & Solomon
(1992)
considered the stability of two
gravitationally coupled gas disks having different sound speeds, but
Rafikov (2001)
improved their analysis to include multiple stellar
components. These analyses show that while the gas component may
contain a small fraction (typically
 10%) of the total
mass, its low sound speed causes it to have a disproportionate effect on
the overall stability.
10%) of the total
mass, its low sound speed causes it to have a disproportionate effect on
the overall stability.
3.4. Self-consistent Density Waves
Fig. 4 gives, for four different values of
Q, a
graphical representation of the stellar (solid curves),
3 and gaseous (dashed
curves) dispersion relations
eqs. (9) & (10) respectively. The curves do not
differ for Q = 0 for which s is imaginary when k
> kcrit.
The dashed and solid curves have similar forms for moderate k but
differ substantially for large k, where frequencies in the stellar
sheet cannot exceed
| -
m
-
m | =
| =
 . Thus waves in a
stellar sheet extend only from CR, where s = 0, to the Lindblad
resonance on either side, where s = 1.
4
. Thus waves in a
stellar sheet extend only from CR, where s = 0, to the Lindblad
resonance on either side, where s = 1.
4
A gas layer, on the other hand, can support gravitationally modified sound waves of arbitrarily high frequency. Note that this important difference from the stellar case can lead to artificial modes when hydrodynamic equations are used as a simplifying approximation for a stellar disk; while meaningful results can be obtained using this approximation, it is important to check that the derived modes do not cross Lindblad resonances.
Since the dispersion relation (9) does not depend on the sign
of k, curves for negative k, or leading waves, are simple
reflections about the k = 0 axis. Furthermore, the relation gives
the square of ( -
m
-
m ), implying
that solutions when this
frequency is negative, which arise inside CR, are simple reflections
about the s = 0 axis. Thus were the signs not suppressed,
Fig. 4 shows only the panel for trailing waves
outside CR, while the other quadrants would be reflected images.
), implying
that solutions when this
frequency is negative, which arise inside CR, are simple reflections
about the s = 0 axis. Thus were the signs not suppressed,
Fig. 4 shows only the panel for trailing waves
outside CR, while the other quadrants would be reflected images.
In the quadrant shown, there are either two values of k for each
value of s, known as the short- and long-wave branches,
or there are none. Only for the marginally stable case of Q = 1 do
solutions exist for all frequencies 0
 s
s
 1 and all |k|.
When Q > 1, a forbidden region opens up in the vicinity of
CR where waves are evanescent.
1 and all |k|.
When Q > 1, a forbidden region opens up in the vicinity of
CR where waves are evanescent.
Recall that the relation (9) was derived assuming both |kR|
>> 1 and that the waves are tightly wrapped - i.e. that the
wave vector is directed radially. These approximations may not be too bad
on the short-wave branch (large k) but long-wave branch solutions
may not exist at all, except in a very low-mass disks where
kcrit is large, and must fail as |k|
 0.
Lynden-Bell &
Kalnajs (1972)
gave an improved dispersion relation (their eq. A11) that
relaxed the requirement that the wave vector be radial, but still used
the plane wave potential (7) that requires |kR| >> 1.
Their relation is no different for tightly-wrapped waves, but has no
long-wave branch or forbidden region for open waves when Q
0.
Lynden-Bell &
Kalnajs (1972)
gave an improved dispersion relation (their eq. A11) that
relaxed the requirement that the wave vector be radial, but still used
the plane wave potential (7) that requires |kR| >> 1.
Their relation is no different for tightly-wrapped waves, but has no
long-wave branch or forbidden region for open waves when Q
 1.
1.
The waves in a disk described by the local dispersion relations
(9) & (10) have a phase speed, equal to the pattern
speed, in the azimuthal direction. However,
Toomre (1969)
pointed out that these dispersive waves also have a group velocity that is
directed radially. Since vg =

 /
/
 k, the group
velocity is proportional to the slope of the lines in
Fig. 4. Each portion of a wavetrain, or wave
packet, is carried radially at the group velocity, retaining its pattern
speed
k, the group
velocity is proportional to the slope of the lines in
Fig. 4. Each portion of a wavetrain, or wave
packet, is carried radially at the group velocity, retaining its pattern
speed  whilst gradually
adjusting its wavelength, and also
transporting wave action or angular momentum.
whilst gradually
adjusting its wavelength, and also
transporting wave action or angular momentum.
For trailing waves outside CR, the situation illustrated in Fig. 4, the group velocity is positive on the short-wave branch, and the waves carry angular momentum outwards towards the OLR. The curves have the opposite slope inside CR, where density waves are disturbances of negative angular momentum (stars there lose angular momentum as the wave is set up, Lynden-Bell & Kalnajs 1972), and therefore the inward group velocity on the short-wave branch leads once more to outward angular momentum transport. Outward angular momentum transport is in the sense expected from the gravitational stresses of a trailing spiral (Lynden-Bell & Kalnajs 1972).
However, the sign of the slope

 /
/
 k on the
dubious long-wave branch is opposite to that of short waves,
indicating that angular momentum in that regime is transported in the
direction opposite to the gravity torque! This apparent contradiction
is resolved by the phenomenon of lorry transport, a term coined by
Lynden-Bell &
Kalnajs (1972)
to describe an advective Reynolds stress (see also
Appendix J of BT08). Stars in their epicyclic oscillations gain
angular momentum from the wave near apo-center and lose it back to the
wave near peri-center; no star suffers a net change, but angular
momentum is still carried inward at a sufficient rate to overwhelm the
gravity torque.
k on the
dubious long-wave branch is opposite to that of short waves,
indicating that angular momentum in that regime is transported in the
direction opposite to the gravity torque! This apparent contradiction
is resolved by the phenomenon of lorry transport, a term coined by
Lynden-Bell &
Kalnajs (1972)
to describe an advective Reynolds stress (see also
Appendix J of BT08). Stars in their epicyclic oscillations gain
angular momentum from the wave near apo-center and lose it back to the
wave near peri-center; no star suffers a net change, but angular
momentum is still carried inward at a sufficient rate to overwhelm the
gravity torque.
Thus where eq. (9) holds in a disk with approximately uniform
Q  1, a
tightly wrapped trailing wave packet originating
on the long-wave branch, will travel towards CR until it reaches the
edge of the forbidden zone where it "refracts" into a short wave
that carries it away towards the Lindblad resonance. The details of
the turning point at the forbidden zone, which requires an evanescent
wave propagating into the forbidden region, were described by
Mark (1976)
and by
Goldreich &
Tremaine (1978).
1, a
tightly wrapped trailing wave packet originating
on the long-wave branch, will travel towards CR until it reaches the
edge of the forbidden zone where it "refracts" into a short wave
that carries it away towards the Lindblad resonance. The details of
the turning point at the forbidden zone, which requires an evanescent
wave propagating into the forbidden region, were described by
Mark (1976)
and by
Goldreich &
Tremaine (1978).
The fate of the short-wave propagating towards theLindblad resonance also requires more sophisticated analysis because the dispersion relation (9) does not hold near resonances. Mark (1974) carried the analysis to second order to show that the wave is absorbed there through the wave-particle coupling described by Lynden-Bell & Kalnajs (1972).
Goldreich &
Lynden-Bell (1965b),
Julian & Toomre
(1966)
and
Toomre (1981)
extended the above analysis
for tightly-wrapped waves to waves of arbitrary inclination. They
again adopted the approximate potential of a short-wavelength plane
wave (eq. 7), and focused on a local patch of the disk
whose dimensions were assumed small compared with R0,
the radial distance of the patch center, which orbits the disk center at
the local circular speed V = R0
 0.
0.
Following
Hill (1878),
they then introduced Cartesian-like
coordinates, x  R - R0 and y
R - R0 and y
 R0(
R0( -
-
 0),
where
0),
where
 0
is the azimuth of the orbiting center of the patch. The
vertical coordinate, z, is unchanged from the usual cylindrical
polar coordinate. Since the angular rotation rate decreases outwards
in galaxies, the motion of stars in the patch is that of a shear flow,
with a speed that varies as
0
is the azimuth of the orbiting center of the patch. The
vertical coordinate, z, is unchanged from the usual cylindrical
polar coordinate. Since the angular rotation rate decreases outwards
in galaxies, the motion of stars in the patch is that of a shear flow,
with a speed that varies as
 =
-2Ax, together with Coriolis
forces arising from the rotation of the patch at angular rate
=
-2Ax, together with Coriolis
forces arising from the rotation of the patch at angular rate
 0. The
Oort "constant" A = -1/2 R
d
0. The
Oort "constant" A = -1/2 R
d / dR. The undisturbed surface density of matter,
/ dR. The undisturbed surface density of matter,
 , is assumed constant
over the patch. This set of approximations is described as the
sheared sheet (see BT08 pp. 678-681).
, is assumed constant
over the patch. This set of approximations is described as the
sheared sheet (see BT08 pp. 678-681).
If  is the inclination
angle of a plane density wave to the
azimuthal direction, with 180° >
is the inclination
angle of a plane density wave to the
azimuthal direction, with 180° >
 > 90° for leading
waves and 90° >
> 90° for leading
waves and 90° >  > 0° for trailing waves, then the
shear causes the pitch angle to change with time as cot
> 0° for trailing waves, then the
shear causes the pitch angle to change with time as cot
 = 2At, with t = 0 when
= 2At, with t = 0 when
 = 90°, i.e.
when the wave is purely radial. Both
Goldreich &
Lynden-Bell (1965b)
for a gaseous sheet and
Julian & Toomre
(1966)
for a stellar sheet found waves amplify strongly, but transiently, as
they shear from leading to trailing.
= 90°, i.e.
when the wave is purely radial. Both
Goldreich &
Lynden-Bell (1965b)
for a gaseous sheet and
Julian & Toomre
(1966)
for a stellar sheet found waves amplify strongly, but transiently, as
they shear from leading to trailing.
The unremitting shear flow ultimately tears apart any transient
disturbance, and indeed
Julian & Toomre
(1966)
asserted that the stellar sheet is
locally stable at small-amplitudes when Q
 1, but they did not
give the proof.
1, but they did not
give the proof.
Fig. 5, from Toomre (1981), illustrates the dramatic transient trailing spiral that results from a small input leading disturbance. This calculation illustrates that even a global, small-amplitude disturbance does not persist, but decays after its transient flourish. In the late stages, the "wave action" propagates at the group velocity (Section 3.5) away from corotation, where it is absorbed at the Lindblad resonances.
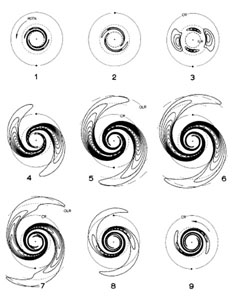 |
Figure 5. The time evolution of an input leading wave packet in the half-mass Mestel disk. The unit of time is half a circular orbit period at the radius marked CR. Reproduced from Toomre (1981). |
Notice also that the wave in this Q = 1.5 disk extends across the forbidden region near CR. The absence of solutions near s = 0 when Q > 1 in Fig. 4 is a consequence of assuming a steady, tightly-wrapped wave; shearing waves do not respect this restriction.
The responsiveness of a disk to input leading signal depends both on
the degree of random motion - it decreases rapidly over the range 1
< Q < 2 - and upon the azimuthal wavelength, which is
 y =
2
y =
2 R / m for an
m-armed pattern.
Julian & Toomre
(1966)
defined the parameter
R / m for an
m-armed pattern.
Julian & Toomre
(1966)
defined the parameter
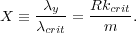 |
(12) |
In a disk having a flat rotation curve,
Toomre (1981)
showed that the strongest amplification occurs when 1
 X
X
 2.5, or about
twice the scale of the longest unstable axisymmetric wave. The
peak amplification shifts to larger X values for declining rotation
curves and vice versa for rising ones. Naturally, the
phenomenon of swing amplification disappears entirely in uniformly
rotating disks.
2.5, or about
twice the scale of the longest unstable axisymmetric wave. The
peak amplification shifts to larger X values for declining rotation
curves and vice versa for rising ones. Naturally, the
phenomenon of swing amplification disappears entirely in uniformly
rotating disks.
By making the assumption that spiral patterns in galaxies are
amplified solely by this mechanism,
Athanassoula et
al. (1987)
argued that the
multiplicity of the observed spiral arm pattern can be used to place
bounds on the mass density in the disk. The circular orbit speed in a
nearly axisymmetric galaxy affords a direct estimate of the central
attraction, but it is hard to determine how much of the total
attraction is contributed by the disk, and how much by other
components, such as the dark matter halo. Because
 crit is
proportional to the disk surface density, for
a given rotation curve, the most vigorous amplification shifts to higher
sectoral harmonics in lower mass disks. Generally, disks that
contribute most of the central attraction, i.e. close to maximum
disks, would prefer bi-symmetric spirals, while higher arm
multiplicities would indicate significantly sub-maximal disks
(see also
Sellwood &
Carlberg 1984).
crit is
proportional to the disk surface density, for
a given rotation curve, the most vigorous amplification shifts to higher
sectoral harmonics in lower mass disks. Generally, disks that
contribute most of the central attraction, i.e. close to maximum
disks, would prefer bi-symmetric spirals, while higher arm
multiplicities would indicate significantly sub-maximal disks
(see also
Sellwood &
Carlberg 1984).
2 Goldreich & Lynden-Bell (1965a) derived the vertically-integrated equations for "2D pressure" in a gas disk. Back.
3 The reduction factor used assumes a Gaussian distribution of radial velocities. Back.
4 Additional Bernstein-type waves exist near integer values of s > 1, but such solutions seem to be of little dynamical importance. Back.