Appendix V. Cumulative Gaussian and Chi-Squared Distributions

The ² confidence limit is the probability of Chi-squared exceeding the observed value; i.e.,

where P_p for p degrees of freedom is given by Eq. (30a).

Gaussian Confidence Limits

Let ² = [x / ]². Then for n_D = 1,

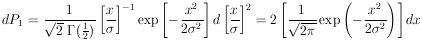
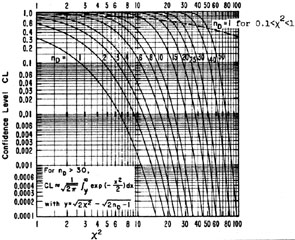
Thus CL for n_D is twice the area under a single Gaussian tail. For example the n_D = 1 curve for ² = 4 has a value of CL = 0.046. This means that the probability of getting | x| 2 is 4.6% for a Gaussian distribution.

Figure 10. 2 Confidence Level vs. 2 for nD Degrees of Freedom (9).