7. THE PHYSICS OF THE PHASE DIAGRAM

7.1. Energy densities in the two phases

At temperature much larger than the transition temperature, we expect the particles of the glue to behave as free particles, ignoring completely the presence of the color force. Thus, as for a photon gas, we expect the energy density to be proportional to T⁴, (eq. (6) with a numerical coefficient (demographic coefficient) given by the sum of the multiplicity of all the relativistic particle species in presence (quarks and gluons above the critical temperature; pions below). Thus, as we cross the border, we expect a decrease in the energy density (proportional to T⁴) as given by the ratio of the demographic coefficients. More on this later.

7.1.1. The bag models

A simplified phenomenological model has been devised to give a more intuitive representation of the situation: the bag model of the nucleon (Thomas 1984).

The quarks are assumed to move freely in a bag. At the border of the bag, they meet a potential which keeps them confined. There are several versions. The MIT bag is characterized by a sharp boundary with radius 1 fm. In the chiral bag model, the quarks are confined to a much smaller region. The outer region is made of pions coupled to the quarks at the surface of the bag.

The main idea is to introduce in the lagrangian of the model a vacuum energy density term B. For the MIT bag the value of B(MIT) = (145 MeV)⁴, while, because of the smaller size bag, the chiral B is higher: B(chiral) = (276 MeV)⁴. (As discussed before, it is convenient to measure energy densities in units of the equivalent radiation density (T⁴) at temperature T).

A negative pressure term is also introduced in the stress-energy tensor to preserve Lorentz invariance. Its physical meaning can be seen in the following way. The pressure in the glue, becomes:

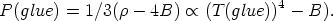
(19)

At high T the quarks behave as free relativistic particles (P = (1/3) T⁴). But as T approaches B^1/4, the attractive force slowly reduces the pressure.

The term B also appears in the cosmic expansion equation.

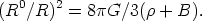
(20)

If, as suggested by the preliminary QCD calculations, the transition is first-order, the universe supercools below the critical temperature which, in this model, is T B^1/4. Then nucleation sets-in and pockets of hadrons (mesons and nucleons) appear in the glue. By surface effects, they grow and non-adiabatically release vacuum-energy to the radiation gas, thus reheating the universe.

As in the case of earlier phase transitions (GUT at T = 10¹⁵ GeV; Weinberg-Salam at T = 10² GeV) we expect the QH phase transition to accelerate the expansion rate, inducing a period of inflation. Compared to the previous chapter of inflation, this one will be very modest, leading to an increase of at best fifteen percent in the cosmic scale factor.

7.1.2. A simple model of the Q-H phase transition

A first estimation of the properties of the Q-H phase transition can be obtained with the help of a simplified model of the two phases. We assume no net baryon number (equal number of particle and antiparticles). In other words we set the baryon chemical potential µ equal to zero. Ideal gas properties are assumed for both phases.

In the low temperature case we have a gas of massless, non-interacting pions (three varieties; ⁰, ⁺, ^-, thus the factor 3 in the following equations). The energy density is then given by

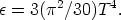
The relativistic pressure is one third of the energy density:

At high temperature we have a relativistic gas of zero mass quarks and gluons. To obtain free quarks, one adds to the kinetic energy density the B term introduced previously. The Lorentz invariance of the stress-energy tensor then forces us to add a negative term (-B) to the pressure. (This can also be obtained simply from the relation dE = - PdV and E = BV with B = cst.) This term will play the role of an effective cosmological constant in our astrophysical discussion. (The term B is often assigned to the confined phase instead of the unconfined phase as here. In this case the (then positive) pressure B has the physical meaning of a pressure from the vacuum to keep the quarks inside their confinement volume.)

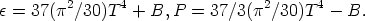
The multiplicity factor 37 has the following interpretation. For the gluons: 16 [2, for the spin, times 8, for the number of color charges]. For the quarks: 21 [3, for the colors, times 2, for the quarks and antiquarks, times 2, for the savors (only the u and the d are considered here), times 2, for the spin, times a factor 7/8, from the nondimensional integral appropriate to the fermions.]

In fig. 8a the pressure of the two phases is plotted as a function of the temperature. The phase with the higher pressure is the stable one. The transition takes place at the critical temperature T_c at which the two pressures are equal.

In the fig. 8b the energy densities of the two phases are plotted in the same units. At T_c there is a density energy jump of

Fig. 8c gives the equation of state P = P(). The entropy density is given by: s = 1/VdP/dT|_V = 4( / 30) / 3T³ for each boson and the same number x × 7/8 for each fermion.

Thus s (q and g) = 37(4/3)( / 30)T³; s(pions) = 4( / 30)T³.

In fig. 8d, s is plotted as a function of T³. The jump of s at the critical temperature is the sign of a first order transition (in this model dots). One finds = T_c s as expected.

This simple model serves to illustrate the physics. In the high temperature phase, the increase in the number of degree of freedom (37 compared to 3) is couterbalanced by the vacuum density energy needed to free the quarks. The transition takes place at the critical temperature where the two effects compensate each other. The difference between the number of degree of freedom gives a measure of the entropy density to be released during the transition.

Figure 8. Illustration of the ideal gas model of the transition, given in the text for pedagogical reasons.