1.4. Dispersion orbits

Most remarkably after that fine beginning [in 1925-27], it took
Lindblad not three further months or years, but three whole
decades, to connect this implied epicyclic frequency and the
ordinary angular speed of rotation into the kinematic wave
speeds like ± /m, which we very much associate with him
nowadays, especially when muttering phrases like `Lindblad resonances'.
Toomre 1996, p.2-3

These fresh winds did not catch Lindblad unawares. The importance of differential rotation was already conceived by him from radio observations (Kwee et al 1954; Schmidt 1956), and he even noticed - for the Galaxy and, later, for M31 (van de Hulst et al 1957) and M81 (Munch 1959) - the curious empirical near-constancy of a combination

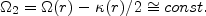(4)

And the dynamical stability problems were always comprised by his spiral theories. Already from 1938 on, dispersion relations of type (3) surfaced in his evolving papers, growing more and more complicated by way of various gradient-term inclusions for a tentatively better description of the crucial - unstable - bar-mode (see Genkin & Pasha 1982). ³²

However, the idea of applying the collective-dynamical methods to shearing stellar galaxies hardly ever impressed Lindblad. He must have felt (Lindblad 1959) the limits of his hydrodynamical approach (long-wave solutions at differential rotation were unattainable analytically, while, on the short-wave side, the whole approach failed for want of an equation of state), not having yet a means of solving kinetic equations. Also, Lindblad perhaps doubted the very possibility of steady modes in shearing galaxies. Either way, the empirical relation (4) that he himself had stated inspired him the most. With it as a centerpiece he started a new, "more definite theory of the development of spiral structure" (Lindblad 1962b, p.148), one he called the dispersion orbit theory (Lindblad 1956, 1961). It was imbued, intuitively, with a hope that gas and Population I stars "are somehow aggregated on their own into a few such orbits in each galaxy - almost like some vastly expanded meteor streams" (Toomre 1996, p.3).

Lindblad described epicyclic stellar oscillations in a reference system rotating with angular velocity _n = - / n, n = d / d, and he imagined a star's radial displacement to depend on its azimuth as cos n( - ₀), ₀ being apocentric longitude. The simplest forms of orbits occurred for integer n's, the case of n = 2 satisfying the empirical condition (4). For this case, "the most general form of an ellipsoidal distribution with vertex deviation" was obtained (Lindblad 1962b, p.152), with which Lindblad sought to calculate the total gravitational potential and, by extracting its averaged (over time and angle) part, to treat the remainder as a contribution to the perturbing force. He Fourier-decomposed this force and retained the m = 1, 2 harmonics to analyze disturbances to a ring of radius r composed of small equal-mass particles. Like Maxwell (1859) in his similar Saturn ring problem, ³³ Lindblad obtained four basic modes for each m. Two of them described nearly frozen, practically co-rotating with material, disturbances to the ring density. Two others - "deformation waves" - ran with speeds ± / m, the minus sign being for the slower mode. It was, at m = 2, "essentially this slowly advancing kinematic wave [...] composed of many separate but judiciously-phased orbiting test particles" (Toomre 1977, p.441) that Lindblad meant by his dispersion orbit (). The fact that its angular velocity was independent of radius, _p(r) = ₂ = const (with an observational accuracy of the condition (4)), implied a stationary state for all test rings, i.e. over the entire radial span where this condition was well obeyed.

"This fact greatly intrigued Lindblad - who did not need to be told that strict constancy [of _p(r)] would banish wrapping-up worries or that the nicest spirals tend to have two arms. Yet astonishingly, that is about as far as he ever got. [...] It never occurred very explicitly to [him ...] to combine already those `orbits' into any long-lived spiral patterns" (Toomre 1977, p.442).

³² Lindblad's dispersion relation in its simplest form (Lindblad 1938) was rather similar to Safronov's relation (3), both showed the same terms, but, as Lindblad was focused on global modes and Safronov dealt with short-wave radial oscillations only, their treatment of the correcting factor in gravity term was technically different. Still, "Lindblad, despite all his words, never quite seemed to relate those formulas to any spiral structures, and [...] only applied them literally to non-spiral or bar-like disturbances". (Toomre) Back.

³³ Maxwell's problem was on disturbances of N equal-mass particles placed at the vertices of an N-sided regular polygon and rotating in equilibrium around a fixed central body. Back.