4. GLOBAL EVIDENCE FOR MAGNETIC ENERGY IN INTERGALACTIC SPACE

4.1. Black holes as sources of IGM magnetic fields

The very largest BH-fed radio sources appear to have lost the least energy in other forms (e.g. PdV expansion work) as they expand into space, and in this sense are the best quantitative calibrators of gravitational-to-magnetic energy in the IGM [19]. Figure 9 illustrates this with a plot of the estimated energy content of giant radio sources. It is less than 2 orders of magnitude below the gravitational infall energy (to the Schwarzschild radius) of a 10⁹ M black hole. Various corrections discussed in [19], such as particle diffusion etc. would correct the GRG points upward, and the BH energy downward, thus further narrowing the energy gap. The gap between the cluster sources and the giant radio lobes is approximately the independently measurable PdV work done as the lobes expand against the pressure of the ICM.

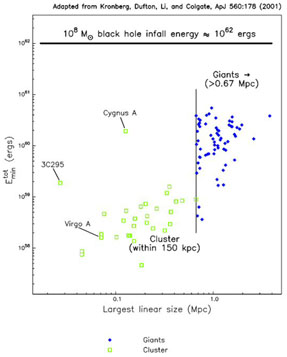Figure 9. Radio lobe energy content for extended sources within galaxy clusters (squares) and the very largest BH-powered radio sources (diamonds) showing that the upper envelope of the latter is within 2 orders of magnitude of the putative gravitational formation energy of the central supermassive black hole [19].

The high efficiency of the gravitational to magnetic energy conversion implied by Fig 8, combined with the known space density of > 10⁶ M black holes, ~ 10⁵ M / Mpc³, implies a global magnetic energy density in the galaxy over-dense zones (the galaxy filament zones of LSS) which is

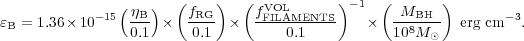(4)

_B is the intergalactic energy density, _B is gravitational to magnetic energy conversion efficiency factor, f_RG the fraction of all L^* galaxies that produce radio lobes over a Hubble time, and f^VOL_FILAMENTS is the volume fraction of the mature universe (still well beyond a GZK distance) that is occupied by LSS filaments, i.e. the complement of the cosmic void fraction. For the normalizations adopted in (4), the corresponding intergalactic magnetic field strength is

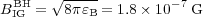(5)

Before describing some first attempts below to detect and measure IGM fields, I now turn to another, though less quantifiable source of intergalactic fields due to star-driven outflows.

4.2 Early dwarf galaxy outflows before z ~ 7 as sources of IGM magnetic fields

Using detailed starburst outflow parameters measured for a mass range of galaxies down to ~ 10⁸ M it is possible to project such data backwards in Cosmic time to z 10, where the ~1000x smaller co-moving volumes might have contained mostly dwarf galaxies, since hierarchical merging into larger galaxies will have taken place mostly after that time. At z 10, each co-expanding "cell" will be more easily filled with star and supernova-driven magnetized winds.

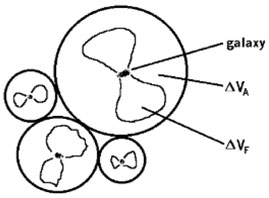Figure 10. A cartoon dwarf galaxy outflow filling of the IGM. VF is the local IGM volume filled by the starburst outflow, and VA the volume available to be filled, in a bubble model representation of intergalactic space (ref. [20]).

As time progresses toward the present epoch, these co-expanding cells will retain their volume filling factor from early times when they were small in absolute size. Model calculations of this kind [20] show two interesting results that persist over a wide range of model parameters: First, the global fraction, [V_F] in Fig. 10, of IGM volume within the galaxy filament zones that is ultimately filled with dwarf galaxy outflow magneto-plasma reaches 20% of [V_A] by the present epoch (z = 0). Second, in a variety of model parameter combinations this substantial filling factor nearly reaches the z = 0 value by z ~ 7 [20].

If the "V_F clouds" were to expand adiabatically as Proper Time proceeds, this collective starburst outflow contribution to <|B_IGM|> within the galaxy filament zones would reach ~ 10^-8 to 10^-9 G by z = 0. However, as Ryu, Vishniac, and others have recently calculated, large scale gravitationally-driven inflow into filaments, galaxies and galaxy groups evolve is accompanied by large scale shearing and turbulence of the IGM gas [21, 22]. These models produce an amplified <|B_IGM|> in galaxy filaments that reaches as high as ~ 10^-7 G. Although these calculated scenarios are more removed from confirming observations, it is interesting that is can they can produce <|B_IGM|> in filaments that are comparable with the supermassive BH - generated IGM fields in equation (5). The implication for UHECR propagation and anisotropy modeling is for a high contrast in <|B_IGM|> between the filaments and voids of the local universe.