4. GALAXY INTERACTIONS

The original concept of galaxies as "island universes" is, of course, the truth, but not the whole truth. While many galaxies do seem to be evolving in isolation there are clear indications that some galaxies are interacting strongly with other galaxies or their larger scale environment. We describe these interactions in the remainder of this section.

4.1. Galaxy Orbits

A consequence of the hierarchical nature of structure formation in a cold dark matter universe is that dark matter halos are built through the merging together of earlier generations of less massive halos. While for a long time numerical simulations indicated that all trace of earlier generations of halos was erased during the merging process (Katz and White 1993; Summers et al. 1995) it was understood on analytical grounds that this was likely a numerical artifact rather than a physical result (Moore et al. 1996a). Beginning in the late 1990's, N-body simulations clearly demonstrated that this was indeed the case (Tormen et al. 1998; Moore et al. 1999; Klypin et al. 1999). Unlike earlier generations of simulations, they found that halos can persist as subhalos within larger halos into which they merge. The current highest resolution simulations of individual halos (Kuhlen et al. 2008; Springel et al. 2008) show almost 300,000 subhalos ¹¹ and even show multiple levels of subclustering (i.e. subhalos within subhalos within subhalos...). Each subhalo may, in principle, have acted as a site of galaxy formation and so may contain a galaxy which becomes a satellite in the host potential.

These subhalos are gravitationally bound to their host halo and, as such, will orbit within it. A subhalo's orbit can take it into regions where interactions affect the properties of any galaxy that it may contain. We begin, therefore, by considering the orbits of subhalos.

At the point of merging, which we will define as the time at which the center of mass of a subhalo-to-be first crosses the virial radius of its future host halo, we expect the orbital parameters (velocities, energy etc.) to be of order unity when expressed in units of the characteristic scales of the host halo. For example, Benson (2005) shows that the radial and tangential velocities of merging subhalos are distributed close to unity when expressed in units of the virial velocity of the host halo (Fig. 4). This distribution of velocities reflects the influence of the host halo (infall in its potential well) but also of the surrounding large scale structure which may have torqued the infalling subhalo.

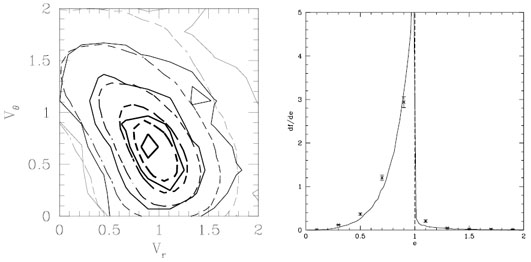Figure 4. Left-hand panel: The joint distribution of radial and tangential velocities of infalling subhalos as they cross the virial radius of their host halo. Velocities are expressed in units of the virial velocity of the host halo. Solid contours indicate measurements from a compilation of N-body simulations while dashed lines indicate the fitting formula of Benson (2005). Contours are drawn at values of d2 f / d V d Vr (the normalized distribution function) of 0.01, 0.1, 0.5, 1.0 and 1.4. Right-hand panel: The corresponding distribution of orbital eccentricities. The distribution peaks close to e = 1 (parabolic orbits) and it is apparent that some subhalos are on unbound (e > 1) orbits. Points indicate the distribution measured from a compilation of N-body simulations, while the solid line indicates the distribution found from the same fitting formula as used in the left-hand panel. Reproduced, with permission, from Benson (2005).

Such orbits will typically carry subhalos into the inner regions of halos. Figure 5 shows the distribution of orbital pericenters assuming an NFW halo with concentration 10 and the orbital parameter distribution of Benson (2005). Most orbits initially reach to 40% of the virial radius, but a significant tail have orbits which carry them into the inner 10% of the halo.

Figure 5. The distribution of orbital pericenters (in units of the virial radius) in an NFW halo having concentration parameter of 10, assuming the orbital parameter distribution of Benson (2005).

4.2. Gravitational Interactions

4.2.1. Mergers

Orbiting subhalos are gravitationally bound to their host halos and, as such, rarely encounter other subhalos at velocities resulting in a bound interaction (Angulo et al. 2008; Somerville et al. 2008b; Wetzel et al. 2008).

To cause gravitationally bound interactions between subhalos and their galaxies typically requires a dissipative process to reduce their orbital energies. Dynamical friction fulfills this role and tends to drag subhalos down towards the center of their host halo, where they may merge with any other galaxy which finds itself there. The classic derivation of dynamical friction acceleration from Chandrasekhar (1943) has been used extensively to estimate dynamical friction timescales within dark matter halos. For example, Lacey and Cole (1993) applied this formula to estimate merging timescales for subhalos in isothermal dark matter halos, finding:

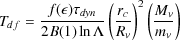(41)

where _dyn = R_v / V_v is the dynamical time of the halo, m_v the virial mass of the orbiting satellite,

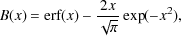(42)

ln ln( r_v V_v² / G m_v) ln(M_v / m_v) is the Coulomb logarithm (treating the satellite as a point mass), f() encapsulates the dependence on the orbital parameters through the quantity = J / J_c(E) where J is the angular momentum of the satellite and J_c(E) is the angular momentum of a circular orbit with the same energy, E, as the actual orbit and r_c is the radius of that circular orbit. Lacey and Cole (1993) found that f() = ^0.78 was a good fit to numerical integrations of orbits experiencing dynamical friction.

Besides the fact the dark matter halos are not isothermal, there are a number of other reasons why this simple approach is inaccurate:

1. Chandrasekhar's derivation assumes an infinite, uniform medium, not a dark matter halo with a radially varying density profile;

2. There is the usual uncertainty in how to define the Coulomb logarithm (e.g. what is the maximum impact parameter for interactions between the satellite and dark matter particles);

3. Subhalos will experience mass loss as they orbit, changing the timescale;

4. The host halo itself is constantly evolving;

5. The host halo is non-spherical and has a non-isotropic velocity dispersion.

While some of these limitations can be overcome (e.g. mass loss can be modeled; Benson et al. 2002a, Taylor and Babul 2004; the Coulomb logarithm can be treated as a parameter to be fit to numerical results; results exists for dynamical friction in anisotropic velocity distributions; Binney 1977b, Benson et al. 2004) others are more problematic. Recently, attempts have been made to find empirical formulae which describe the merging timescale. These usually begin with an expression similar to the one in eqn. (41) but add empirical dependencies on subhalo mass and orbital parameters which are constrained to match results from N-body simulations. Results from such studies (Jiang et al. 2008; Boylan-Kolchin et al. 2008) show that the simple formula in eqn. (41) tends to underestimate the timescale for low mass satellites (probably because it ignores mass loss from such systems) and overestimates the timescale for massive satellites (probably due to a failure of several of the assumptions made in this limit). Alternative fitting formulae have been derived from these studies. For example, Boylan-Kolchin et al. (2008) find

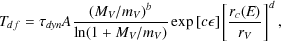(43)

with A = 0.216, b = 1.3, c = 1.9 and d = 1.0 while Jiang et al. (2008) finds

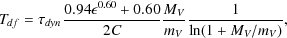(44)

with C = 0.43. A comparison of the Boylan-Kolchin et al. (2008) fit, eqn. (41) and measurements from numerical simulations is shown in Fig. 6.

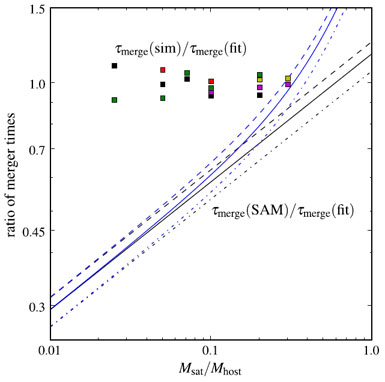Figure 6. A comparison of dynamical friction timescales measured from N-body simulations, merge(sim), with the fitting formula of Boylan-Kolchin et al. (2008), merge(fit), is shown by the colored points, with colors coding for orbital circularity: = 0.33 (red), 0.46 (green), 0.65 (black), 0.78 (magenta) and 1.0 (yellow). Lines compare merge(fit) with the expectation from eqn. (41), labeled merge(SAM). The different color curves correspond to different choices of Coulomb logarithms in eqn. (41): ln(1 + Mv / mv) (black curves) and 1/2 ln(1 + Mv2 / mv2) (blue curves). Reproduced, with permission, from Boylan-Kolchin et al. (2008).

The consequences of merging for the galaxies involved are discussed in Section 5.2.1.

4.2.2. Tidal Destruction

An orbiting subhalo and its galaxy will experience tidal forces which may strip away the outer regions or, in extreme cases, entirely disrupt the galaxy resulting in a stellar stream (as seems to be happening with the Sagittarius dwarf galaxy in orbit around the Milky Way; Belokurov et al. 2006).

In a rotating frame in which an orbiting satellite instantaneously has zero tangential velocity, the effective tidal field felt by the satellite is

(45)

where M_h(r) is the mass enclosed within radius r in the host halo and is the instantaneous angular velocity of the satellite. An estimate of the radius, r_t, in the satellite subhalo/galaxy system beyond which tidal forces become important can be made by equating the tidal force to the self-gravity of the subhalo

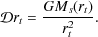(46)

Beyond this tidal radius material becomes unbound from the satellite, forming a stream of dark matter and, potentially, stars which continue to orbit in the host potential.

This simple estimate ignores the fact that particles currently residing in the inner regions of a subhalo may have orbits which carry them out to larger radii where they may be more easily stripped. As such, the degree of tidal mass loss should depend not only on the density profile of the satellite but also on the velocity distribution of the constituent particles. Attempts to account for this find that particles in an orbiting satellite that are on prograde orbits are more easily stripped than those on radial orbits which are in turn more easily stripped than those on retrograde orbits (Read et al. 2006). Additionally, some material will be stripped from within the classical tidal radius, as particles which contribute to the density inside that radius may be on orbits which carry them beyond it. This can lead to more extensive and continuous mass loss as the reduction in the inner potential of the satellite due to this mass loss makes it more susceptible to further tidal stripping. (Kampakoglou and Benson 2007).

4.2.3. Harassment

A less extreme form of tidal interaction arises when tidal forces are not strong enough to actually strip material from a galaxy. The tidal forces can, nevertheless, transfer energy from the orbit to internal motions of stars in the galaxy, effectively heating the galaxy. The generic results of such heating are to cause the galaxy to expand and to destroy cold, ordered structures such as disks (Moore et al. 1996b, 1998; Mayer et al. 2001a, b; Gnedin 2003; Mastropietro et al. 2005a, b). The harassment process works via tidal shocking in which the stars in a galaxy experience a rapidly changing tidal field along its orbit and gain energy in the form of random motions, leading to the system expanding and becoming dynamically hotter. During such tidal shocks, the energy per unit mass of the galaxy changes by (Gnedin 2003)

(47)

where ⟨ r²⟩ is the mean squared radius of the galaxy and

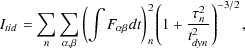(48)

where the sums extend over all n peaks in the density field (i.e. the host halo and any other subhalos that it may contain) and over all components of the tidal tensor

(49)

where is the gravitational potential. Here, _n is the effective duration of the encounter with peak n and t_dyn is the dynamical time at the half-mass radius of the galaxy. The (1 + _n² / t_dyn²)^-3/2 term describes the transition from the impulsive to adiabatic shock regimes (Gnedin and Ostriker 1999; see also Murali and Weinberg 1997a, Murali and Weinberg 1997b, Murali and Weinberg 1997c).

4.3. Hydrodynamical Interactions

While the collisionless dark matter is affected only by gravity the baryonic content of galaxies (and their surrounding atmospheres of gas) can be strongly affected by hydrodyamical forces.

4.3.1. Ram pressure

The orbital motion of a subhalo through the hot atmosphere of a host halo leads to a large ram pressure. The characteristic magnitude of that pressure

(50)

can greatly exceed the binding energy per unit volume of both hot gas in subhalos and interstellar medium (ISM) gas in their galaxies. As such, ram pressure forces may be expected to quite efficiently remove the hot atmospheres of satellite galaxies, a process with several grim aliases including strangulation and starvation, and the ISM of the galaxy.

The first quantification of this process was made by Gunn and Gott (1972) who showed that the ram pressure force could remove material from a galactic disk if it exceeded the gravitational restoring force per unit area which itself cannot exceed

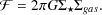(51)

For a disk of mass M_d with gas mass M_g having an exponential surface density profile for both gas and stars with scale length r_d, the gravitational restoring force per unit area is given by (Abadi et al. 1999):

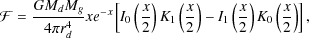(52)

where x = r / r_d and I₀, I₁, K₀ and K₁ are Bessel functions.

The mass loss caused by this ram pressure can, in many cases, be further enhanced by related effects, such as turbulent viscous stripping (Nulsen 1982). This initial estimate has been revised and calibrated more accurately using numerical simulations (Abadi et al. 1999; McCarthy et al. 2008). The process of ram pressure stripping has been incorporated into some semi-analytic models of galaxy formation (Lanzoni et al. 2005; Font et al. 2008) where it plays an important role in mediating the transition of cluster galaxies from the blue cloud of star-forming galaxies to the red sequence of passively evolving galaxies (Font et al. 2008).

¹¹ This is a lower limit due to the limited resolution of the simulations. The earliest generations of cold dark matter halos may have masses as low as 10^-12 M (depending on the particle nature of the dark matter) while state of the art simulations resolve only halos with masses greater than around 10⁵ M. The ability of even lower mass halos to survive is a subject of much debate (Berezinsky et al. 2006; Zhao et al. 2007; Goerdt et al. 2007; Angus and Zhao 2007; Elahi et al. 2009). Back.