It has been stated that the Tully-Fisher (TF) relation is the ``workhorse'' of peculiar velocity surveys. One can anticipate a time in the not so distant future when more accurate techniques may supplant it, but for the next few years at least, the TF relation is likely to remain the most widely used distance indicator in cosmic velocity studies. Its role in such studies to date has been, in fact, too large to be reviewed here, and interested readers are referred to Strauss & Willick (1995) Section 7. Several recent developments are discussed in Section 8.
The TF relation is one of the most fundamental properties of spiral galaxies. It is the empirical statement of an approximately power-law relation between luminosity and rotation velocity. Specifically, it is found that
or, using the logarithmic formulation preferred by working astronomers,
In equation (4)
M = -2.5 log(L) + const. is the absolute magnitude, and
the velocity width parameter
An important fact, not always sufficiently appreciated,
is that the power law exponent
The numerical value of the TF zero point A has no absolute
significance in itself, reflecting mainly the photometric
system in which the TF measurements are done.
Absolute magnitudes measured
in different bandpasses can differ numerically by a few magnitudes
for a given object.
Clearly, this must have no meaning for distance measurements,
and it is the zero point that absorbs such differences.
For any given measurement system, however, the value of
A is highly significant. To see this,
consider how the TF relation is used to
infer distances and peculiar velocities. Given a measured apparent
magnitude m and width parameter
The TF relation has a rich history (cf.
Bottinelli et
al. 1983)
which can not be discussed in any detail here.
Its discovery is generally
credited to
Tully & Fisher (1977),
who were the first to suggest
a linear correlation between absolute magnitude and log rotation
speed. Until the late 1980s, the velocity widths used in TF studies
were generally obtained from analysis of the 21 cm line profiles,
rather than from direct measurements of rotation
curves. Thus, the
TF relation was (and to some extent remains) closely associated
with 21 cm radio astronomy. There is no inherent connection,
however, between the TF relation and the 21 cm line.
The TF relation is also closely associated
in the minds of many with infrared magnitudes. This is largely
due to the pioneering H-band (1.6 µm) TF work of a group
headed by the late Marc Aaronson in the late 1970s through the
mid-1980s (e.g.,
Aaronson et al. 1980,
1982,
1986).
This group argued that the infrared was better than the optical
for TF purposes because infrared magnitudes
are less subject to internal and Galactic extinction
(Section 3.2), and
because they are sensitive mainly to
the old stellar population that best traces mass.
While these arguments are
true at some level, work over the last decade has demonstrated
that CCD imaging photometry in red passbands (e.g., R or I)
results in TF relations that, empirically, work as well or better
than the H-band version
(Pierce & Tully 1988,
1992;
Willick 1991;
Han 1992;
Courteau 1992;
Bernstein et al. 1994;
Willick et al. 1995,
1996).
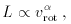
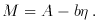
log(2vrot) - 2.5,
where vrot is expressed
in km s-1, is a useful dimensionless measure of rotation velocity.
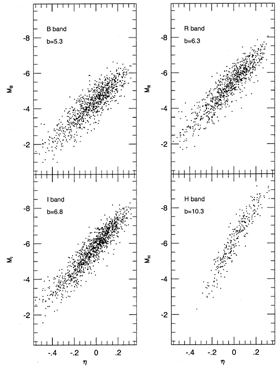
Figure 2. TF relations in four bandpasses.
The absolute magnitudes are given in units
such that d = 100.2(m-M) is the galaxy
distance in km s-1. Adapted from
Strauss & Willick
(1995).
does not have a unique value.
The details of both the photometric and spectroscopic
measurements affect it. A typical result found in
contemporary studies is 
3. The corresponding value
of the ``TF slope,'' b, is ~ 7.5. However, slight changes
in the details of measurement can result in significant changes
in b. This is illustrated in Figure 2, in which
TF relations in four bandpasses are plotted. The optical
bandpasses (B, R, and I) all represent data from
the sample of
Mathewson et
al. (1992).
In each case the slope
is < 7. This is because Mathewson et al. defined their
velocity widths rather differently than most observers, with
the result that small velocity widths are made smaller still relative
to other width measurement systems, while large velocity widths
are unchanged in comparison with other systems. If one were
to transform the Mathewson et al. widths to those of standard
systems, one would obtain and I-band TF slope of ~ 7.7,
comparable to other I-band samples
(Willick et al. 1997).
Note, however, that the slope increases steadily from B to I.
This reflects a general trend of increasing TF slope toward
larger wavelengths, which was noted over a decade ago
(Bottinelli et
al. 1983).
The H-band data come from the compilation of
Aaronson et al. (1982),
as reanalyzed by
Tormen & Burstein
(1995) and
Willick et al. (1996).
The H-band TF slope is considerably greater than its optical
counterparts. In part this reflects the trend just noted. However, to a
greater extent it is
due to the relatively small apertures within which the H-band
photometry is done: the TF slope increases as the photometric
aperture size is decreased
(Willick 1991).
Infrared TF samples in which total magnitudes
were measured exhibit TF slopes not much in excess of the optical values
(Bernstein et
al. 1994).
, one infers the
distance modulus to the galaxy as µ = m - (A - b). The
corresponding distance d 
100.2µ. It follows that
an error 
A in the TF
zero point thus corresponds to a
fractional distance error f = 10-0.2A. A Hubble constant
inferred from such distances will then be off by a factor
f-1. For peculiar velocities, calibration of the
TF relation consists in choosing A such that d 100.2[m - (A - b )]
gives a galaxy's distance in km s-1. There is no requirement that it
yield the distance in Mpc. Nonetheless, as mentioned above,
zero point calibration errors are still possible. Errors in
A produce distances in km s-1 that
differ by a fraction f from the true Hubble velocity, with resultant
peculiar velocity errors vp = -fd.