


In this section I review models for the observations, as well as several problems and their possible solutions. I begin with the problem of confining the jet. Next I consider models for the radio-to-X-ray spectrum and problems posed by the short lifetimes of the optical (and X-ray) emitting electrons. Finally a possible model for the jet kinematics is sketched out.
A. Confinement of the Jet
If we assume the jet is a steady-state structure, then some external pressure must act to counter the jet's internal pressure, in order to confine the jet and maintain its narrow appearance. That is, a static pressure balance must exist between the jet and external medium. It is possible to estimate both the pressure in the emitting regions and the external pressure, and test for such a balance. The minimum pressure required to provide the observed synchrotron luminosity may be estimated from the usual synchrotron calculations (e.g., Moffet 1975). The resulting pressure is proportional to the observables as
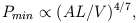
|
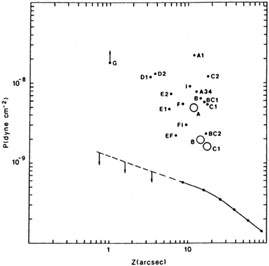
where L is the luminosity, V is the source volume, and A is a weak function of the spectral shape. The largest uncertainty in this calculation is perhaps the source volume. For example, if we assume the emissivity is uniform across the jet's radius we obtain a pressure Pmin = 5 x 10-9 dyne cm-2 for knot A (Owen, Hardee, and Bignell 1980), while a larger pressure Pmin = 2 x 10-8 dyne cm-2 results if we assume the emission originates in a thin layer near the jet's surface (OHC89; Fig. 20). The external pressure maybe estimated from cooling accretion flow models fit to X-ray observations of the thermal emission (e.g., Lea, Mushotzky, and Holt 1982; Schreier, Gorenstein, and Feigelson 1982; White and Sarazin 1988). At the distance from the nucleus of knot A, typical estimates of the external pressure are Pext ~ 6 X 10-10 dyne cm-2. This is much less than either estimate of the minimum pressure in knot A, and suggests a problem in confining the jet and explaining its narrow appearance. A similar situation exists for most other knots in the jet.
|
Figure 20. Comparison of minimum internal pressures in the knots with external pressures derived from models of X-ray observations. Dots show internal pressures derived by OHC89, while large circles show values derived from largest source volume consistent with the radio observations. Line shows estimated external pressure. Original Figure from OHC89. |
One solution is that our initial assumption of static pressure balance is incorrect. As we have discussed in Section 2.A., it seems likely that knots A and C are shock waves, and hence the emitting material may be in a region of transient expansion, rather than static pressure balance. Another solution is that the jet is freely expanding and confined only by its own inertia, in which case there is again no pressure balance. This possibility seems most applicable to the inner jet (region including knots D, E, F, and I), which appears to be an expanding cone, and may represent a high Mach number flow (c.f. Sec. 2.A.). These two solutions together would appear to resolve the confinement problem everywhere in the jet except knot B (Biretta, Owen, and Hardee 1983). Knot B appears as a non-expanding cylinder, and contains no obvious shock-like structures. Here it may be necessary to invoke magnetic confinement, where magnetic field lines wrapped around the jet serve to amplify the external pressure, and thereby confine the jet (Benford 1978). This is not necessarily inconsistent with the polarization observations (OHC89) showing the magnetic field to be oriented along the jet in knot B, since the confining field could lie mostly outside the visible jet.
In summary, confinement of the jet appears to be a serious problem only in the region of knot B, and perhaps magnetic effects play a role here.