


The cosmic microwave background radiation (CMBR) is the dominant radiation field in the Universe, and one of the most powerful cosmological tools that has yet been found. 25 years after its discovery by Penzias & Wilson (1965) (2) much is now known about the properties of the radiation (see the recent review by Partridge 1995), and a vigorous community studies the CMBR to extract all the cosmological and astrophysical data that it carries.
Within a few years of the discovery of the CMBR, it was established
the radiation field is close to isotropic, with a spectrum
characterized by a single temperature, Trad  2.7 K.
The specific intensity of the radiation is therefore close to
2.7 K.
The specific intensity of the radiation is therefore close to
which corresponds to a peak brightness
Imax ~ 3.7 x 10-18 W m-2
Hz-1 sr-1 at
required to close the Universe. In these equations, h is Planck's
constant, c is the speed of light,
Although specific small parts of the sky (stars, radio sources, and
so on) are brighter than the CMBR, overall the CMBR constitutes the
major electromagnetic radiation field in the Universe and contributes
about 60 per cent of the relativistic energy density (the other 40 per
cent being provided by the neutrinos, assumed to be massless
here). The integrated brightness of the sky in the CMBR is not small,
and a comparison with a bright radio source may be useful. Cygnus A is
one of the brightest extragalactic radio sources at low
frequencies. A comparison of the relative brightness of Cygnus A and
the CMBR, as observed by a telescope with a 1 square degree beam, is
shown in Figure 1. It can be seen that the CMBR easily
dominates over a wide range of frequencies above 10 GHz. It is not signal
strength that makes measuring the intensity of the CMBR difficult, but
rather the problem of making absolute measurements,
since the CMBR is present in all directions with almost
equal intensity.
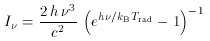
 max ~ 160 GHz, a photon
density n
max ~ 160 GHz, a photon
density n ~ 4 x 108 photons m-3, and an
energy density u
~ 4 x 108 photons m-3, and an
energy density u ~ 4 x 10-14 J m-3, which
can also be expressed as a mass density
~ 4 x 10-14 J m-3, which
can also be expressed as a mass density

 ~ 5 x
10-31 kg m-3, much less than the critical density
~ 5 x
10-31 kg m-3, much less than the critical density

 is the frequency,
kB is the Boltzmann constant, G is the
gravitational constant, and
h100 = H0/100 km s-1
Mpc-1 is a dimensionless
measure of the value of the Hubble constant,
H0. Recent estimates
give 0.5
is the frequency,
kB is the Boltzmann constant, G is the
gravitational constant, and
h100 = H0/100 km s-1
Mpc-1 is a dimensionless
measure of the value of the Hubble constant,
H0. Recent estimates
give 0.5  h100
h100  0.8 (e.g.,
Sandage et al.
1996;
Falco et al. 1997;
Sandage & Tammann 1997;
Freedman et al. 1997).
0.8 (e.g.,
Sandage et al.
1996;
Falco et al. 1997;
Sandage & Tammann 1997;
Freedman et al. 1997).
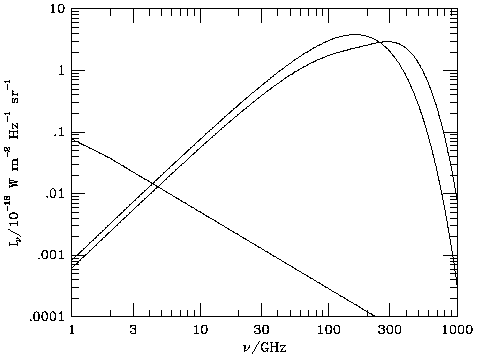
Figure 1.The spectrum of the microwave background
radiation, and the microwave background radiation after passage
through an (exaggerated) scattering atmosphere with y = 0.1 and

 = 0.05 (as defined in
Sections 3 and 6),
compared with the integrated emission from the
bright radio source Cygnus A as observed by a telescope with solid angle
= 0.05 (as defined in
Sections 3 and 6),
compared with the integrated emission from the
bright radio source Cygnus A as observed by a telescope with solid angle
 beam = 1 square
deg. Note that the microwave background
radiation dominates at high frequencies. Scattering (the
Sunyaev-Zel'dovich effect) causes a fractional decrease in the
low-frequency intensity of the CMBR that is proportional
to y. The location of the cross-over point, where the scattered CMBR
and the unscattered CMBR have equal brightness,
is a measure of
beam = 1 square
deg. Note that the microwave background
radiation dominates at high frequencies. Scattering (the
Sunyaev-Zel'dovich effect) causes a fractional decrease in the
low-frequency intensity of the CMBR that is proportional
to y. The location of the cross-over point, where the scattered CMBR
and the unscattered CMBR have equal brightness,
is a measure of 
 . This scattered
spectrum was calculated
using the Kompaneets formula (59), rather than
the relativistic results (eq. 51) of
Rephaeli (1995a),
and hence is only accurate for
low cluster gas temperatures (see Sec. 3.3),
although the difference is imperceptible in this figure.
. This scattered
spectrum was calculated
using the Kompaneets formula (59), rather than
the relativistic results (eq. 51) of
Rephaeli (1995a),
and hence is only accurate for
low cluster gas temperatures (see Sec. 3.3),
although the difference is imperceptible in this figure.
2 Low-significance indications of excess
microwave radiation had been reported earlier (e.g.,
Shmaonov 1957;
Ohm 1961),
but not
attributed to cosmic processes or lost in the
error estimates. In hindsight a universal radiation field could
have been deduced from the excitation of some interstellar molecules
(Thaddeus 1972).
Back.