Annu. Rev. Astron. Astrophys. 1992. 30: 499-542 Copyright © 1992 by Annual Reviews. All rights reserved

3.2 The Age of the Universe

By a trivial change of variables in Equation 9, from a to z and from to t, we obtain an integral that relates redshift z₁ to lookback time from the present,

16.

This integral can be solved analytically in some special cases, e.g. when = 0 (Kolb & Turner 1990, equations 3.22-3.25; Sandage 1961a) or when _tot = 1 (Weinberg 1989). In general, it can be calculated numerically without difficulty. By inspection of the integrand, one sees that at fixed _M, increasing lengthens the lookback time to any redshift. Eliminating in favor of _tot, one likewise finds that at fixed _tot, the lookback time to any z lengthens for decreasing _M. Figure 3 shows the lookback time as a function of redshift for the five models A-E.

Figure 3. Lookback time as a function of redshift for the five models A-E. Even at moderate redshifts (z 1), the -dominated models separate cleanly from the open models. However, the absolute differences are small.

The integral in Equation 16 goes to a finite limit, the age of the universe, as z₁ -> . Kolb & Turner (1990) give analytic formulas for the special cases = 0 and _tot = 1 (see also Sandage 1961a). Figure 4 shows the results of numerical integration for general cases with , _tot in the same range that was shown in Figure 1. One sees that if _M, is bounded to a plausible range, then the age of the universe is between about 0.5 and 2 Hubble times. In Section 4.2 we will compare these ages to observational constraints.

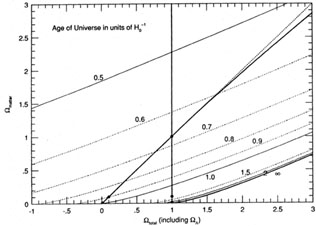
Figure 4. Contours of the age of the universe are shown in the M, tot) plane. Away from the infinity line, the contours are close to straight lines, and Equation 17 is a good analytic approximation.

Because the contours in Figure 4 are not too different from lines of constant slope, one can readily write a simple approximation that is valid to within a few percent in the range 0 < _M 1, 0 < _tot 1,and serviceable anywhere away from the loitering line;

17.

where

18.

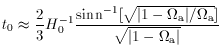
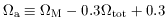
and ``sinn^-1'' is defined as sinh^-1 if _a 1 (the usual case) and as sin^-1 if _a > 1. (In fact, Equation 17 is the exact result when _tot = 1.)