If progenitors of quasars or protogalaxies form at
high redshifts (larger than 10), then the millimeter domain
is the best place to detect them
(Loeb 1993;
Braine 1995).
Both continuum and
line emission could be detected, provided enough sensitivity,
with about 10 times more collecting surface as present
ones (either with a single dish, as the GBT-100m of NRAO,
or with the ALMA interferometer).
As was clear in the previous sections, the detection of
the submm continuum emission from actively star-forming
objects at high redshifts is much easier than the CO line detection.
The line emission does not have such a negative
K-correction, since in the low frequency domain, the
flux of the successive lines increases roughly as
2
(optically thick domain), instead of
4 for the continuum.
Nevertheless the line emission is essential to study
the nature of the object (the AGN-starburst connection
for instance), and deduce more physics (kinematics,
abundances, excitation, etc..). Given the gas and dust
temperatures, the maximum flux is always reached at
much lower frequencies than in the continuum, since the lines
always reflect the energy difference between two levels;
this is an advantage, given the largest atmospheric opacity at high
frequencies.
4.1. Predicted Line and Continuum Fluxes
The best tracer of molecular gas at large-scale is the CO molecule, the most abundant after H2. All other molecules will give weaker signals. The fine-structure CII line at 158 µm, formed in PDR at the border of molecular clouds, is also thought to be a useful tool for proto-galaxies or proto-quasars (Loeb 1993), but it has revealed disapointing in starbursts, or compact objects, due to optical thickness or inefficiency of gas heating (e.g. Malhotra et al. 1997): the LCII / LFIR ratio decreases as LFIR / LB increases.
To model high-redshift starburst objects, let us
extrapolate the properties of more local ones:
the active region is generally confined to a compact nuclear
disk, sub-kpc in size
(Scoville et al. 1997a,
Solomon et al. 1990,
1997).
The gas is much denser here than in average over a normal galaxy, of the order
of 104 cm-3, with clumps at least of
106 cm-3
to explain the data on high density tracers (HCN, CS..);
large gas masses can pile up in the center, due to
torques exerted in galaxy interactions and mergers (e.g.
Barnes &
Hernquist 1992,
1996).
To schematize, the ISM maybe modelled by two
density and temperature components, at 30 and 90K
(cf. Combes et
al. 1999).
The total molecular mass considered will be 6 x 1010
M
and the
average column density N(H2) of 1024 cm-2,
typical of the Orion cloud center.
Going towards high redshift (z > 9), the temperature of
the cosmic background Tbg becomes of the same order
as the interstellar dust temperature, and the excitation of
the gas by the background radiation competes with that
of gas collisions. It might then appear easier to detect the lines
(Silk & Spaans
1997), but this is not the case
when every effect is taken into account. To have an idea
of the increase of the dust temperature with z, the simplest
assumption is to consider the same heating power due
to the starburst. At a stationary state, the dust must then radiate
the same energy in the far-infrared that it receives from
the stars, and this is proportional to the quantity
Tdust6 - Tbg6,
if the dust is optically thin, and its opacity varies in
, with
= 2. Keeping this
quantity constant means that the energy re-radiated by the dust,
proportional to Tdust6, is always equal to
the energy it received from the cosmic background,
proportional to Tbg6, plus the constant
energy flux coming from the stars. Since
can also be equal to
1 or 1.5, or the dust be optically thick, we have also
considered the possibility of keeping
Tdust4 - Tbg4
constant; this does not change fundamentally the results.
Computing the populations of the CO rotational levels with an LVG code, and in the case of the two component models described earlier, the predictions of the line and continuum intensities as a function of redshift and frequencies are plotted in Fig. 4.
|
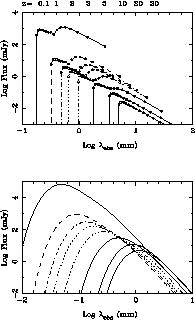
Figure 4. Expected flux for the two-component cloud model, for various redshifts z = 0.1, 1, 2, 3, 5, 10, 20, 30, and q0 = 0.5. Top are the CO lines, materialised each by a circle (they are joined by a line only to guide the eye). Bottom is the continuum emission from dust. It has been assumed here that Tdust6 - Tbg6 is conserved (from Combes et al. 1999). |
When comparing these predictions with the present instrumental sensitivities, it appears that the continuum is detectable at any redshift already, for an ultra-luminous source, while the line emission has to await the order of magnitude increase that will be provided by the next generation in the mm and sub-mm domains. The recent reported detections (cf. Table 1) have been possible because of gravitational lens magnifications (or maybe for 1-2 cases, an exceptional object).