


Giant elliptical galaxies occupy only a small portion of the
three-dimensional parameter space defined by the central
velocity dispersion ( 0), effective surface brightness
(Ie) and effective radius (re). The
manifold occupied by ellipticals is most simply represented as a scaling law
0), effective surface brightness
(Ie) and effective radius (re). The
manifold occupied by ellipticals is most simply represented as a scaling law
with A
Several attempts have been made to assess whether dE galaxies lie on
the fundamental plane defined by the giants. The task is complicated by
the great difficulty in measuring velocity dispersions for faint,
low-surface-brightness galaxies. The faint end of the
luminosity function is represented by Local Group companions, while the
brighter dE's for which velocity dispersions have been measured are
mostly members of the Virgo cluster. These latter samples are far from
complete, and are biased toward high-surface-brightness
objects
(Bender and Nieto
1990;
Peterson and
Caldwell 1993).
Furthermore, the prominent nuclei in many of the
brighter dE's may be distinct dynamical entities, so using
central velocity dispersions may not be sensible if correlations
of global parameters are sought.
Nieto et al. (1990)
presented the first attempt to extend the fundamental
plane to low galactic mass. Their analysis suggested that dE's and
also globular clusters fall near or within the fundamental plane,
albeit with more scatter than expected from the measurement errors.
More recent analysis suggests that dE's do not follow the
giant-elliptical scaling relations
(Bender et al. 1992;
de Carvalho and
Djorgovsky 1992;
Peterson and
Caldwell 1993).
Fig. 4 shows the distribution of
low-luminosity ellipticals (not all of the diffuse kind) along one
projection of the fundamental plane. The local dE's evidently depart
from the giant E fundamental plane. The two sequences appear to
intersect at MB
Dwarf ellipticals appear to define more nearly a one-parameter family,
when L, re, Ie, and
The analysis of the existing samples suggests correlations that are in
reasonable agreement with the predictions of Dekel and Silk (1986).
Specifically, while the fundamental-plane relation for
giant ellipticals implies M / L 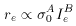
 1.4 and
B
1.4 and
B  -0.9
(Kormendy and Djorgovsky 1989;
Bender et al. 1992).
Simple arguments from the virial theorem and the above scaling relation
suggest that M / L
-0.9
(Kormendy and Djorgovsky 1989;
Bender et al. 1992).
Simple arguments from the virial theorem and the above scaling relation
suggest that M / L  M1/6
M1/6  L1/5
(Dressler et
al. 1987).
Properties of the stellar populations appear to
be closely related to structure and dynamics; tight relations also
exist between re, Ie and color or
metallicity
(de Carvalho and
Djorgovsky 1989).
While the existence of a ``fundamental plane'' for elliptical galaxies
provides important clues about the formation of these galaxies, the
implications of these clues are currently understood only at a
qualitative level
(Kormendy and
Djorgovsky 1989;
Bender et al. 1992).
L1/5
(Dressler et
al. 1987).
Properties of the stellar populations appear to
be closely related to structure and dynamics; tight relations also
exist between re, Ie and color or
metallicity
(de Carvalho and
Djorgovsky 1989).
While the existence of a ``fundamental plane'' for elliptical galaxies
provides important clues about the formation of these galaxies, the
implications of these clues are currently understood only at a
qualitative level
(Kormendy and
Djorgovsky 1989;
Bender et al. 1992).
 -17, in the regime of the brighter cluster
dE's. However, the situation is rendered ambiguous by the large
scatter in the dE measurements, and by an apparent inconsistency
between the two largest datasets.
Bender and Nieto
(1990)
measured
-17, in the regime of the brighter cluster
dE's. However, the situation is rendered ambiguous by the large
scatter in the dE measurements, and by an apparent inconsistency
between the two largest datasets.
Bender and Nieto
(1990)
measured  for
seven low-luminosity ellipticals. However their sample was manifestly
biased toward high-surface-brightness galaxies, and interacting ones at
that. Only two of their galaxies fall on the canonical dE sequence,
and these had central velocity dispersions of 65 ± 4 and 52 ± 6
km s-1.
Peterson and
Caldwell (1993)
measured eight additional dE's, all but one of the nucleated
variety. They found 16.4 <
for
seven low-luminosity ellipticals. However their sample was manifestly
biased toward high-surface-brightness galaxies, and interacting ones at
that. Only two of their galaxies fall on the canonical dE sequence,
and these had central velocity dispersions of 65 ± 4 and 52 ± 6
km s-1.
Peterson and
Caldwell (1993)
measured eight additional dE's, all but one of the nucleated
variety. They found 16.4 <  < 39
km s-1. It is not clear whether the discrepancy with
Bender and Nieto
(1990)
is due to the different types of galaxies in the samples, the different
spectral resolutions, or different apertures. It is clear that
measurements are needed for a complete sample that is unbiased in
surface-brightness to determine the true relations for dE's between
structure and velocity dispersion. Measurements for cluster dE's with
absolute magnitudes fainter than -15, while exceedingly difficult to
obtain, are important for testing whether cluster dE's and local dE's
really are the same type of object.
< 39
km s-1. It is not clear whether the discrepancy with
Bender and Nieto
(1990)
is due to the different types of galaxies in the samples, the different
spectral resolutions, or different apertures. It is clear that
measurements are needed for a complete sample that is unbiased in
surface-brightness to determine the true relations for dE's between
structure and velocity dispersion. Measurements for cluster dE's with
absolute magnitudes fainter than -15, while exceedingly difficult to
obtain, are important for testing whether cluster dE's and local dE's
really are the same type of object.

Figure 4. One projection of the fundamental plane of elliptical
galaxies. Data from
Dressler et
al. (1987)
for Virgo and
Fornax cluster
ellipticals are shown as x's. Galaxies surveyed by
Bender and Nieto
(1990)
are shown as circles, while those of
Peterson and
Caldwell (1993)
are squares. Local group galaxies are shown by their
abbreviations. Data
for the Local Group galaxies come from the compilation by
Bender et al. (1992),
with the exception of Sextans, for which we have used the values in
Peterson and
Caldwell (1993).
The solid line is the least
square fit to the Dressler et al. Virgo-cluster data. The dotted
line shows the trend for M / L  L-0.37 predicted by
Dekel and Silk
(1986).
At fixed luminosity, the velocity dispersions reported by
Peterson and Caldwell are uniformly lower than those reported by Bender
and Neito, rendering it difficult to tell which sequence the cluster
dE's actually inhabit.
L-0.37 predicted by
Dekel and Silk
(1986).
At fixed luminosity, the velocity dispersions reported by
Peterson and Caldwell are uniformly lower than those reported by Bender
and Neito, rendering it difficult to tell which sequence the cluster
dE's actually inhabit.
 0 are considered; the
scatter in the surface-brightness-magnitude relation
(Sect. 2.2.2) is not
significantly reduced if surface-brightness is replaced by a linear
combination of surface-brightness and log
0 are considered; the
scatter in the surface-brightness-magnitude relation
(Sect. 2.2.2) is not
significantly reduced if surface-brightness is replaced by a linear
combination of surface-brightness and log  0. However, the paucity
of velocity dispersions for galaxies between MB = -16 and
MB = -11 renders this to a certain extent an exercise
in small-number statistics. The statistics can be improved by
substituting color for velocity dispersion. For Virgo and
Fornax cluster dE's, there is a small improvement if
color is added as an additional parameter, but large scatter remains.
The rather heterogeneous samples of galaxies for which colors
have been measured seriously compromises the analysis of scaling
relations and tests for the number of independent parameters.
While giant E samples are reasonably free from selection effects
based on color and surface-brightness, dE samples are greatly
affected by such selection effects. Low surface-brightness galaxies
are typically detected on blue-sensitive emulsions; hence the
discovery technique will tend to find blue galaxies and miss red
ones if there is a spread in color at fixed bolometric surface-brightness.
Furthermore, photometry samples in the literature are a mix
of bright, relatively high-surface brightness dE's
chosen for ease in getting photometry
(Bothun and
Caldwell 1984;
Caldwell and
Bothun 1987),
and extremely low surface-brightness dE's chosen because they are interesting
(Impey et al. 1988;
Bothun et al. 1991).
0. However, the paucity
of velocity dispersions for galaxies between MB = -16 and
MB = -11 renders this to a certain extent an exercise
in small-number statistics. The statistics can be improved by
substituting color for velocity dispersion. For Virgo and
Fornax cluster dE's, there is a small improvement if
color is added as an additional parameter, but large scatter remains.
The rather heterogeneous samples of galaxies for which colors
have been measured seriously compromises the analysis of scaling
relations and tests for the number of independent parameters.
While giant E samples are reasonably free from selection effects
based on color and surface-brightness, dE samples are greatly
affected by such selection effects. Low surface-brightness galaxies
are typically detected on blue-sensitive emulsions; hence the
discovery technique will tend to find blue galaxies and miss red
ones if there is a spread in color at fixed bolometric surface-brightness.
Furthermore, photometry samples in the literature are a mix
of bright, relatively high-surface brightness dE's
chosen for ease in getting photometry
(Bothun and
Caldwell 1984;
Caldwell and
Bothun 1987),
and extremely low surface-brightness dE's chosen because they are interesting
(Impey et al. 1988;
Bothun et al. 1991).
 L1/5, the relation for
dwarfs is M / L
L1/5, the relation for
dwarfs is M / L  L-0.4, close to the M / L
L-0.4, close to the M / L  L-0.37
relation expected for mass-loss within a dominant dark halo
(Dekel and Silk
1986).
However, it must be noted that the observed relation has
large scatter (and involves many assumptions) and there is a hint of
a correlation of M / L for local dE's with position in the outer-galaxy
halo (see below).
L-0.37
relation expected for mass-loss within a dominant dark halo
(Dekel and Silk
1986).
However, it must be noted that the observed relation has
large scatter (and involves many assumptions) and there is a hint of
a correlation of M / L for local dE's with position in the outer-galaxy
halo (see below).