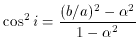
where i is the inclination, (b/a) is the observed axial
ratio, and  is the axial ratio for an edge-on system. We can also allow
is the axial ratio for an edge-on system. We can also allow
 to
be a function of morphological type (e.g.,
Heidmann et al. 1972;
Bottinelli et
al. 1983;
Fouqué et
al. 1990).
This approach assumes that galaxies have circular isophotes, to which there
are clearly counter examples (e.g., M101). Fortunately, the most severe cases
are obvious
and can be excluded. Less obvious cases increase the uncertainty in i,
particularly at low i, but the effect due to variations in
to
be a function of morphological type (e.g.,
Heidmann et al. 1972;
Bottinelli et
al. 1983;
Fouqué et
al. 1990).
This approach assumes that galaxies have circular isophotes, to which there
are clearly counter examples (e.g., M101). Fortunately, the most severe cases
are obvious
and can be excluded. Less obvious cases increase the uncertainty in i,
particularly at low i, but the effect due to variations in  may be
equally important. Historically, axial ratios have
been estimated by eye using the Sky Survey or larger scale plates. This is
an uncertain procedure, particularly when applied to systems
whose inner (higher surface brightness) isophotes are not circular
(cf.
van den Bergh 1988).
Pierce (1988) and
Pierce and Tully
(1988)
have advocated the use of ellipse fitting
to CCD images in order to estimate axial ratios and hence inclinations.
They found that deep images reveal an apparent underlying old-disk
population in dwarf systems which can be used to improve the accuracy of the
inclination estimates for these systems.
Even for bright galaxies, fitting ellipses to
isophotes is more quantitative and objective than are eye estimates
using photographic plates. Nevertheless, some personal judgement is still
required as significant variations in ellipticity with radius are often
present in typical galaxies.
may be
equally important. Historically, axial ratios have
been estimated by eye using the Sky Survey or larger scale plates. This is
an uncertain procedure, particularly when applied to systems
whose inner (higher surface brightness) isophotes are not circular
(cf.
van den Bergh 1988).
Pierce (1988) and
Pierce and Tully
(1988)
have advocated the use of ellipse fitting
to CCD images in order to estimate axial ratios and hence inclinations.
They found that deep images reveal an apparent underlying old-disk
population in dwarf systems which can be used to improve the accuracy of the
inclination estimates for these systems.
Even for bright galaxies, fitting ellipses to
isophotes is more quantitative and objective than are eye estimates
using photographic plates. Nevertheless, some personal judgement is still
required as significant variations in ellipticity with radius are often
present in typical galaxies.
An alternative, and promising technique makes use of the two
dimensional velocity field of the galaxy to constrain its inclination.
This approach requires an extensive map of the velocity field of the
galaxy, using either Fabry-Perot imaging at H or aperture
synthesis techniques at 21-cm. While this requires considerably more
effort, the technique offers real promise for improving inclination
estimates. In this approach the gas is assumed to be rotating in
circular motion and the velocity field defines the inclination.
However, the gas is also subject to noncircular
motions originating from non-axisymmetric mass distributions
(e.g., bars, ovals, spiral
structure), and so inclination estimates will be somewhat model
dependent, although independent of the photometric estimates.
Work currently underway by
Schommer et
al. (1989)
should clarify
some of these concerns and provide sufficient data to access the
technique more fully. Initial results indicate good agreement between
the kinematic and photometric inclination estimates.
or aperture
synthesis techniques at 21-cm. While this requires considerably more
effort, the technique offers real promise for improving inclination
estimates. In this approach the gas is assumed to be rotating in
circular motion and the velocity field defines the inclination.
However, the gas is also subject to noncircular
motions originating from non-axisymmetric mass distributions
(e.g., bars, ovals, spiral
structure), and so inclination estimates will be somewhat model
dependent, although independent of the photometric estimates.
Work currently underway by
Schommer et
al. (1989)
should clarify
some of these concerns and provide sufficient data to access the
technique more fully. Initial results indicate good agreement between
the kinematic and photometric inclination estimates.


