| © CAMBRIDGE UNIVERSITY PRESS 1999
| |
1.3.1 Doppler Shift
If a source of light moves with velocity v relative to the observer so
that the velocity vector makes an angle 
with respect to the radial
vector from the observer to the source, the spectral shift of the
light from the source measured by the observer will be given by
where v = |v| and c is the speed of light. For
small velocities, this
formula reduces to the Newtonian limit z = v / c for
radially outward motion.
For stellar motion in our galaxy it is common to find small Doppler
shifts both positive and negative and these are interpreted in terms
of stars moving away from or towards us. James Terrell in 1966
interpreted the quasar redshifts as arising from fast ejection of
quasars from the galactic centre. The problem with this concept was
that it endowed the galactic centre with violent activity, which was
not consistent with the undisrupted motions of stars in the area. This
idea was taken further by Hoyle and Burbidge (1966), who suggested
that, unlike the case for our galaxy, ejection from a galactic nucleus
that shows violent activity would appear normal. in particular, they
suggested NGC 5128 as a likely site for ejection.
The Doppler hypothesis thus delinks redshift from distance: that is,
a large redshift does not imply that the source is very distant. This
eases the energy budget of a typical source. However, if several such
sources are to be ejected from a single site (such as NGC 5128) the
energetics of that site becomes problematical. Moreover, there is the
problem of blueshifts.
The problem was first highlighted by P. Strittmatter in 1966
(unpublished). A site of explosion will eject quasars in all
directions. Thus, unless the explosion occurred a long time ago, some
quasars would still be travelling towards the observer and these
should show blueshifts. Moreover, these quasars would appear brighter
due to the blueshift effect and would tend to dominate in a sample
that is complete with respect to all quasars brighter than a specified
flux level. Detailed calculations based on isotropic ejection of all
such quasars show that the ratio of blueshifted (N-)
quasars to redshifted (N+) quasars in a flux-limited
sample is
where zm is the maximum redshift in the sample, and
We will return to this problem in Chapter 15. For the time being we
leave the topic of Doppler shifts as it was perceived in the early
sixties, and move on to the next alternative.
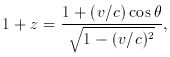
is the spectral
index of a typical source. Thus, for a maximum redshift of 2, say, and
a spectral index unity, we may expect 81 times as many blueshifted
sources as redshifted ones!