4. Dark energy scalar field

At the time of writing the popular picture for dark energy is a classical scalar field with a self-interaction potential V() that is shallow enough that the field energy density decreases with the expansion of the universe more slowly than the energy density in matter. This idea grew in part out of the inflation scenario, in part from ideas from particle physics. Early examples are Weiss (1987) and Wetterich (1988). ⁽⁵⁰⁾ The former considers a quadratic potential with an ultralight effective mass, an idea that reappears in Frieman et al. (1995). The latter considers the time variation of the dark energy density in the case of the Lucchin and Matarrese (1985a) exponential self-interaction potential (Eq. [38]). ⁽⁵¹⁾

In the exponential potential model the scalar field energy density varies with time in constant proportion to the dominant energy density. The evidence is that radiation dominates at redshifts in the range 10³ z 10¹⁰, from the success of the standard model for light element formation, and matter dominates at 1 z 10³, from the success of the standard model for the gravitational growth of structure. This would leave the dark energy subdominant today, contrary to what is wanted. This led to the proposal of the inverse power-law potential in Eq. (31) for a single real scalar field. ⁵²

We do not want the hypothetical field to couple too strongly to baryonic matter and fields, because that would produce a "fifth force" that is not observed. ^{(53), (54)} Within quantum field theory the inverse power-law scalar field potential makes the model non-renormalizable and thus pathological. But the model is meant to describe what might emerge out of a more fundamental quantum theory, which maybe also resolves the physicists' cosmological constant problem (Sec. III.B), as the effective classical description of the dark energy. ⁽⁵⁵⁾ The potential of this classical effective field is chosen ad hoc, to fit the scenario. But one can adduce analogs within supergravity, superstring/M, and brane theory, as reviewed in the Appendix.

The solution for the mass fraction in dark energy in the inverse power-law potential model (in Eq. [33] when << , and the numerical solution at lower redshifts) is not unique, but it behaves as what has come to be termed an attractor or tracker: it is the asymptotic solution for a broad range of initial conditions. ⁽⁵⁶⁾ The solution also has the property that is decreasing, but less rapidly than the mass densities in matter and radiation. This may help alleviate two troubling aspects of the cosmological constant. The coincidences issue is discussed in Sec. III.B. The other is the characteristic energy scale set by the value of ,

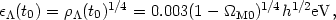(47)

when _R0 and _K0 may be neglected. In the limit of constant dark energy density, cosmology seems to indicate new physics at an energy scale more typical of chemistry. If is rolling toward zero the energy scale might look more reasonable, as follows (Peebles and Ratra, 1988; Steinhardt et al., 1999; Brax et al., 2000).

Suppose that as conventional inflation ends the scalar field potential switches over to the inverse power-law form in Eq. (31). Let the energy scale at the end of inflation be (t_I) = (t_I)^1/4, where (t_I) is the energy density in matter and radiation at the end of inflation, and let (t_I) be the energy scale of the dark energy at the end of inflation. Since the present value (t₀) of the dark energy scale (Eq. [47]) is comparable to the present energy scale belonging to the matter, we have from Eq. (33)

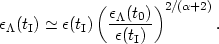(48)

For parameters of common inflation models, (t_I) ~ 10¹³ GeV, and (t₀) / (t_I) ~ 10^-25. If, say, = 6, then

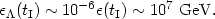(49)

As this example illustrates, one can arrange the scalar field model so it has a characteristic energy scale that exceeds the energy ~ 10³ GeV below which physics is thought to be well understood: in this model cosmology does not force upon us the idea that there is as yet undiscovered physics at the very small energy in Eq. (47). Of course, where the factor ~ 10^-6 in Eq. (49) comes from still is an open question, but, as discussed in the Appendix, perhaps easier to resolve than the origin of the factor ~ 10^-25 in the constant case.

When we can describe the dynamics of the departure from a spatially homogeneous field in linear perturbation theory, a scalar field model generally is characterized by the time-dependent values of w_X (Eq. [43]) and the speed of sound c_sX (e.g., Ratra, 1991; Caldwell et al., 1998). In the inverse power-law potential model the relation between the power-law index and the equation of state parameter in the matter-dominated epoch is independent of time (Ratra and Quillen, 1992),

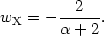(50)

When the dark energy density starts to make an appreciable contribution to the expansion rate the parameter w_X starts to evolve. The use of a constant value of w_X to characterize the inverse power-law potential model thus can be misleading. For example, Podariu and Ratra (2000, Fig. 2) show that, when applied to the Type Ia supernova measurements, the XCDM parametrization in Eq. (50) leads to a significantly tighter apparent upper limit on w_X, at fixed _M0, than is warranted by the results of a computation of the evolution of the dark energy density in this scalar field model. Caldwell et al. (1998) deal with the relation between scalar field models and the XCDM parametrization by fixing w_X, as a constant or some function of redshift, deducing the scalar field potential V() that produces this w_X, and then computing the gravitational response of to the large-scale mass distribution.

⁵⁰ Other early examples include those cited in Ratra and Peebles (1988) as well as Endo and Fukui (1977), Fujii (1982), Dolgov (1983), Nilles (1985), Zee (1985), Wilczek (1985), Bertolami (1986), Ford (1987), Singh and Padmanabhan (1988), and Barr and Hochberg (1988). Back.

⁵¹ For recent discussions of this model see Ferreira and Joyce (1998), Ott (2001), Hwang and Noh (2001), and references therein. Back.

⁵² In what follows we focus on this model, which was proposed by Peebles and Ratra (1988). The model assumes a conventionally normalized scalar field kinetic energy and spatial gradient term in the action, and it assumes the scalar field is coupled only to itself and gravity. The model is then completely characterized by the form of the potential (in addition to all the other usual cosmological parameters, including initial conditions). Models based on other forms for V(), with a more general kinetic energy and spatial gradient term, or with more general couplings to gravity and other fields, are discussed in the Appendix. Back.

⁵³ The current value of the mass associated with spatial inhomogeneities in the field is m(t₀) ~ H₀ ~ 10^-33 eV, as one would expect from the dimensions. More explicitly, one arrives at this mass by writing the field as (t, ) = <>(t) + (t, ) and Taylor expanding the scalar field potential energy density V() about the homogeneous mean background <> to quadratic order in the spatially inhomogeneous part , to get m² = V"(<>). Within the context of the inverse power-law model, the tiny value of the mass follows from the requirements that V varies slowly with the field value and that the current value of V be observationally acceptable. The difference between the roles of m and the constant m_q in the quadratic potential model V = m_q^{2 2} / 2 is worth noting. The mass m_q has an assigned and arguably fine-tuned value. The effective mass m ~ H belonging to V ^- is a derived quantity, that evolves as the universe expands. The small value of m(t₀) explains why the scalar field energy cannot be concentrated with the non-relativistic mass in galaxies and clusters of galaxies. Because of the tiny mass a scalar field would mediate a new long-range fifth force if it were not weakly coupled to ordinary matter. Weak coupling also ensures that the contributions to coupling constants (such as the gravitational constant) from the exchange of dark energy bosons are small, so such coupling constants are not significantly time variable in this model. See, for example, Carroll (1998), Chiba (1999), Horvat (1999), Amendola (2000), Bartolo and Pietroni (2000), and Fujii (2000) for recent discussions of this and related issues. Back.

⁵⁴ Coupling between dark energy and dark matter is not constrained by conventional fifth force measurements. An example is discussed by Amendola and Tocchini-Valentini (2001). Perhaps the first consideration is that the fifth-force interaction between neighboring dark matter halos must not be so strong as to shift regular galaxies of stars away from the centers of their dark matter halos. Back.

⁵⁵ Of course, the zero-point energy of the quantum-mechanical fluctuations around the mean field value contributes to the physicists' cosmological constant problem, and renormalization of the potential could destroy the attractor solution (however, see Doran and Jäckel, 2002) and could generate couplings between the scalar field and other fields leading to an observationally inconsistent "fifth force". The problems within quantum field theory with the idea that the energy of a classical scalar field is the dark energy, or drives inflation, are further discussed in the Appendix. The best we can hope is that the effective classical model is a useful approximation to what actually is happening, which might lead us to a more satisfactory theory. Back.

⁵⁶ A recent discussion is in Brax and Martin (2000). Brax, Martin, and Riazuelo (2000) present a thorough analysis of the evolution of spatial inhomogeneities in the inverse power-law scalar field potential model and confirm that these inhomogeneities do not destroy the homogeneous attractor solution. For other recent discussions of attractor solutions in a variety of contexts see Liddle and Scherrer (1999), Uzan (1999), de Ritis et al. (2000), Holden and Wands (2000), Baccigalupi, Matarrese, and Perrotta (2000), and Huey and Tavakol (2002). Back.