


3.2. Accuracy of
Determination of
 v and
wLG
v and
wLG
The mock catalogs also enable us to
determine the reliability of the small-scale velocity dispersion
 v derived
from VELMOD. This quantity may be viewed as the quadrature sum of true
velocity noise
(
v derived
from VELMOD. This quantity may be viewed as the quadrature sum of true
velocity noise
( nv)
and IRAS velocity prediction errors
(
nv)
and IRAS velocity prediction errors
( Iv)
resulting
from shot noise and imperfectly modeled nonlinearities. (For the real data,
there is an additional contribution from redshift measurement errors, which
are zero in the mock catalog.) We can measure both
Iv)
resulting
from shot noise and imperfectly modeled nonlinearities. (For the real data,
there is an additional contribution from redshift measurement errors, which
are zero in the mock catalog.) We can measure both
 nv
and
nv
and  Iv
directly from the mock catalogs. To measure velocity noise, we
determined
Iv
directly from the mock catalogs. To measure velocity noise, we
determined  u,
the rms value of pair velocity differences cz(ri)
- cz(rj)
of mock catalog TF galaxies within 3500 km s-1 outside of the
mock Virgo core, for |ri - rj|
u,
the rms value of pair velocity differences cz(ri)
- cz(rj)
of mock catalog TF galaxies within 3500 km s-1 outside of the
mock Virgo core, for |ri - rj|
 rmax.
We found
rmax.
We found
 u to
be insensitive to the precise value of rmax, provided it
was
u to
be insensitive to the precise value of rmax, provided it
was  150
km s-1, implying that we are not including the gradient of the
true velocity field on these scales. Taking rmax = 150 km
s-1, we found
150
km s-1, implying that we are not including the gradient of the
true velocity field on these scales. Taking rmax = 150 km
s-1, we found
 u
= 71 km s-1, corresponding
to
u
= 71 km s-1, corresponding
to  nv
=
nv
=  u /
21/2 = 50 km
s-1. This value is so small because the PM code does not
properly model particle-particle interactions on small scales.
u /
21/2 = 50 km
s-1. This value is so small because the PM code does not
properly model particle-particle interactions on small scales.
We measured the IRAS prediction errors
 Iv
as follows. For each mock TF particle (again, within 3500 km s-1
and outside the mock Virgo core), we computed an IRAS-predicted
redshift czI =
ri + u(ri) +
fri - wLG .
Iv
as follows. For each mock TF particle (again, within 3500 km s-1
and outside the mock Virgo core), we computed an IRAS-predicted
redshift czI =
ri + u(ri) +
fri - wLG .
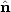 i, where
ri was the true distance of the object,
u(ri) was the IRAS-predicted radial
peculiar velocity in the Local Group frame (for
i, where
ri was the true distance of the object,
u(ri) was the IRAS-predicted radial
peculiar velocity in the Local Group frame (for
 I
= 1), f was a zero-point error in the IRAS model (cf.
Section 3.3),
and wLG
was the mock Local Group peculiar velocity, which (just as in the real
data) is not known precisely and was treated also as a free parameter. We
then minimized the mean squared difference between
czI
and the actual redshifts czi over the entire
TF sample with respect to f and
wLG. The rms value
of (czi - czI) at
the minimum was then our estimate of the quadrature sum of IRAS
prediction error and true velocity noise, which we found to be 98 ± 2
km s-1 after averaging over the 20 mock catalogs. Subtracting
off the small value
of
I
= 1), f was a zero-point error in the IRAS model (cf.
Section 3.3),
and wLG
was the mock Local Group peculiar velocity, which (just as in the real
data) is not known precisely and was treated also as a free parameter. We
then minimized the mean squared difference between
czI
and the actual redshifts czi over the entire
TF sample with respect to f and
wLG. The rms value
of (czi - czI) at
the minimum was then our estimate of the quadrature sum of IRAS
prediction error and true velocity noise, which we found to be 98 ± 2
km s-1 after averaging over the 20 mock catalogs. Subtracting
off the small value
of  nv
found above, we obtain
nv
found above, we obtain
 Iv
Iv
 84
km s-1. This surprisingly small value is indicative of the high
accuracy of the IRAS predictions for nearby galaxies not in
high-density environments.
84
km s-1. This surprisingly small value is indicative of the high
accuracy of the IRAS predictions for nearby galaxies not in
high-density environments.
The value  v
= 98 km s-1 is somewhat smaller than the real universe value
of
v
= 98 km s-1 is somewhat smaller than the real universe value
of  v
= 125 km s-1 (Section 4.5).
Because we
wanted the mock catalogs to reflect the errors in the real data, we added
artificial velocity noise of 110 km s-1 to the redshift of
each mock TF galaxy before applying the VELMOD algorithm,
increasing
v
= 125 km s-1 (Section 4.5).
Because we
wanted the mock catalogs to reflect the errors in the real data, we added
artificial velocity noise of 110 km s-1 to the redshift of
each mock TF galaxy before applying the VELMOD algorithm,
increasing  v
to 147 km s-1.
(11) The mean value
of
v
to 147 km s-1.
(11) The mean value
of  v
from the VELMOD runs on the 20 mock catalogs was
<
v
from the VELMOD runs on the 20 mock catalogs was
< v>
= 148.7 ± 4.6 km s-1, in excellent agreement with
the expected value. We conclude that VELMOD produces an unbiased estimate
of the
v>
= 148.7 ± 4.6 km s-1, in excellent agreement with
the expected value. We conclude that VELMOD produces an unbiased estimate
of the  v,
just as it does
of
v,
just as it does
of  I.
The rms error in the determination
of
I.
The rms error in the determination
of  v
from a single realization is ~ 20 km s-1.
v
from a single realization is ~ 20 km s-1.
The calculation in which we
minimized (czi - czI)2 also
yielded estimates of the Cartesian components of Local Group
random velocity vector wLG.
Their mean values over 20 mock catalogs are given in
Table 1, together with the
corresponding mean values
returned from VELMOD over the 20 mock catalog runs. The two are in
excellent agreement. These values reflect an offset between the cosmic
microwave background (CMB)-to-LG
transformation assigned to the simulation and the average value of
wLG assigned by the mock IRAS reconstruction for
 I
= 1. We conclude that VELMOD properly measures the Cartesian components
of wLG to within ~ 50 km
s-1 accuracy per mock catalog.
I
= 1. We conclude that VELMOD properly measures the Cartesian components
of wLG to within ~ 50 km
s-1 accuracy per mock catalog.
11 In
retrospect, we added more noise than was necessary, but at the time we had
a higher estimate of the real
universe  v.
Back
v.
Back