4. THE CIB-CRB CONNECTION FOR VARIOUS COSMIC STAR FORMATION HISTORIES

Several models have been recently developed for calculating the evolution of _IR(z) with redshift. Here we will focus on some select representative cases: Malkan & Stecker (2001, baseline model), Chary & Elbaz (2001, pure luminosity evolution model), and Xu et al. (2001, peak model). These models were developed to explain the limits and detections of the CIB spectrum and galaxy number counts obtained with the IRAS, the Infrared Space Observatory (ISO), and the Submillimeter Common User Bolometric Array (SCUBA) instrument on the James Clerk Maxwell Telescope (JCMT) at various IR and submillimeter wavelengths. Figure 2 presents the evolution of _IR(z) for these select models. Also plotted are observational estimates of the comoving cosmic star formation rate (CSFR) at different redshifts. Star formation rates were converted to IR luminosity densities using the relation (Kennicutt 1998): _IR(L Mpc^-3) = 6 × 10⁹ × _*(M yr^-1 Mpc^-3). References to the CSFR data can be found in the papers listed above. Also shown in the figure are approximate upper and lower limits to the IR luminosity density. The top-hat function centered on redshift z = 1 in the figure represents the instantaneous energy injection model used by Haarsma & Partridge (1998) to calculate the CRB. The amplitude of the function was chosen so that it reproduced the nominal ~ 5-1000 µm CIB intensity of 50 nW m^-2 sr^-1 (Hauser & Dwek 2001).

Figure 2. The comoving IR luminosity density predicted by several galaxy number count models as a function of redshift. The data points represent optical and IR determinations of the comoving cosmic star formation rate as a function of redshift (references to the data can be found in the papers listed above). A scaling factor of 6 × 109 was used to convert the CSFR (in M yr-1) to an IR luminosity density (in L Mpc-3). The dashed blue lines represent approximate upper and lower limits to the CSFR.

Figure 3 shows the value of g() as a function of for the different star formation histories depicted in Figure 2. The function g() is insensitive to the cosmic star formation history, a direct consequence of the fact that the integrands in eq. (13) differ only by a factor of (1 + z)^-1. For 0.6-1.2, the most probable range of values for the radio spectral index, g() is well approximated by:

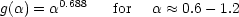(14)

to an accuracy better than 2%.

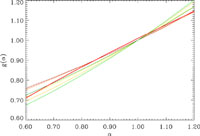Figure 3. The function g(), defined by eq. (13) is plotted against for the various CSFR depicted in Figure 2. The thick red line represents a 0.688 power-law fit to the function for values between 0.6 and 1.2.

Using eqs. (12), (14) and the linear radio-IR correlation at ₀ = 1.49 GHz: P(W Hz^-1) = 2.47 × 10¹¹ L_IR(L), we can express the CIB intensity in terms of the radio brightness temperature at frequency _R = 178 MHz as:

(15)

Table 1 lists the values of the CIB intensity and the 178 MHz brightness temperature obtained from the respective use of eqs. (10) and (11) for the different star formation histories and radio spectral index . The entries in the table satisfy the analytical approximation for the relation between I_CIB, T_crb, and to an accuracy of a few percent. For comparison we also listed the observed limits and detections of the CIB (Hauser & Dwek 2001) and the 178 MHz CRB brightness temperature inferred from the Bridle data (Bridle 1967) for the different values of . Also shown in the table are the observed T_crb/I_CIB ratios and those predicted by the CIB-CRB correlation [eq. (15)].

Table 1. CIB and CRB Intensities Predicted by Various Models for the Cosmic Star Formation Rate ^a

Model	ICIB(nW m-2 sr-1)	Tcrb(K) at 178 MHz
	(3.5-1000 µm)	= 0.6	= 0.7	= 0.8	= 0.9
minimum CSFR	8.5	2.7	3.1	3.5	4.1
maximum CSFR	73	24.8	28.0	31.5	35.6
Haarsma & Partridge (1998)	50	15.7	18.1	20.8	24.1
Malkan & Stecker (2001)	23	8.2	9.1	10.2	11.4
Chary & Elbaz (2001)	37	12.3	13.9	15.8	18.0
Xu et al. (2001)	58	18.8	21.5	24.5	27.9
Observational Limits b	50±25	57±11	37±8	23±5	15±3
-1 Tcrb / ICIB (calculated)c		0.33	0.37	0.42	0.48
Tcrb / ICIB (observed)		1.1±0.6	0.7±0.4	0.5±0.25	0.30±0.16
a The CSFR predicted by the tabulated models are shown in Figure 2. CIB intensities and CRB temperatures were calculated from eqs. (10) and (11), respectively. The parameter is the spectral index of the radio sources.
b Observational limits on the CIB intensity are summarized in Hauser & Dwek (2001). CRB temperatures were taken from Bridle (1967, Table VIII).
c Calculated using eq. (15).

It is interesting to compare our model predictions with the result obtained by Haarsma & Partridge (1998). HP98 found that the star-forming galaxies that produce the detected 120-260 µm CIB intensity of ~ 22 nW m^-2 sr^-1 contribute about 15 K to the 178 MHz brightness temperature (they adopted a value of = 0.7). This result seems at first glance to be in disagreement with the entry for the HP98 model in the table. The reason for this apparent "discrepancy" is that HP98 expressed the radio-IR correlation in terms of the FIR luminosity of galaxies. Had they expressed this correlation in terms of their IR luminosity, which is about twice the FIR value, they would have derived a radio brightness temperature of ~ 17 K for a 3.5-1000 µm background intensity of 50 nW m^-2 sr^-1, almost identical to the value listed in the table. The analytical expression presented in this paper reproduces the results derived by HP98 for their specific CIB production scenario, and generalizes their treatment to any cosmic star formation history.