8.4. Inverse Compton Emission

Inverse Compton (IC) scattering may modify our analysis in several ways. IC can influence the spectrum even if the system is optically thin (as it must be) to Compton scattering (see e.g. [245]). In view of the high energies involved we assume that only one IC scattering takes place. After this scattering the photon's energy is so high that in the electron's rest frame it is above the Klein-Nishina energy and in this case the decrease in the Compton cross section makes this scattering unlikely. The effect of IC depends on the Comptonization parameter Y = ² _e. For fast cooling one can show [103] that Y satisfies:

(69)

IC is unimportant if Y < 1 and in this case it can be ignored.

If Y > 1, which corresponds to _e > _B and to Y = (_e / _B)^1/2 then a large fraction of the low energy synchrotron radiation will be up scattered by IC and a large fraction of the energy will be emitted via the IC processes. If those IC up scattered photons will be in the observed energy band then the observed radiation will be IC and not synchrotron photons. Those IC photons might be too energetic, that is their energy may be beyond the observed energy range. In this case IC will not influence the observed spectra directly. However, as IC will take a significant fraction of the energy of the cooling electrons it will influence the observations in two ways: it will shorten the cooling time (the emitting electrons will be cooled by both synchrotron and IC process). Second, assuming that the observed -ray photons results from synchrotron emission, IC will influence the overall energy budget and reduce the efficiency of the production of the observed radiation. We turn now to each of this cases.

Consider, first, the situation in which Y > 1 and the IC photons are in the observed range so that some of the observed radiation may be due to IC rather than synchrotron emission. This is an interesting possibility since one might expect that the IC process will ease the requirement of rather large magnetic fields that is imposed by the synchrotron process. We show here that, somewhat surprisingly, this cannot be the case.

An IC scattering boosts the energy of the photon by a factor ²_e. Typical IC photons will be observed at the energy:

(70)

where B_1G = B / 1 Gauss and _{E100 E/100}. The Lorentz factor of electrons radiating synchrotron photons which are IC scattered on electrons with the same Lorentz factor and have energy h in the observed range is the square root of the _e required to produce synchrotron radiation in the same frequency. The required value for _e is rather low relative to what one may expect in an external shock (in which _e,ext ~ _e(m_p / m_e) _sh). In internal shocks we expect lower values (_e,int ~ _e(m_p / m_e)) but in this case the equipartition magnetic field is much stronger (of the order of few thousand Gauss, or higher). Thus IC might produce the observed photons in internal shocks if _B is rather small (of order 10^-5).

These electrons are cooled both by synchrotron and by IC. The latter is more efficient and the cooling is enhanced by the Compton parameter Y. The cooling time scale is:

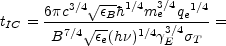(71)

As we see in the following discussion for external shocks, t_IC(100 keV), the IC cooling time if the IC radiation is in the observed range (soft gamma-rays) is too long, while for internal shocks t_IC(100 keV) is marginal. However, even if IC does not produce the observed -ray photons it still influences the process if Y > 1. It will speed up the cooling of the emitting regions and shorten the cooling time, t_syn estimated earlier (Eq. 59) by a factor of Y. Additionally IC also reduces the efficiency by the same factor, and the efficiency becomes extremely low as described below.