Discussion of BBN can begin when the Universe is already a few tenths of a second old and the temperature is a few MeV. At such early epochs the Universe is too hot and dense to permit the presence of complex nuclei in any significant abundances and the baryons (nucleons) are either neutrons or protons whose relative abundances are determined by the weak interactions
 |
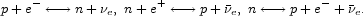(1) |
The higher neutron mass favors protons relative to neutrons, ensuring
proton dominance. When the weak interaction rates (Eq. 1)
are fast compared to the universal expansion rate (and in the absence
of a significant chemical potential for the electron neutrinos),
n / p 
exp(- 
m /
T), where
m is the
neutron-proton mass difference and T is the temperature
(T
= Te =
T
=
TN prior to e±
annihilation). If there were an asymmetry
between the number densities of
e and
e ("neutrino
degeneracy"), described by a chemical potential µe
(or, equivalently, by the dimensionless degeneracy parameter
e
µe / T) then, early on,
n / p
exp(- m /
T -
e).
For a significant positive chemical potential
(e
0.01; more
e than
e) there
are fewer neutrons than for the "standard" case
(SBBN) which, as described below, leads to the formation of less
4He.
The first step in building complex nuclei is the formation of deuterons
via n + p
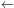
D +
.
Sufficiently early on, when
the Universe is very hot (T
80
keV), the newly-formed deuterons find
themselves bathed in a background of gamma rays (the photons whose relics
have cooled today to form the CBR at a temperature of 2.7 K) and are
quickly
photo-dissociated, removing the platform necessary for building heavier
nuclides. Only below ~ 80 keV has the Universe cooled sufficiently
to permit BBN to begin, leading to the synthesis of the lightest nuclides
D, 3He, 4He, and 7Li. Once BBN begins,
D, 3H, and 3He are rapidly
burned (for the baryon densities of interest) to 4He, the
light nuclide
with the largest binding energy. The absence of a stable mass-5 nuclide,
in combination with Coulomb barriers, suppresses the BBN production of
heavier nuclides; only 7Li is synthesized in an
astrophysically interesting
abundance. All the while the Universe is expanding and cooling. When
the temperature has dropped below ~ 30 keV, at a time comparable
to the neutron lifetime, the thermal energies of the colliding nuclides
is insufficient to overcome the Coulomb barriers, the remaining free
neutrons decay, and BBN ends.
From this brief overview of BBN it is clear that the relic abundances of the nuclides produced during BBN depend on the competition between the nuclear and weak interaction rates (which depend on the baryon density) and the universal expansion rate (quantified by the Hubble parameter H), so that the relic abundances provide early-Universe baryometers and chronometers.