


2.2. The SBBN-Predicted Abundances
The primordial abundances of D, 3He, and
7Li(7Be) are rate
limited, depending sensitively on the competition between the
nuclear reactions rates and the universal expansion rate. As
a result, these nuclides are potential baryometers since their
abundances are sensitive to the universal density of nucleons.
As the universe expands, the nucleon density decreases so it
is useful to compare the nucleon density to that of the CMB photons

 nN /
n
nN /
n . Since this ratio
will turn out to be very small, it is convenient to introduce
. Since this ratio
will turn out to be very small, it is convenient to introduce
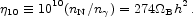 |
(25) |
As the universe evolves (post-e± annihilation)
this ratio is accurately preserved so that
 BBN
=
BBN
=  0.
Testing this relation over ten orders of magnitude in redshift,
over a range of some ten billion years, can provide a confirmation
of or a challenge to the standard model.
0.
Testing this relation over ten orders of magnitude in redshift,
over a range of some ten billion years, can provide a confirmation
of or a challenge to the standard model.
In contrast to the other light nuclides, the primordial abundance
of 4He (mass fraction Y) is relatively insensitive to the baryon
density, but since virtually all neutrons available at BBN are
incorporated in 4He, it does depend on the competition between
the weak interaction rate (largely fixed by the accurately measured
neutron lifetime) and the universal expansion rate (which depends
on geff). The higher the nucleon density, the earlier can
the D-bottleneck be breached. At early times there are more
neutrons and, therefore, more 4He will be synthesized. This
latter effect is responsible for the very slow (logarithmic)
increase in Y with
 . Given the
standard model relation
between time and temperature and the nuclear and weak cross
sections and decay rates measured in the laboratory, the evolution
of the light nuclide abundances may be calculated and the frozen-out
relic abundances predicted as a function of the one free parameter,
the nucleon density or
. Given the
standard model relation
between time and temperature and the nuclear and weak cross
sections and decay rates measured in the laboratory, the evolution
of the light nuclide abundances may be calculated and the frozen-out
relic abundances predicted as a function of the one free parameter,
the nucleon density or
 . These are
shown in Figure 1.
. These are
shown in Figure 1.
Not shown on Figure 1 are the relic abundances
of 6Li, 9Be,
10B, and 11B, all of which, over the same range in
 ,
lie offscale, in the range 10-20 - 10-13.
,
lie offscale, in the range 10-20 - 10-13.
The reader may notice the abundances appear in
Figure 1 as bands.
These represent the theoretical uncertainties in the predicted
abundances. For D/H and 3He/H they are at the ~ 8% level,
while they are much larger, ~ 12%, for 7Li. The reader may
not notice that a band is also shown for 4He, since the
uncertainty in Y is only at the ~ 0.2% level
( Y
Y
 0.0005). The results
shown here are from the BBN code developed
and refined over the years by my colleagues at The Ohio State
University. They are in excellent agreement with the published
results of the Chicago group
(Burles, Nollett &
Turner 2001)
who, in a reanalysis of the relevant published cross sections
have reduced the theoretical errors by roughly a factor of three
for D and 3He and a factor of two for 7Li. The
uncertainty in Y is largely due to the (very small) uncertainty in the
neutron lifetime.
0.0005). The results
shown here are from the BBN code developed
and refined over the years by my colleagues at The Ohio State
University. They are in excellent agreement with the published
results of the Chicago group
(Burles, Nollett &
Turner 2001)
who, in a reanalysis of the relevant published cross sections
have reduced the theoretical errors by roughly a factor of three
for D and 3He and a factor of two for 7Li. The
uncertainty in Y is largely due to the (very small) uncertainty in the
neutron lifetime.
The trends shown in Figure 1 are easy to
understand based on our previous discussion. D and 3He are
burned to 4He. The higher
the nucleon density, the faster this occurs, leaving behind fewer
nuclei of D or 3He. The very slight increase of Y with
 is largely due to BBN starting earlier, at higher nucleon density
(more complete burning of D, 3H, and 3He to
4He) and
neutron-to-proton ratio (more neutrons, more 4He). The behavior
of 7Li is more interesting. At relatively low values of
is largely due to BBN starting earlier, at higher nucleon density
(more complete burning of D, 3H, and 3He to
4He) and
neutron-to-proton ratio (more neutrons, more 4He). The behavior
of 7Li is more interesting. At relatively low values of

 3, mass-7 is
largely synthesized as 7Li (by
3H(
3, mass-7 is
largely synthesized as 7Li (by
3H( ,
,
 )
7Li reactions) which is easily destroyed
in collisons with protons. So, as
)
7Li reactions) which is easily destroyed
in collisons with protons. So, as
 increases at
low values,
7Li/H decreases. However, at relatively high values of
increases at
low values,
7Li/H decreases. However, at relatively high values of

 3, mass-7 is
largely synthesized as 7Be (via
3He(
3, mass-7 is
largely synthesized as 7Be (via
3He( ,
,
 )
7Be reactions) which is more tightly
bound than 7Li and, therefore, harder to destroy. As
)
7Be reactions) which is more tightly
bound than 7Li and, therefore, harder to destroy. As
 increases at high values, the abundance of 7Be increases.
Later in the evolution of the universe, when it is cooler and
neutral atoms begin to form, 7Be will capture an electron
and
increases at high values, the abundance of 7Be increases.
Later in the evolution of the universe, when it is cooler and
neutral atoms begin to form, 7Be will capture an electron
and  -decay to
7Li.
-decay to
7Li.