5. WITNESSING THE END OF COSMIC REIONIZATION

5.1. Introduction - Some Weighty Questions

We now turn to the exciting and rapidly developing area of understanding cosmic reionization. This event, which marks the end of the so-called `Dark Ages' when the intergalactic medium became transparent to ultraviolet photons, was a landmark in cosmic history. In some ways the event might be considered as important as the epoch of recombination which isolates the formation of the hydrogen atom, or the asssociated surface of last scattering when photons and baryonic matter decouple. In the case of the era of cosmic reionization, although we cannot yet be sure, many believe we are isolating that period when the first sources had sufficient output to contribute to the energy balance of the intergalactic medium. Even though some early luminous forerunners might be present, the epoch of reionization can be directly connected with cosmic dawn for starlight.

It seems an impossible task to give an authoritative observational account of how to probe this era. So many issues are complete imponderables! When did reionization occur? Was it a gradual event made possible by a complex time sequence of sources, or was there a spectacular synchronized moment? Can we conceive of an initial event, followed by recombination and a second phase?

What were the sources responsible? History has shown the naivety of astronomers in assuming a single population to be responsible for various phenomena - usually some complex combination is the answer. And, perhaps most ambitiously, what is the precise process by which photons escape the sources and create ionized regions?

Four independent observational methods are helpful in constraining the redshift range where we might search for answers to the above questions. In this lecture, we will explore how these work and the current (and rapidly changing) constraints they offer. These are:

• Evolution in the optical depth of Lyman series absorption lines in high redshift quasars (Fan et al 2006a, b). Although QSOs are only found to redshifts of z 6.5, high quality spectroscopic data gives us a glimpse of a potential change in the degree of neutrality of the IGM beyond z 5.5 such as might be expected if reionization was just ending at that epoch.

• The ubiquity of metal absorption lines in the IGM as probed again by various spectra of the highest redshift QSOs. Carbon, in particular, is only produced in stellar nuclei and its presence in all sightlines to z 5-6 (Songaila 2004) is highly indicative of a widespread process of earlier enrichment from the first generation of supernovae.

• The large angular scale power in the temperature - polarization cross-correlation function seen in the microwave background (Kogut et al 2003, Spergel et al 2006). This signal is produced by electron scattering foreground to the CMB. Depending on models of large scale structure, the optical depth of scattering gives some indication of the redshift range where the ionized particles lie.

• The stellar mass density in assembled sources at redshifts z 5-6, about 1 billion years after the Big Bang, as probed by the remarkable combination of HST and Spitzer (Stark & Ellis 2005, Stark et al 2006a, Yan et al 2006, Eyles et al 2006). A census of the mass in stars must be the integral of past activity which can be compared with that necessary to reionize the Universe.

5.2. The Gunn-Peterson Test and SDSS QSOs

In a remarkable paper, long before QSOs were located at redshifts beyond 2.5, James Gunn and Bruce Peterson realized (Gunn & Peterson 1965) that the absence of broad troughs of hydrogen absorption in the spectra of QSOs must indicate intergalactic hydrogen is ionized. They postulated a future test whereby the spectra of QSOs of successively higher redshift would be scrutinized to locate that epoch when the IGM was neutral.

For an optical spectrum, redshifted to reveal that portion of the rest-frame UV shortward of the Lyman emission line of the QSO itself ( 1216 Å), the relative transmission T is defined as

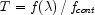where f_cont represents the continuum radiation from the QSOs. The transmission is reduced by Lyman absorption in any foreground (lower redshift) clouds of neutral hydrogen whose Gunn-Peterson opacity is then

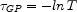The first `complete troughs' in the absorption line spectra of distant QSO were presented by Becker et al (2001) and Djorgovski et al (2001) and a more comprehensive sampling of 11 SDSS QSOs was presented by Fan et al (2003). Recently, an analysis of 19 5.74 < z < 6.42 QSOs was presented by Fan et al (2006b).

Figure 35 illustrates how absorption structures along the same line of sight can be independently probed using Lyman and higher order lines such as Lyman .

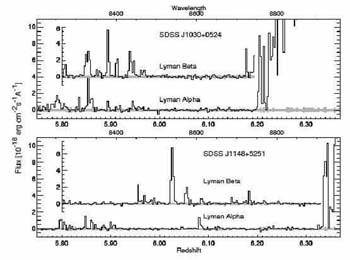Figure 35. The absorption line spectrum of two SDSS QSOs from the recent study of Fan et al (2006b). Here the structures in the Lyman series absorption lines and have been aligned in redshift space thereby improving the signal to noise along a given sightline. The effects of cosmic variance can clearly be seen by comparing the structures seen along the two sightlines.

To understand how this is effective, in a uniform medium _GP is related to the abundance of absorbing neutral hydrogen atoms by the following expression:

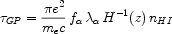where f is the oscillator strength of the Lyman line, H is the redshift-dependent Hubble parameter and n_HI is the neutral number density.

Numerically, this becomes

where x_HI = (1 + z / 7)^3/2 n_HI / n_H is then the neutral fraction.

Inspection of this equation is quite revealing. Firstly, even a tiny neutral fraction, x_HI ~ 10^-4, would give a very deep, seemingly complete GP trough; for reference x_HI 10^-5 today. So clearly the test is not a very sensitive one in absolute terms.

Secondly, since _GP f , for the same n_H, the optical depth in the higher order Lyman and lines would be 6 and 18 times smaller respectively.

In practice, the above relations are greatly complicated by any clumpiness in the medium. This affects our ability to make direct inferences on x_HI as well as to combine the various Lyman lines into a single test.

Instead, workers have examine the relative distribution of _GP with redshift independently from the various Lyman series absorption statistics. An increase in _GP with redshift could just be a natural thickening of the Lyman absorption forest and, given its weak connection with x_HI, not imply anything profound about cosmic reionization. However, if it can be shown empirically that the various diagnostics show a discontinuity in the x_HI-redshift trends, conceivably we are approaching the neutral era.

Figure 36 shows that for z < 5.5, (1 + z / 5)^4.3 for both the Ly and Ly forests to reasonable precision. However, beyond z 5.5, both redshift trends are much steeper, (1 + z)¹¹. The dispersion around the trends also increases significantly at higher redshift. Taken together, both results suggest a qualitative change in nature of the IGM beyond z 5.5 (but for an alternative explanation see Becker et al 2006).

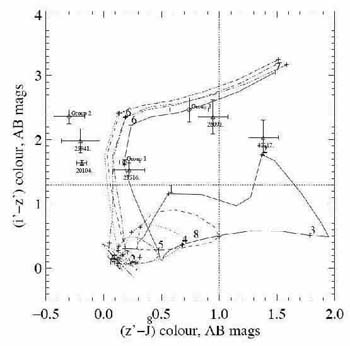Figure 36. Evolution in the Gunn-Peterson optical depth, , for both the Lyman (left) and (right) forests from the distant SDSS QSO analysis of Fan et al (2006). The dotted lines represent fits to the data for zabs < 5.5, beyond which there is evidence in both species for an upturn in the opacity of the intergalactic medium.

Fan et al (2006a, b) discuss several further probes of the nature of the IGM at z 6. One relates to the proximity effect - the region around each QSO where it is clear from the spectrum that the IGM is being ionized by the QSO itself. Although this region is excluded in the analyses above, the extent of this region contains valuable information on the nature of the IGM. It appears that the radius of the region affected, R is less at higher redshift according to R [(1 + z) x_HI]^-1/3 suggesting that the most distant QSOs in the sample (z 6.5) lie in a IGM whose neutral fraction is 14 times higher than those at z 5.7.

Another valuable measure is how the regions of complete absorption, the so-called `dark gaps' in the spectrum where transmission is effectively zero, are distributed. Fan et al define a `gap' as a contiguous region in redshift space where > 3.5. The distribution of gaps contains some information on the topology of reionization. We would expect regions of high transmission to be associated with large HII regions, centered on luminous star-forming sources. The dark gaps increase in extent from 10 to 80 comoving Mpc over the redshift range samples suggesting the IGM is still not neutral at z 6.5. Although the Gunn-Peterson and gap statistics make similar statements about reionization, suggesting the neutral fraction at z 6.2 is 1-4%, Fan et al consider that beyond z 6.5, gap statistics will become a more powerful probe. This is because the redshift distribution of those few spectroscopic pixels where the transmission is non-zero will become the only effective signal.

5.3. Metallicity of the High Redshift IGM

A second measure of the redshift range of early star formation is contained in the properties of the CIV forest observed in the spectra of high redshift QSOs (Songaila 2005, 2006). Carbon is only produced in stellar nuclei (it is not produced in the hot Big Bang) and so the ubiquity of CIV along many sightlines to z 5-6 QSOs is a powerful argument for early enrichment.

CIV was seen in the Ly forest in 1995 (Cowie et al 1995) with N(CIV) / N(HI) ~ 10^-2 to 10^-3. However, it was subsequently seen in even the weakest Ly systems (Ellison et al 2000). This is a particularly powerful point since it argues that enrichment is not confined to localized regions of high column density but is generic to the intergalactic medium as a whole (Figure 37).

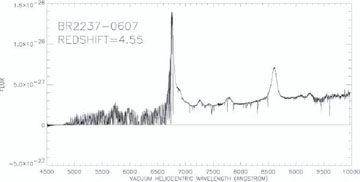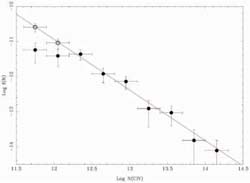Figure 37. (Left) Keck ESI absorption line spectrum of the z = 4.5 QSO BR2237-0607 from the study of Songaila (2005). A sparsely populated CIV forest from 7500-8500Å accompanies the dense Ly forest seen below 6800 Å . (Right) Distribution of column densities of CIV absorbers per unit redshift interval in Q1422+231 from the survey of Ellison et al (2000).

A quantitative interpretation of the CIV abundance, in terms of how much early star formation occurred earlier than the highest redshift probed, relies on locating a `floor' in the abundance-redshift relation. Unfortunately, the actual observed trend, measured via the contribution of the ion to the mass density (CIV) from z 5 to 2, does not seem to behave in the manner expected. For example, there is no strong rise in the CIV abundance to lower redshift despite the obvious continued star formation that occurred within these epochs (Songaila 2006, Figure 38). This is a major puzzle (c.f. Oppenheimer et al 2006).

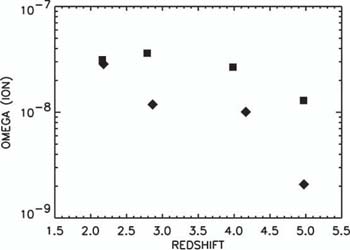Figure 38. Modest evolution in the contribution of intergalactic CIV and SIV over 2 < z < 5 as measured in terms of the ionic contribution to the mass density, (Songaila 2005).

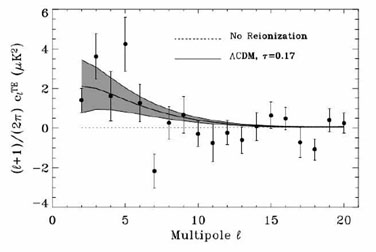
5.4. Linear Polarization in the WMAP Data

In 2003, the WMAP team (Kogut et al 2003) presented the temperature-polarization cross angular power spectrum from the first year's data and located a 4 non-zero signal at very low multipoles (l < 8) which they interpreted in terms of foreground electron scattering of microwave background photons with an optical depth _e = 0.17 ± 0.04 corresponding to ionized structures at z_reion 20 (Figure 39a). The inferred redshift range depends sensitively on the history of the reionization process. Bennett et al (2003) argued that if reionization occurred instantaneously it corresponds to a redshift z_reion 17 ± 5, whereas adopting a more reasonable Press-Schechter formalism and an illustrative cooling and enrichment model, Fukugita & Kawasaki (2003) demonstrated that the same signal can be interpreted with a delayed reionization occurring at z_reion 9-10.

Just before the Saas-Fee lectures, the long-awaited third year WMAP data was published (Spergel et al 2006). A refined analysis significantly lowered both the normalization of the dark matter power spectrum to ₈ = 0.74 ± 0.05, and the optical depth to electron scattering to _e = 0.09 ± 0.03. The same model of instantaneous reionization reduces the corresponding redshift to z_reion = 11 ± 3 (2) - a significant shift from the 1 year data.

To illustrate the uncertainties, Spergel et al introduce a more realistic history of reionization via the ionization fraction x_e. Suppose above z_reion, x_e 0 and below z = 7, x_e 1. Then suppose z_reion is defined as that intermediate point when x_e = x_e⁰ for 7 < z < z_reion. Figure 39b illustrates the remarkable insensitivity of z_reion to the adopted value of x_e⁰ for x_e⁰ < 0.5. Despite improved data, the redshift range implied by the WMAP data spans the full range 10 < z < 20.

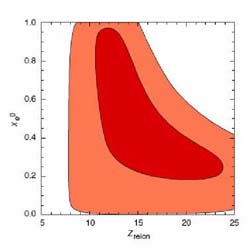Figure 39. (Left) The 4 detection of reionization via an excess signal at large scales in the angular cross correlation power spectrum of the temperature and polarization data in the first year WMAP data (Kogut et al 2003). (Right) Constraints on the redshift of reionization, zreion, from the third year WMAP data (Spergel et al 2006). The contours illustrate how the zreion inferred from the lowered optical depth depends on the history of the ionized fraction xe(z), see text for details.

5.5. Stellar Mass Density at z 5-6

Neither the Gunn-Peterson test nor the WMAP polarization data necessarily demonstrate that reionization was caused by early star-forming sources; both only provide constraints on when the intergalactic medium was first reionized. The CIV test is a valuable complement since it provides a measure of early enrichment which can only come from star-forming sources. Unfortunately, powerful though the ubiquitous presence of CIV is in this context, as we have seen, quantitative constraints are hard to derive. We thus seek a further constraint on the amount of early star formation that might have occurred.

In Lecture 4 we introduced the techniques astronomers are now using to derive stellar masses for distant galaxies. Although the techniques remain approximate, it must follow that the stellar mass density at a given epoch represents the integral over time and volume of the past star formation. Indeed, we already saw a successful application of this in reconciling past star formation with the local stellar mass density observed by 2dF (Figure 21). Specifically, at a particular redshift, z

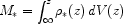Using the techniques described in Lecture 4, stellar mass estimates have become available for some very high redshift galaxies detected by Spitzer (Eyles et al 2005, Mobasher et al 2005, Yan et al 2005). For the most luminous Lyman `dropouts', these estimates are quite substantial, some exceeding 10¹¹ M implying much earlier activity. Recently, several groups (Stark et al 2006a, Yan et al 2006, Eyles et al 2006) have been motivated to provide the first crude estimates on the volume averaged stellar mass density at these early epochs. Part of this motivation is to check whether the massive galaxies seen at such high redshift can be reconciled with hierarchical theory, but as Stark & Ellis (2005) proposed, the established stellar mass can also be used to probe earlier star formation and its likely impact upon cosmic reionization.

A very relevant question is whether the observed mass density at z 5-6 is greater than can be accounted for by the observed previous star formation history. We will review the rather uncertain data on the star formation density _*(z) beyond z 6 in the next Lecture. However, Stark et al (2006a) find that even taking a reasonably optimistic measure of _*(z) from recent compilations by Bouwens et al (2006) and Bunker et al (2006), it is hard to account for the stellar mass density at z 5 (Figure 40).

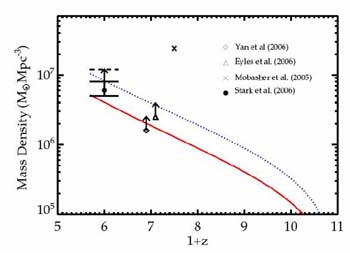Figure 40. A comparison of the assembly history of stellar mass inferred from the observed decline in star formation history to z 10 (solid line) with extant data on the stellar mass density at z 5 and 6 (data points from Stark et al 2006a, Yan et al 2006, Eyles et al 2006). Different estimates at a given redshift represent lower limits based on spectroscopically-confirmed and photometric redshift samples. The red line shows the growth in stellar mass expected from the presently-observed luminous star forming galaxies; a shortfall is observed. The blue dotted line shows the improvement possible when a dominant component of high z lower luminosity systems is included.

There are currently two major limitations in this comparison. First, most of the v- and i-drops are located photometrically; even a small degree of contamination from lower redshift galaxies could upward bias the stellar mass density. On the other hand only star-forming galaxies are located by the Lyman break technique so this bias could easily be offset if there are systems in a quiescent state as evidenced by the prominent Balmer breaks seen in many of the Spitzer-detected sources (Eyles et al 2005, 2006). This limitation will ultimately be overcome with more careful selection methods and deeper spectroscopy. Secondly, and more profoundly, the precision of the stellar masses may not be up to this comparison. Much has to be assumed about the nature of the stellar populations involved which may, quite reasonably, be somewhat different from those studied locally. The discrepancy noted by Stark et al is only a factor of × 2-3, possibly within the range of uncertainty.

Regardless, if this mismatch is reinforced by better data, the implications are very interesting in the context of reionization. It could mean early star-forming systems are extincted, lie beyond z 10 where current searches end, or perhaps most likely that early star formation is dominated by lower luminosity systems (Figure 40). By refining this technique and using diagnostics such as the strength of the tell-tale Balmer break, it may ultimately be possible to age-date the earlier activity and compare its efficacy with that required to reionize the Universe.

5.6. Lecture Summary

In this lecture we have introduced four very different and independent probes of cosmic reionization, each of which suggests star formation activity may extend well into the redshift range 6 < z < 20. Two of these probes rely on a contribution from early star formation (the metallicity of the intergalactic medium and the assembled stellar mass density at z 5-6).

The earliest result was the presence of neutral hydrogen troughs in the spectra of distant QSOs. Although the arguments for reionization ending at z 6 seem compelling at first sight, they ultimately rely on an empirically-deduced transition in the changes in the opacity of the Ly and Ly line below and above z 5.5.

The second result - the ubiquity of carbon in even the weakest absorbing clouds at z 5 is firm evidence for early star formation. However, it seems hard to locate the high redshift `abundance floor' and hence to quantify whether this early activity is sufficient for reionization. Indeed, a major puzzle is the lack of growth in the carbon abundance over the redshift range where galaxies are assembling the bulk of their stars.

The WMAP polarizations results have received the most attention, mainly because the first year data indicated a surprisingly large optical depth and a high redshift for reionization. However, there were some technical limitations in the original analysis and it now seems clear that the constraints on the redshift range when the foreground polarization is produced are not very tight.

Finally, an emerging and very promising technique is simply the census of early star formation activity as probed by the stellar masses (and ages) of the most luminous dropouts at z 5-6. Although significant uncertaintes remain, the prospects for improving these constraints are good and, at this moment, it seems there must have been quite a significant amount of early (z > 6) star formation activity, quite possibly in low luminosity precursors.