3. COSMOGONY

As we have just noticed, the radiation backgrounds contain evidence of what has been happening in the universe now and in the distant past. I discuss here the histories of heavy element formation and the ionization of diffusely distributed matter. Both depend on opinions on the epoch of first star formation in galaxies or pre-galaxy star clusters.

3.1. The Epochs of Structure Formation

The discovery of the CBR, and the demonstration that its spectrum is close to a single Planck function, had two important consequences. First, it gave considerable credibility to the evolving relativistic cosmology, because all sides I have encountered agree that there is no way this radiation could have been thermalized in the universe as it now: the universe has to have expanded from a denser and hotter state. Second, the presence of this radiation, if truly primeval, yields a key constraint on structure formation in the early universe, as follows.

If the CBR is primeval the very early universe was dominated by relativistic material with Jeans length comparable to the Hubble length. That means any departure from homogeneity large enough to break away from the general expansion and form a gravitationally bound system would have had gravitational potential energy large enough for relativistic collapse to a black hole. Unless such black holes are small enough to evaporate by Hawking radiation, their mean energy density scales as a(t)^-3, where a(t) is the expansion factor for the expanding universe, while the energy density of the relativistic material scales as a(t)^-4. Thus structure formation in the very early universe had to have been rare, for otherwise black holes would have made an unacceptably large contribution to the present mean mass density.

In the standard cosmological model, the universe at redshifts less than z_eq ~ 2 x 10⁴ h² is dominated by nonrelativistic matter. In this matter-dominated phase gravity causes the growth of small-scale fluctuations in the distribution of any nonrelativistic nonbaryonic matter that is unaffected by radiation drag or its own pressure. At redshift z_dec ~ 1500 the CBR becomes cool enough to allow the primeval baryonic plasma to combine and decouple from radiation drag, and this matter can join the growing clustering of any nonbaryonic matter. Thus we conclude that in the standard hot evolving cosmology nothing much in the way of structure formation can have happened prior to redshift z ~ 1000 to 10,000.

What was happening at redshift z = 1000? In the Cold Dark Matter model (Frenk 1991) the primeval density fluctuations are adiabatic (fixed entropy per conserved particle number) and Gaussian, with the scale-invariant Zel'dovich power spectrum for which the rms value of the mass density fluctuations appearing at the Hubble length is independent of epoch. In this model the normalization to the density fluctuations appearing at recent epochs implies that the density fluctuations at z = 1000 are small on all scales: baryon structure formation commences at z ~ 30 in clouds at the baryon Jeans mass, about that of a globular star cluster (Peebles 1984). If the fluctuation spectrum were bent to add power on smaller scales it would make structure formation commence earlier. Under isocurvature initial conditions (uniform mass density, entropy per baryon number a random function of position) structure formation could have commenced still earlier, at z ~ 1000.

If star formation at z ~ 1000 reionized an appreciable fraction of the baryons it would recouple the plasma to the CBR, tending to suppress further star formation. ⁽²⁾ I am not aware of any detailed analysis of this self-limiting star formation effect. Perhaps it is a significant coincidence that when radiation drag on diffuse ionized matter becomes unimportant, at z ~ 100, the mean mass density is equivalent to about 10 h² protons cm^-3, which for reasonable values of and h is on the order of the characteristic density within the luminous part of normal giant galaxies.

The material in a galaxy could not have been assembled as a gravitationally bound system until the mean mass density within the object is greater than the cosmological mean. That says the bright parts of normal giant galaxies could not have been assembled before z ~ 100. A more precise and possibly more accurate bound comes from the spherical model. I have trouble believing spherical symmetry is a useful approximation after a protogalaxy has broken away from the general expansion and started collapsing, so I am inclined to put the epoch of assembly at the epoch of maximum expansion in the spherical model (following Partridge and Peebles 1967). This would say the material within r ~ 10h^-1 kpc of an L_* galaxy was assembled at redshift

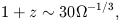
(9)

and the material within the Abell radius of an Abell cluster was assembled at

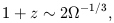
(10)

There are clusters of galaxies at redshift z ~ 1 with the velocity dispersion and central mass concentration characteristic of Abell clusters, so equation (10) does not seem unreasonable. Equation (9) is not inconsistent with the observation of high column density atomic hydrogen gas clouds (the damped Ly systems) detected as broad absorption features in quasar spectra at z ~ 3 (Wolfe 1989). These clouds have the surface densities and comoving number density characteristic of galaxies. Our political leaders have taught us that what quacks like a duck and waddles like a duck very likely is a duck. On the same principle, I think it is a good bet that the damped Ly clouds are members of the long-sought population of young galaxies. To be debated is whether they are typical of the galaxies at that epoch, and whether they are pieces of protogalaxies to be assembled or maybe the first parts of protodiscs collecting around previously assembled spheroids. Meanwhile, a reasonable guess is that the redshift of assembly of the mass in the central parts of galaxies is somewhere between equation (9) and the epoch of the damped Ly systems.

3.2. The History of Element Formation

One has to define what is meant by the epoch of galaxy formation, because different parts of galaxies may have been assembled at quite different times. Thus equation (9) indicates that the inner parts of giant galaxies might have been assembled well before their extended massive dark halos could have been attached. One similarly has to specify what is meant by the epoch of heavy element production, because element production continues to the present day. The present rate in galaxies like the Milky Way is too low to have produced the heavy elements in the time available, however, so there had to have been an epoch when the element production rate per unit mass in the typical progenitor of a normal giant galaxy was considerably larger than it is now. The typical luminosity per unit of mass likely was considerably larger then as well.

We have noted that the damped L systems look like young galaxies. Their heavy element abundances are estimated to be about 10% of solar (Hunstead, Pettini, and Fletcher 1990). This suggests the bulk of element formation in the discs of spirals is at z_e 3.

We have a check from the residual optical/IR background from the starlight that accompanied element formation:

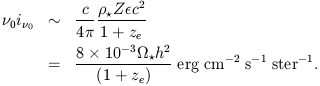
(11)

This assumes the mass fraction Z = 0.03 of baryonic matter with mean mass density _* = _{* crit} has burned from hydrogen to heavier elements, releasing a fraction = 0.007 as starlight. The integrated local background radiation has a minimum near 3 microns, with

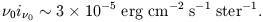
(12)

If 1 + z_e ~ 3, and an appreciable part of the starlight is in the infrared, this would bound the mass density of the heavy element producing material at _* 0.01h^-2, comparable to the baryon density in the standard model for Big Bang nucleosynthesis. Hauser explains the prospects for improving this constraint.

3.3. The Ionization History of Diffuse Matter

Quasar absorption spectra have yielded a remarkably detailed picture of the state of the universe at redshift z ~ 3 (Blades, Turnshek, and Norman 1988). At this epoch the Ly forest clouds fill on the order of 1% to 10% of space, about as much as possible for irregularly shaped clouds. A cloud at the detection limit contains just a few hundred solar masses of neutral atomic hydrogen. The clouds are optically thin to ionizing radiation, however, and the radiation from quasars is sufficient to keep the cloud material highly ionized. At the lowest detected column densities the plasma mass of a cloud is estimated to be about 10⁷ M, that of a present-day dwarf galaxy. The net baryonic mass in these clouds is comparable to what is present now in the galaxies. That is, we can conclude that at z ~ 3 an appreciable fraction of the baryonic matter in our universe was in diffuse ionized clouds, along with a comparable fraction in the neutral damped Ly systems.

What was the universe like a factor of two further back in expansion, at z ~ 7? The rate of intersection of high column density neutral clouds along a line of sight increases with increasing redshift up to the largest presently known, z ~ 5 (Schneider, Schmidt, and Gunn 1991; Lanzetta 1991). This increase in opacity to ionizing radiation suggests that at z ~ 7 the L forest clouds were considerably less strongly ionized, if they had formed.

The possible ionization history at diffuse baryonic matter back to its early tight coupling to the CBR is constrained by the effect on the spectrum of the CBR by scattering by moving electrons (Zel'dovich and Sunyaev 1969). This is usefully measured by the Sunyaev-Zel'dovich parameter

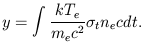
(13)

The plasma has mean pressure n_ekT_e, and _t is the Thomson cross section. The analysis by Field and Perrenod (1977) of models for the origin of the XRB by thermal bremsstrahlung emission from a hot intergalactic plasma predicted values of y considerably in excess of the bounds from the beautiful COBE measurements described by Mather in these Proceedings. There does not seem to be any reasonable way to produce the XRB in hot diffuse plasma either before or after the epoch of the Ly forest; the XRB has to have come from compact sources. Gehrels discusses the fascinating enigma of the XRB sources.

Could a significant part of the baryonic matter have been in diffuse plasma from z ~ 1000 to incorporation in stars at a much lower redshift? The analysis by Bartlett and Stebbins (1991) indicated that relatively cool plasma, as would be produced by photoionization by starlight, need not violate the preliminary COBE bound on y (or the bound on the bremsstrahlung contribution to the 30 cm radio background). The significance of the latest COBE limit on y is under discussion.

Bulk peculiar motions of the plasma act as an effective temperature in the integral in equation (13). Thus plasma in protogalaxies with one-dimensional rms peculiar motion at redshift z contributes

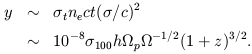
(14)

Here is the cosmological density parameter, _p is the density parameter in optically thin plasma in the protogalaxies, and the velocity dispersion is measured in units of 100 km s^-1. The conclusion is that it is not difficult to choose parameters that reconcile the COBE bound on y with the assumption that the bulk of the baryons are in plasma in protogalaxies at the redshift z ~ 30 in equation (9).

The mean optical depth for scattering by electrons is unity at redshift

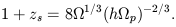
(15)

If structure formed at high redshift in a low density cosmology, with ~ _p ~ 0.1, then the CBR would have been last scattered at z_s ~ 25. This would have smoothed primeval fluctuations in the CBR on angular scales _s ~ 3°. The character of the angular fluctuations in the CBR produced by the scattering plasma depends on what was happening at z_s. One possibility is that the plasma was in protogalaxies with typical angular size _g. Then the contribution to the CBR angular autocorrelation function at separation _g is set by the peculiar velocity autocorrelation function at the mean distance between intersection of clouds along the line of sight, which can be quite small. If this is what happened, untangling the mean of the measurements of the CBR anisotropy may be a considerable challenge.

3.4. Concluding Remarks

Research in the extragalactic background radiation fields has come a long way from the early steps I mentioned in my introductory remarks, but the generally accepted pieces to the puzzle of what it all means still do not fit into any very compelling pattern, which is to say that cosmogony is not yet a very mature branch of physical cosmology. It is one of the most active, however, and one is impressed to note the progress in the last decade in establishing a detailed observational picture of the intergalactic medium back to z ~ 5 and the very significant constraints on what was happening at higher redshifts. It will be fascinating to see what turns up next.

This work was supported in part by the US National Science Foundation.

² A second effect, that the radiation drag on the free electrons would perturb the CBR spectrum, is discussed in the next section. Back.