


Most previous discussions of dust and gas in the host galaxy of an
active nucleus have concentrated on spherically symmetrical
distributions of dust
(Rees et al. 1969,
Bollea & Cavaliere
1976,
Barvainis 1987
- the latter also considered conical sectors, or have
concentrated on their effects on emission line clouds:
Davidson & Netzer
1979,
MacAlpine 1985
and references therein). A few have considered disk-like distributions
(Begelman, McKee &
Shields 1983,
Begelman 1985,
Shlosman & Begelman
1988),
but have assumed the disks
were planar and relatively thin, so that they intercepted only a small
fraction ( 1%) of the
luminosity of the central accretion
disk. Neither of these distributions is very likely. The gas and dust
most probably lie in what could crudely be described as a heavily
warped (and probably clumpy) disk. Beyond about a kiloparsec radius,
the orbital time torb 108 (r / 3
kpc) yr is so long that gas captured
or disturbed by a recent interaction with another galaxy will not have
had time to settle into the preferred plane of the host galaxy's
potential in a quasar lifetime (~ 108 yr). Since quasars seem
commonly to be involved in interactions or mergers
(Hutchings 1983,
MacKenty & Stockton
1984),
large warps and streamers are to be expected. The
warped disk of NGC 5128 (Centurus A) is a famous example. Moving
inwards, warps on kiloparsec scales can be produced by counter
rotating bars
(Vietri 1986),
by continuing infall of gas
(Ostriker & Binney
1989),
and by Kelvin-Helmholtz instability as the disk rotates
through a pressure-supported corona
(Gunn 1979)
Though such warps are
difficult to detect in external galaxies which are not nearly edge-on,
let alone in quasars, the gas at ~ 3 kpc in our own Galaxy is tilted by
about 15° with respect to the plane defined at larger radii
(Vietri 1986),
and most well-studied Seyfert galaxies have strong
kiloparsec-scale bars
(Adams 1977).
1%) of the
luminosity of the central accretion
disk. Neither of these distributions is very likely. The gas and dust
most probably lie in what could crudely be described as a heavily
warped (and probably clumpy) disk. Beyond about a kiloparsec radius,
the orbital time torb 108 (r / 3
kpc) yr is so long that gas captured
or disturbed by a recent interaction with another galaxy will not have
had time to settle into the preferred plane of the host galaxy's
potential in a quasar lifetime (~ 108 yr). Since quasars seem
commonly to be involved in interactions or mergers
(Hutchings 1983,
MacKenty & Stockton
1984),
large warps and streamers are to be expected. The
warped disk of NGC 5128 (Centurus A) is a famous example. Moving
inwards, warps on kiloparsec scales can be produced by counter
rotating bars
(Vietri 1986),
by continuing infall of gas
(Ostriker & Binney
1989),
and by Kelvin-Helmholtz instability as the disk rotates
through a pressure-supported corona
(Gunn 1979)
Though such warps are
difficult to detect in external galaxies which are not nearly edge-on,
let alone in quasars, the gas at ~ 3 kpc in our own Galaxy is tilted by
about 15° with respect to the plane defined at larger radii
(Vietri 1986),
and most well-studied Seyfert galaxies have strong
kiloparsec-scale bars
(Adams 1977).
The same warp-inducing processes can operate on scales of parsecs. The molecular torus extending from 2-8 pc from our Galactic center is tilted by ~ 15° (in the opposite direction from the 3 kpc warp!) with respect to the plane defined by the stars. The parsec-scale dust torus in NGC 1068 (Antonucci & Miller 1985) has its axis at right angles to the kiloparsec-scale disk of gas and stars (Wilson & Ulvestad 1982), and the axes of similar tori inferred to exist in other Seyferts make random angles to the minor axes of the disks of their host galaxies (Unger et al. 1987, Haniff et al. 1988).
We conclude that warps are common and substantial enough to allow the nuclear continuum to illuminate the dust and gas on scales from 1-104 parsecs. The broad-line region (BLR) will be obscured by this dust over a range of viewing angles comparable to the covering factor of the dust disk. Except in the case that the narrow line gas is cospatial with the dusty disk in a symmetrical warp, it is difficult to prevent the narrow line region from being visible from some viewing angles when the BLR is obscured. This occurs for a fraction of sky of order the covering factor of the dust disk extending beyond the NLR: typically ~ 0.05-0.1. Since molecular clouds are larger than the 0.1-1 pc scale of the BLR, the same fraction of quasars with obscured broad line regions would be expected even if the dust were in clouds at high latitudes rather than in a disk. These objects would appear as ``quasar-2's'' (by analogy to Seyfert 2's). Such objects have been rare in optical surveys, but appear common in infrared-selected samples (Sanders et al. 1988b). In radio samples they may masquerade as narrow line radio galaxies (Scheuer 1987, Barthel 1989).
We now examine the state of dust in a warped disk illuminated by the central accretion disk of a quasar, and its possible relevance to the infrared and submillimeter spectra of quasars. We postpone to section 5 a discussion of the vertical structure of an illuminated disk, and the expected optical and radio emission therefrom.
The equilibrium temperature Tg of dust grains of
characteristic
radius a  20
Å at distance r from a radiation source of luminosity
density L
20
Å at distance r from a radiation source of luminosity
density L (erg
s-1 Hz-1) is determined implicitly by
(erg
s-1 Hz-1) is determined implicitly by
where Qabs (
In quasars, most of the energy from the central source is emitted at
frequencies for which Qabs (
where < Qabs(T) > is the Planck-averaged
absorption efficiency
(cf. Draine 1981),
< Qabs(T) >
The heat capacity of grains with a
The emission from dust at temperature Tg at long
wavelengths scales
as 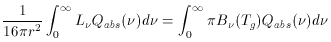
 ) is
the absorption efficiency (cross section in units of
) is
the absorption efficiency (cross section in units of
 a2), at frequency
a2), at frequency
 . Graphite grains with a ~ 0.1
µm are transparent
to X-rays with h
. Graphite grains with a ~ 0.1
µm are transparent
to X-rays with h > 0.4 keV and
for 0.1 keV < h
> 0.4 keV and
for 0.1 keV < h < 0.28 keV
(K-edge of
Carbon), so Qabs(
< 0.28 keV
(K-edge of
Carbon), so Qabs( )
)
 a at those
energies. At wavelengths
a at those
energies. At wavelengths  < 2
< 2
 a
where the grain is not transparent Qabs
a
where the grain is not transparent Qabs
 1. At longer wavelengths
1. At longer wavelengths
 >
2
>
2  a the grain becomes a weakly
coupled antenna, Qabs
a the grain becomes a weakly
coupled antenna, Qabs  (2
(2  a /
a /
 ) f(
) f( )
where f(
)
where f( ) depends on
the dielectric tensor and shape of the grains
(fits for various grain compositions and shapes, and discussion of the
Mie scattering range can be found in
Martin 1978,
Draine & Lee 1984,
Wright 1987,
and references therein). Observations of galactic dust
indicate that outside resonances f(
) depends on
the dielectric tensor and shape of the grains
(fits for various grain compositions and shapes, and discussion of the
Mie scattering range can be found in
Martin 1978,
Draine & Lee 1984,
Wright 1987,
and references therein). Observations of galactic dust
indicate that outside resonances f( )
) 
 1-
1- , with 1
, with 1


 2
(Whittet 1988).
2
(Whittet 1988).
 )
)  1, and reradiated
at wavelengths
1, and reradiated
at wavelengths  >
2
>
2  a. Hence if we ignore
heating and cooling of grains by collisions
with atoms (generally weak), the temperature of directly illuminated
grains is given approximately by
a. Hence if we ignore
heating and cooling of grains by collisions
with atoms (generally weak), the temperature of directly illuminated
grains is given approximately by
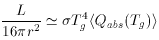
 Tg
Tg . Crudely, therefore, Tg
. Crudely, therefore, Tg  [L / (r2 a)]1/(4+
[L / (r2 a)]1/(4+ ). At a given distance from
the source, the smallest
grains in thermal equilibrium (~ 30 Å) will be about a factor of 2
hotter than the largest grains commonly considered (~ 0.3 µm).
). At a given distance from
the source, the smallest
grains in thermal equilibrium (~ 30 Å) will be about a factor of 2
hotter than the largest grains commonly considered (~ 0.3 µm).
 20 Å is so low that their
temperature fluctuates, being significantly affected by the absorption
of a single UV photon. Grains deeper in the dusty disk will not be
exposed directly to UV radiation from the central source, but to
longer-wavelength re-emission from shielding gas and dust. This
shielded dust will have a temperature nearly independent of a, and
slightly lower (by a factor ~ (Tre / 2500
K)1/(4+
20 Å is so low that their
temperature fluctuates, being significantly affected by the absorption
of a single UV photon. Grains deeper in the dusty disk will not be
exposed directly to UV radiation from the central source, but to
longer-wavelength re-emission from shielding gas and dust. This
shielded dust will have a temperature nearly independent of a, and
slightly lower (by a factor ~ (Tre / 2500
K)1/(4+ )) than that of the
directly illuminated grains of temperature Tre.
)) than that of the
directly illuminated grains of temperature Tre.



 2+
2+ Tg, peaks
very sharply at h
Tg, peaks
very sharply at h ~ (3 +
~ (3 +
 )kTg
(
)kTg
( ~ 30
T2-1 µm, where
Tg = 100 T2 K) and declines
exponentially at higher
frequencies. Except for the small grains of fluctuating temperature,
which can contribute high frequency emission from regions where the
equilibrium temperature is low, most emission at frequency v will come
from the radius where the equilibrium dust temperature
Tg ~ h
~ 30
T2-1 µm, where
Tg = 100 T2 K) and declines
exponentially at higher
frequencies. Except for the small grains of fluctuating temperature,
which can contribute high frequency emission from regions where the
equilibrium temperature is low, most emission at frequency v will come
from the radius where the equilibrium dust temperature
Tg ~ h / (3 +
/ (3 +
 ) k, i.e., from a radius
r
) k, i.e., from a radius
r  L1/2
L1/2  -(4+
-(4+ )/2, and the flux of radiation at
that frequency will (for an isotropic central source) be proportional
to the fraction of the sky at the central source covered by dust at
the appropriate radii. This radius-frequency scaling can cause curious
effects. At frequencies where
)/2, and the flux of radiation at
that frequency will (for an isotropic central source) be proportional
to the fraction of the sky at the central source covered by dust at
the appropriate radii. This radius-frequency scaling can cause curious
effects. At frequencies where  is
large (e.g., 1 <
is
large (e.g., 1 <  < 7
µm, where
< 7
µm, where 
 1.7 -
Whittet 1988),
the temperature is nearly independent of radius,
enhancing the probability of having a large warp at an appropriate
radius, and hence a large sky covering factor and a large ~ 3
µm flux
(see figure 2). This may be the
cause of the ``3-5 µm bumps'' commonly observed in AGN
(Edelson & Malkan
1986).
1.7 -
Whittet 1988),
the temperature is nearly independent of radius,
enhancing the probability of having a large warp at an appropriate
radius, and hence a large sky covering factor and a large ~ 3
µm flux
(see figure 2). This may be the
cause of the ``3-5 µm bumps'' commonly observed in AGN
(Edelson & Malkan
1986).