4.3. Multiple Encounters?

It is likely that most ring-making intruder galaxies are bound or almost bound to the target disk. Moreover, they will be more tightly bound after the collision as a result of dynamical friction. The amount of friction will depend on the mass and structure of the spheroidal components and halos of the two galaxies. Merger simulations of simple spheroidal galaxies indicate that friction can be very effective in many cases. In these cases the first collision will be followed by a second, and typically a prompt merger after that.

When the intruder galaxy initially strikes the target disk off-center on the first pass, the second collision is likely to be even more asymmetric. Nonetheless, it is useful to study the special case of two successive symmetric collisions as an introduction to the phenomena that can be produced in multiple interactions. This situation has been discussed in a manuscript of Lotan and Struck-Marcell (1991), and Lotan-Luban (1990), where models and numerical simulations were presented.

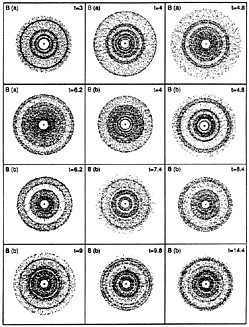
Equation (4.5) is based on the assumption that the disk stars were in simple circular orbits before the collision. This is clearly not the case at the time of the second collision, since the stars are executing radial epicyclic oscillations as a result of the first collision. The magnitude (and direction) of the radial velocities of the disk stars at the time of the second impact will be a smooth function of radius in the disk. That is, some stars will be moving inward, others outward, others will be near apoapse or periapse and will not have a significant radial velocity. If we assume that the second collision is also impulsive, the velocity impulse will be added to the star's current velocity. If the star already had a significant velocity inward, this will result in a considerable increase in that velocity. If the star was moving outward, the second collision may result in a net radial velocity of nearly zero. That is, in some range of radii within the disk the two perturbations will nearly cancel, while in others they will add, with intermediate values in between. The results can be dramatic, with very wide caustic rings in the large amplitude regions, and thin rings or only modest orbit crowding zones in the low amplitude regions. Moreover, simulations show that thick and thin rings tend to alternate (see Figure 18, from Lotan and Struck-Marcell 1991). Further details are given in Appendix 1.

Figure 18. Pole-on view of a test particle disk from the multiple impact simulations of Lotan-Luban (1990, also Lotan and Struck-Marcell 1991). Upper case letter identifies the model, and it is followed by either an "a" for the (control) case of a single collision, or a "b" for the multiple collision case. Dimensionless time is also given (in units of the initial disk radius divided by the rotational velocity at that radius).