4.2. Luminosity Functions

In this section, we compare the detected EBL with the EBL predicted by luminosity functions measured as a function of redshift. To avoid unnecessary complications in defining apparent magnitude cut-offs, and to facilitate comparison with other models of the luminosity density as a function of redshift, we compare luminosity functions with the total EBL rather than with EBL23, as in the previous section. To do so, we combine the EBL23 flux measured in Paper I with the flux from number counts at brighter magnitudes, as given in Table 2. Systematic errors in photometry of the sort discussed in Section 4.1 are likely to be relatively small for redshift surveys because the objects selected for spectroscopic surveys are much brighter than the limits of the photometric catalogs (although see Dalcanton 1998 for discussion of the effects of small, systematic photometry errors on inferred luminosity functions). We have not tried to compensate for such effects here.

The integrated flux from galaxies at all redshifts is given by

(1)

in which V_c(z) is the comoving volume element, D_L(z) is the luminosity distance, ₀ is the observed wavelength, and _z = ₀(1 + z)^-1 is the rest-frame wavelength at the redshift of emission. To compare the detected EBL to the observed luminosity density with redshift, (, z), we begin by constructing the SED of the local luminosity density as a linear combination of SEDs for E/S0, Sb, and Ir galaxies, weighted by their fractional contribution to the local B-band luminosity density:

(2)

in which the subscript i denotes the galaxy Hubble type (E/S0, Sb, or Ir), f_i() denotes the galaxy SED (the flux per unit rest-frame wavelength), and _i(B, 0) is the B-band, local luminosity density in ergs s^-1 Å^-1 Mpc^-3. To produce the integrated spectrum of the local galaxy population, we use Hubble-type-dependent luminosity functions from Marzke et al. (1998) and SEDs for E, Sab, and Sc galaxies from Poggianti (1997). We adopt a local luminosity density of _B = 1.3 × 10⁸ hL Mpc^-3, consistent with the Loveday et al. (1992) value adopted by CFRS and also with Marzke et al. (1998). ⁽¹⁾ The spectrum we obtain for (, 0) is shown in Figure 7.

Figure 7. The local luminosity density constructed by combining the spectral energy distributions of E/S0, Sab, and Sc galaxies weighted according to the type-dependent luminosity functions as described in Section 4.2 and Equation 2.

We note that the recent measurement of the local luminosity function by Blanton et al. (2001) indicates a factor of two higher local luminosity density than found by previous authors. Previous results are generally consistent with Loveday et al. to within 40%. Blanton et al. attribute this increase to deeper photometry which recovers more flux from the low surface brightness wings of galaxies in their sample relative to previous surveys (see discussions in Section 4.1), and photometry which is unbiased as a function of redshift. For the no-evolution and passive evolution models discussed below, the implications of the Blanton et al. results can be estimated by simply scaling the resulting EBL by the increase in the local luminosity density. Although the Blanton et al. results do not directly pertain to the luminosity functions measured by CFRS at redshifts z > 0.2, they do suggest that redshift surveys at high redshifts will underestimate the luminosity density, as discussed by Dalcanton (1998).

In the upper panel of Figure 8, we compare the EBL flux we detect with EBL flux predicted by five different models for (, z), using the local luminosity density derived in Equation 2 as a starting point. For illustrative purposes, the first model we plot shows the EBL which results if we assume no evolution in the luminosity density with redshift, i.e. (, z) = (, 0). The number counts themselves rule out a non-evolving luminosity density, as has been discussed in the literature for over a decade; inconsistency between the detected EBL and the no-evolution model is just as pronounced. The predicted EBL for the no-evolution model is a factor of 10 fainter than the detected values (filled circles). These are 1.7, 2.1, and 2.2 discrepancies at U₃₀₀, V₅₅₅ and I₈₁₄, respectively. More concretely, the no-evolution prediction is at least a factor of 12 × , 4 × , and 3.7 × lower than the flux in individually resolved sources at U₃₀₀, V₅₅₅ and I₈₁₄ (lower-limit arrows). Note that the no-evolution model still underpredicts the EBL if we rescale the local luminosity density to the Blanton et al. (2001) values. This model demonstrates the well-known fact that luminosity density is larger at higher redshifts.

Figure 8. The upper panel shows the spectrum of the EBL calculated by integrating the luminosity density over redshift (Equation 1) for constant luminosity density, passively evolving luminosity density, and evolution of the form (, z) = (, 0)(1 + z)(), with (, z) constant at z > 1. The lower panel shows () for the three cases of (1 + z)() as labeled in the figure and described in the text. Line types and hatch-marked regions in upper panel correspond to values of () with the same line type in the lower panel. Filled circles show the mean EBL detections with 2 error bars. The error bars are dashed where they extend below the cumulative flux in detected sources - the minimum values for the EBL - indicated by the lower limit brackets.

The second model we plot in Figure 8 shows the effect of passive evolution on the color of the predicted EBL. In this model, we have used the Poggianti (1997) SEDs for galaxies as a function of age for H₀ = 50 km s^-1 Mpc^-1 and q₀ = 0.225. In the Poggianti models, stellar populations are 2.2 Gyrs old at a z ~ 3. The resulting (, z) is bluer than the no-evolution model due to a combination of K-corrections and increased UV flux for younger stellar populations. The passive evolution model does provide a better qualitatively match to the SED of the resolved sources (lower limits) and EBL detections (filled circles); however, it is still a factor of 3 × less than the flux at U₃₀₀, and a factor of 2 × less than the flux we recover from resolved sources at V₅₅₅ and I₈₁₄. For the adopted local luminosity density and Poggianti models, passive evolution is therefore not sufficient to produce the detected EBL. Again, the passive evolution adopted here still underpredicts the EBL if we rescale the local luminosity density by a factor of two to agree with the Blanton et al. (2001) value.

As a fiducial model of evolving luminosity density, we adopt the form of evolution implied by the CFRS redshift survey (Lilly et al. 1996, hereafter CFRS) and Lyman-limit surveys of Steidel et al. (1999): (, z) = (, 0)(1 + z)⁽⁾ over the range 0 < z < 1 and roughly constant luminosity density at 1 < z < 4. The remaining three models shown in Figure 8 test the strength of evolution of that form which is allowed by the EBL detections. The hatch-marked region shows the EBL predicted for values of () which represent the ± 1 range found by CFRS for the redshift range 0 < z < 1. The value of the exponent () is indicated in the lower panel of Figure 8, and the hatch-marked region reflects the uncertainty in the high redshift luminosity density due to the poorly constrained faint-end slope of the luminosity functions. This ± 1 range of the predicted EBL is consistent with the detected EBL at U₃₀₀, but is inconsistent with the EBL detections at V₅₅₅ and I₈₁₄ at the 1 level of both model and detections. It is, however, consistent with the integrated flux in detected sources at V₅₅₅ and I₈₁₄.

To test the range of evolution allowed by the full ± 2 range of the EBL detections, we can explore two possibilities: (1) stronger evolution at 0 < z < 1, shown in Figure 8; and (2) evolution continuing beyond z = 1, shown in Figures 9, 10, and 11. Addressing the possibility of constant luminosity density at z > 1, the dashed line in the upper panel of Figure 8 shows the EBL predicted by the 2 upper limit for () from CFRS; the dot-dashed line corresponds to the value of () required to obtain the upper limits of the EBL detections at all wavelengths. Note that the latter implies a value for (4400Å, 1) which is ~ 10 × higher than the value estimated by CFRS. This result emphasizes that the 2 interval of the EBL detections span a factor of 4 in flux at 4400Å, and thus the allowed range in the luminosity density for < 4400Å and 0 < z < 1 is similarly large. Also, for each model in which the luminosity density is constant at z > 1, less than 50% of the EBL will come from beyond z = 1 due to the combined effects of K-corrections and the decreasing volume element with increasing redshift (see Figure 10).

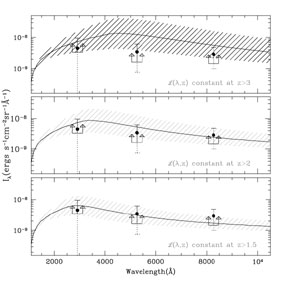
Figure 9. The three panels show the spectrum of the EBL calculated assuming (, z) = (, 0)(1 + z)() over the range 0 < z < 1.5 (bottom panel), 0 < z < 2 (middle), and 0 < z < 3 (top). In all cases, the luminosity density is held constant beyond the indicated redshift limit. The hatch-marked regions each show the ± 1 range of CFRS values for (), as in Figure 8. The integrated EBL as a function of redshift is shown in Figure 10. Luminosity density as a function of redshift is shown in Figure 11 for some combinations of () and the redshift cut-off for evolution. The filled circles, error bars and lower limit symbols are as in Figure 8.

Figure 10. The integrated EBL at V555 contributed as a function of increasing redshift from z = 0 to z = 10. As marked in the figure, the lines show the integrated flux for no evolution in the luminosity density, passive evolution, and evolution of the form (, z) (1 + z)() for the -1, mean, and +1 range of () values determined by CFRS. For each (), we plot the growth of the EBL with redshift if (, z) is held constant at z > 1, z > 1.5, z > 2, and z > 3, corresponding to Figures 8 and 9.

Evolution continuing beyond z = 1 is possible if the Lyman-limit-selected surveys have not identified all of the star formation at high redshifts, and estimates of the luminosity density at 3 z 4 are subsequently low. Figures 9 and 10 show the EBL predicted by models in which the luminosity density increases as (1 + z)⁽⁾ to redshifts of z = 1.5, 2 and 3. Clearly, significant flux can come from z > 1 if the luminosity density continues to increase as a power law. The rest-frame U₃₀₀ luminosity density is plotted as a function of redshift in Figure 11 for limiting values of the cut-off redshift for evolution and (). Although the strongest evolution plotted over-predicts the detected EBL, our detections are clearly consistent with some of the intermediate values of the () and increasing luminosity density beyond z = 1. For example, the mean rate of increase in the luminosity density found by CFRS can continue to redshifts of roughly 2.5-3 without over-predicting the EBL.

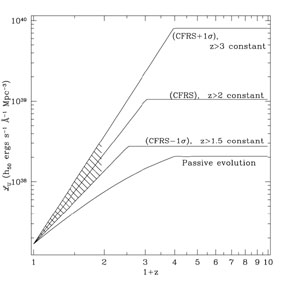
Figure 11. The luminosity density at U300 as a function of redshift corresponding to limiting cases plotted in Figure 9. The hatch-marked region indicates the ± 1 range given by the CFRS measurements of () over the range 0 < z < 1. The horizontal line segments show the luminosity density corresponding to the -1 limit for () held constant at z > 1.5; the mean value for () held constant at z > 2; and the +1 limit for () held constant at z > 3.

In all models, we have adopted the same cosmology (h = 0.5 and = 1.0) as assumed by CFRS and Steidel et al. (1999) in calculating (, z) and (). Although the luminosity density inferred from these redshift surveys depends on the adopted cosmological model, the flux per redshift interval is a directly observed quantity. The EBL is therefore a directly observed quantity over the redshift range of the surveys, and is also model-independent. To the degree that the luminosity density becomes unconstrained by observations at higher redshifts, the EBL does depend on the assumed (not measured) luminosity density and on the adopted cosmology through the volume integral. Although dependence of the predicted EBL on H₀ cancels out between the luminosity density, volume element, and distance in Equation 1, H₀ has some impact through cosmology-dependent time scales, which affect the evolution of stellar populations. If the luminosity density is assumed to be constant for z > 1, the predicted EBL increases by 25% at V₅₅₅ for (_M = 0.2, = 0) and corresponding values of (), and decreases by 50% for (0.2,0.8). The luminosity densities corresponding to the 2 upper limit of the detected EBL change by the same fractions for the different cosmologies if is constant at z > 1. Similarly, for models in which the luminosity density continues to grow at z > 1, the luminosity density required to produce the EBL will be smaller if we adopt (0.2,0) than (1,0), and smaller still for (0.2,0.8). The exact ratios depend on rate of increase in the luminosity density.

Several authors (Treyer et al. 1998, Cowie et al. 1999, and Sullivan et al. 2000) have found that the at UV wavelengths (2000-2500Å) is higher than claimed by CFRS (2800Å) in the range 0 < z < 0.5 and have found weaker evolution in the UV luminosity density, corresponding to (2000Å) ~ 1.7. The implications for the predicted EBL can be estimated from the plots of the (U₃₀₀, z) shown in Figure 11, and the corresponding EBL in Figures 9 and 10. For instance, if the local UV luminosity density is a factor of 5 higher than the value we have adopted and if (2000Å) ~ 1.7 over the range 0 < z < 1, then the rest-frame UV luminosity density at z = 1 is similar to that measured by CFRS, and the predicted U₃₀₀ EBL will be roughly 3.5 × 10^-9 cgs, very similar to the EBL we derive from our modeled local luminosity density and the mean values for () from CFRS.

¹ For a B-band solar irradiance of L = 4.8 × 10²⁹ergs s^-1 Å^-1, (B, 0) = 6.1 × 10³⁷h ergs s^-1 Mpc ^-3 = 4.0 × 10¹⁹h₅₀ W Hz^-1 Mpc^-3. Back.