2.3. Some reasons for caution

Despite the above impressive set of agreements cited above, one should keep an open mind. The question of the age of the Universe is not an issue: models fitting adequately the C_l curve leads to similar ages, consistent with existing constraint. For instance, the model drawn on figure 2 (left side) has an age of 15 Gyr, well consistent with age estimates (actually a model with t₀ ~ 10 Gyr should probably not be securely rejected on this basis). Identically the amplitude of matter fluctuations on small scales is sometimes claimed to be inconsistent with a high density universe, while there is actually a degeneracy between this amplitude and the matter density parameter _m. Very often, authors implicitly refer to the standard CDM scenario (_m = 1, h ~ 0.5, n = 1). Actually this simplest CDM model is known to be ruled out from several different arguments, but there exists also different way one can imagine the spectrum to be modified in order to match the data (an example is a possible contribution of hot dark matter of the order of 20%).

A high density universe is actually inconsistent (because of the age problem) with value of the Hubble constant as high as those found by the HST. However, the HST measurement of the Hubble constant has been questioned (Arp, 2002). In order to illustrate the argument I show the figure given by Arp, which is claimed to represent the Hubble diagram from the HST data. Clearly, a firm conclusion on the Hubble constant from this data seems difficult and actually Arp claims that data can favor H₀ ~ 55 km/s/Mpc. An other doubt on the Hubble constant comes from the Sunyaev-Zeldovich measurements: in a recent review Carlstrom et al. (2002) found that the best value slightly depends on the cosmology, but that in an Einstein-de Sitter model one finds an average values of H₀ ~ 55 km/s/Mpc, furthermore given that such determination suffers from possible clumping of the gas (Mathiesen et al., 1999; see below), the actual value could be 25% less!

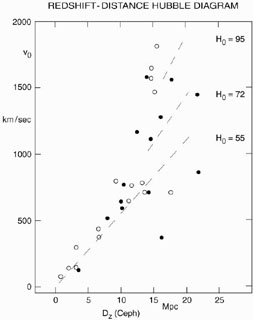Figure 3. Hubble diagram from HST cepheids according to Arp (2002). Clearly the derivation of a value from this data set is uncertain. But a value of 55 km/s/Mpc seems as least as adequate as the HST finding (72 km/s/Mpc).

Let us now examine observational direct evidences for or against a non-zero cosmological constant. Distant SNIa are observed to be fainter than expected (in a non-accelerating universe) given their redshift, indicating very directly that the universe is accelerating should they be standard candles. The signal is of the order of 0.3 magnitude (compared to an Einstein-de Sitter universe). It is important to realize that several astrophysical effects of the same order are already existing, and that their actual amplitude might be difficult to properly evaluated. Rowan-Robinson (2002) argued for instance that the dust correction might have been underestimated in high redshift SNIa, while such a correction is of the same order of the signal. Identically, the K-correction that has to be applied to high redshift supernovae is large (in the range 0.5-1. mag) and is estimated from zero redshift spectral templates; one can therefore worry whether some shift in the zero-point would not remain from the actual spectrum, with an amplitude larger than the assumed uncertainty (2%). Identically, the progenitor population at redshift 0.5 is likely to be physically different from the progenitors of local SNIa (age, mass, metalicity). Consequence on luminosity are largely unknown.

Finally it is worth noticing that the first 7 distant SNIa which were analyzed conducted to conclude to the rejection of a value of as large as 0.7: < 0.51 (95%) (Perlmutter et al., 1997). Several arguments have been used in the past or recently to set upper limit on a dominant contribution of (Maoz et al., 1993; Kochanek, 1996; Boughn et al., 2002). There is therefore a number of arguments for caution:

1) SNIa measurements provide the single direct evidence for a cosmological constant,
2) most measurements of _m are local in nature (mostly inferred from clusters),
3) some upper limits have been published on which do not agrre with recent measurements ,
4) a non-zero cosmological constant is an extraordinary new result in physics and therefore deserves extraordinary piece of evidence.

Before the existence of the cosmological constant can be considered as scientifically established, it is probably necessary to reinforce evidence for the convergence model by obtaining further direct evidence for a cosmological constant. Because there exist degeneracies in parameters determination with the CMB, even the Planck experiment will not allow to break these degeneracies. It is therefore necessary to use tests which provide complementary information. The data provided by the distant SNIa satisfies well this requirement. As it is difficult to think of a new test measuring directly the presence of a cosmological constant, the best approach is probably still to try to have reliable estimates of the matter density from global technics. In this respect, clusters are probably the most powerful tool, as they provide several major roads to measure the density matter of the Universe and which exploration is still in its infancy. Here I will concentrate on this perspective.