Annu. Rev. Astron. Astrophys. 2000. 38: 289-335 Copyright © 2000 by Annual Reviews. All rights reserved

5.2. Mass Estimates

One of the most important applications of X-ray observations of groups has been mass estimates. Prior to ROSAT, mass determinations for groups were largely based on application of the virial theorem to the group galaxies. For a typical cataloged group with only four or five velocity measurements, the virial method can be unreliable (e.g. Barnes 1985, Diaferio et al 1993).

The method used to estimate group masses from X-ray data is analogous to the technique developed for rich clusters (e.g. Fabricant et al 1980, 1984; Fabricant & Gorenstein 1983; Cowie et al 1987). The fundamental assumption is that the hot gas is trapped in the potential well of the group and is in rough hydrostatic equilibrium. This assumption is probably a reasonable one for most groups, given the short sound-crossing times in these systems. A further assumption is that the only source of heating for the gas is gravitational, i.e. that the gas temperature is a direct measure of the potential depth and therefore of the total mass. This assumption may not be strictly true for some groups. In particular, the fact that the heavy metal abundance of the intragroup medium is non-zero suggests that some of the gas has been reprocessed in the stars in galaxies and ejected by supernovae-driven winds. In addition to polluting the intragroup gas with metals, such winds also provide additional energy to the gas. It has generally been assumed in the literature that the energy contribution of such winds is negligible. Semi-analytic models suggest that this assumption is fair as long as the temperature of the system is greater than about 0.8 keV (Balogh et al 1999, Cavaliere et al 1999). Thus, for many groups, the hydrostatic mass estimator should be valid.

With the further assumption of spherical symmetry, the mass interior to radius R is given by (Fabricant et al 1984):

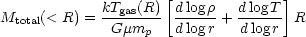where k is Boltzmann's constant, T_gas(R) is the gas temperature at radius R, G is the gravitational constant, µ is the mean molecular weight, m_p is the mass of the proton, and is the gas density. In principle, all of the unknowns in this equation can be calculated from the X-ray data. Typically, the gas temperature is measured directly from the X-ray spectrum and the gas density profile is determined by fitting the standard beta model to the surface brightness profile. Unfortunately, it is often necessary to make a further assumption that the gas is isothermal (i.e. d log T / d log r = 0). For a few groups, the temperature profile can be directly measured. The resulting mass estimates suggest that the isothermal assumption generally results in an error in the mass of no more than about 10% (e.g. David et al 1994, Davis et al 1996). With the isothermal assumption, M_total (< R) T_gas R (as long as R is much larger than the core radius in the beta model. Therefore, if is underestimated from the surface brightness profile fits by a factor of ~ 2 (see Sections 3.3.3 and 3.4.3), then the mass estimates are also too small by a factor of ~ 2.)

ROSAT measurements indicated a small range of total group masses with nearly all of the systems clustered around 10¹³ h^-1 M (see Figure 8; Mulchaey et al 1993, Ponman & Bertram 1993, David et al 1994, Pildis et al 1995, Henry et al 1995, Mulchaey et al 1996a). The narrow range of group masses is not too surprising, given that nearly all the groups in these surveys have temperatures of ~ 1 keV.

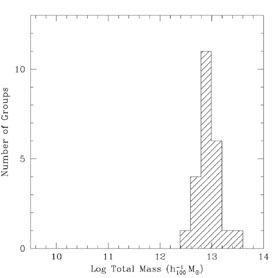Figure 8. Distribution of X-ray-determined total group masses. In each case, the masses are determined out to the radius to which the X-ray emission is detected. The sample is based on the compilation given in Mulchaey et al 1996a, with the addition of a few groups with more recent X-ray mass estimates in the literature.

The X-ray mass estimates can generally be applied only to a radius of several hundred kiloparsecs. Beyond that, the gas density profile is not well-constrained. Because the virial radius for a 1 keV group is approximately ~ 0.5 h^-1₁₀₀ Mpc, the X-ray method measures only a fraction of the total mass (Ponman & Bertram 1993; David et al 1995; Henry et al 1995). Simply extrapolating out to the virial radius, the total group masses are a factor of approximately two to three times larger than those implied from the X-ray studies (Mass R). However, if non-gravitational heat is important in groups, the extrapolation out to the virial radius is more uncertain (Loewenstein 2000).

Because of their relatively large masses, X-ray groups make a substantial contribution to the mass density of the universe (Mulchaey et al 1993, Henry et al 1995, Mulchaey et al 1996a). Based on their X-ray-selected group sample, Henry et al (1995) estimate that X-ray luminous groups contribute ~ 0.05. However, their sample contained only the most luminous, elliptical-rich groups. When one corrects for the groups missing from Henry et al's (1995) sample (assuming a similar mass density), groups might contribute as much as ~ 0.25. These estimates are comparable to the numbers found for richer clusters, which verifies the cosmological significance of poor groups.