

The structure and general properties of stellar disks have been reviewed in some detail by Ken and myself at previous honorary symposia (e.g. van der Kruit 2002, Freeman 2007). The radial distribution of surface brightness can be approximated by an exponential (Freeman 1970) and the vertical distribution with an isothermal sheet (van der Kruit & Searle 1981a) with a scaleheight that is independent of galactocentric distance. A more general form is (van der Kruit 1988)
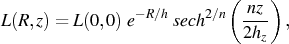 |
(1) |
This ranges from the isothermal distribution (n = 1) to the
exponential function (n =
 ) and allows for
more realistic
stellar distribution that are not exactly isothermal in z. From
actual fits in I and K'
de Grijs et
al. (1997)
found
) and allows for
more realistic
stellar distribution that are not exactly isothermal in z. From
actual fits in I and K'
de Grijs et
al. (1997)
found
 |
(2) |
A detailed study by de Grijs & Peletier (1997) has shown that the constancy of the vertical scaleparameter hz is very accurate in late-type spiral disks, but in early-types it may increase, in an extreme case by as much as 50% per scalelength h.
The origin of the exponential nature of stellar disks is still uncertain. Freeman (1970, 1975) already pointed out that the distribution of angular momentum in a self-gravitating exponential disk resembles that of the uniform, uniformly rotating sphere (Mestel 1963). This also holds for an exponential density distribution with a flat rotation curve (Gunn 1982, van der Kruit 1987), so that a model with collapse with detailed conservation of angular momentum (see also Fall & Efstathiou 1980) would give a natural explanation for the exponential nature of disks (and maybe their truncations; see below). However, bars or other non-axisymmetric structures may induce severe redistribution of angular momentum; the work on the effects of nonaxisymmetric instabilities on the secular evolution of disks and their structural parameters by (Debattista et al. 2007) shows the potential of such approaches.
The distribution of the scale parameters is most easily studied in edge-on galaxies. Following on from the studies by van der Kruit & Searle (1981a, 1981b, 1982a), an extensive sample of edge-on galaxies has been studied by de Grijs (1998), and has been re-analysed by Kregel et al. (2002). The scale parameters correlate well with the rotation velocity of the galaxy, e.g. for the scaleheight
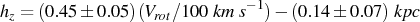 |
(3) |
with a scatter of 0.21 kpc. This could be useful to find a statistical estimate of the thickness of disks in galaxies that are not seen edge-on. The flattest galaxies (largest ratio of h and hz) appear to be those with late Hubble type, small rotation velocity and faint (face-on) surface brightness.